A pipe is running full of water. At a certain point A it tapers from 60cm diameter to 2.0cm diameter at B. The pressure difference between A and B is 100cm of water column. Find the rate of flow of water through the pipe.
Diameter of tube at A=60cm
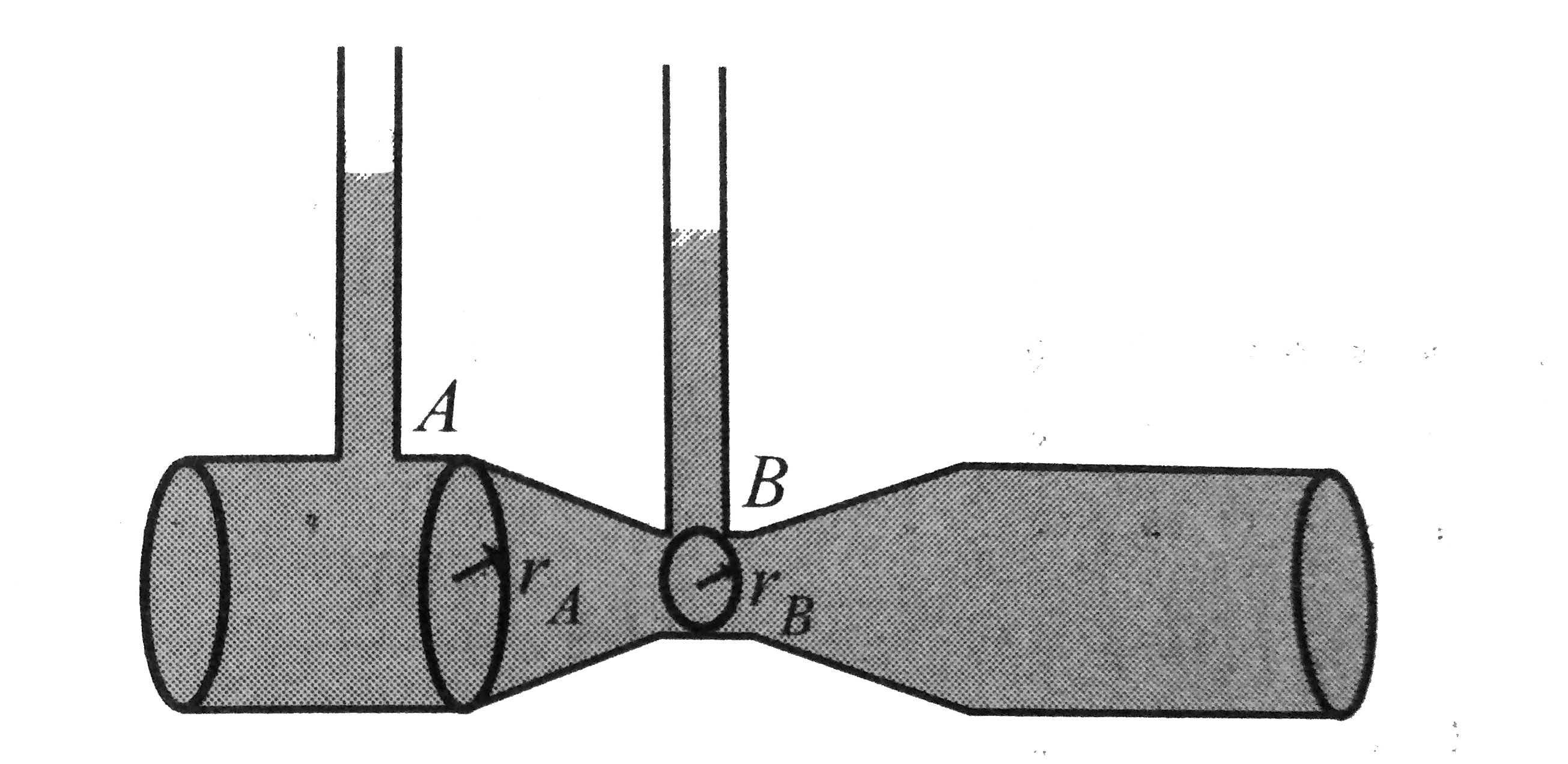
Radius at A=rA=30cm, radius at B=rB=10cm
area of cross section at A=π(30)2cm2=A1
area of cross section at B=π(10)2=A2
PA−PB=100cm of water of 100×1×980dyn/cm2
Using the formula Q=A1A2
⎷2(PA−PB)(A21−A22)d
Substitute the value
Q=(900π)(100π)
⎷2×(100×1×980)(9002π2−1002π2)0
⇒Q=1.4×105cm3/s=1401/s
Topper's Solved these Questions
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - Subjective
25 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - Single Correct
95 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - Exercise 4.3
10 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
A pitot tube is fixed in a main pipe of diameter 20 cm and difference of pressure indicated by the gauge is 5 cm of water column. Find the volumn of water passing through the main pipe in one minute.
View SolutionA pitot tube is fixed in a water pipe of diameter 14cm and difference of pressure by the gauge is 20cm of water column. Calculate the rate of flow of water.
View Solution
Knowledge Check
Water contained in a tank flows through an orifice of a diameter 2 cm under a constant pressure difference of 10 cm of water column. The rate of flow of water through the orifice is
A44cc/sB4.4cc/sC444cc/sD4400cc/sTwo water pipes of diameters 2 cm and 4 cm are connected with the main supply line. The velocity of flow of water in the pipe of 2 cm
A4 times that in the other pipeB14 times that in the other pipeC2 times that in the other pipeD12 times that in the other pipeA horizontal pipe of cross-section diameter 5cm carries water at a speed of 4ms−1. The pipe is connected to a smaller pipe with a cross-sectional diameter 4cm. The velocity of water through the smaller pipe is
A6.25ms−1B5.0ms−1C3.2ms−1D2.56ms−1
The diameter of the throat of a venturimeter is 6 cm .when it is inserted in a horizontal pipe line of diameter 10 cm the pressure difference between the pipe and the thraot equal 8 cm of water . Calulate the rate of flow.
View SolutionA pitot tube is mounted in a main pipe of diameter 40 cm. If the difference in pressure indicated by the gauge ie 6cm of water column, calculate the volume of air passing through the main pipe in two minutes.
View SolutionWater running in a cylindrical pipe of inner diameter 7 cm, is collected in a container at the rate of 192.5 litres per minute. Find the rate of flow of water in the pipe in km/hr.
View SolutionA venturimeter is connected to two points in the mains where its radii are 20cm. And 10cm. Respectively and the levels of water column in the tube differ by 10cms. How much water flows through the pipe per minute?
View SolutionThe dimeter of a pipe at two point, where a venturimeter is connection is 9 cm 4 cm and the difference of level in it is 4 cm. Calculate the volumw of water flowing through the pipe per second.
View Solution
