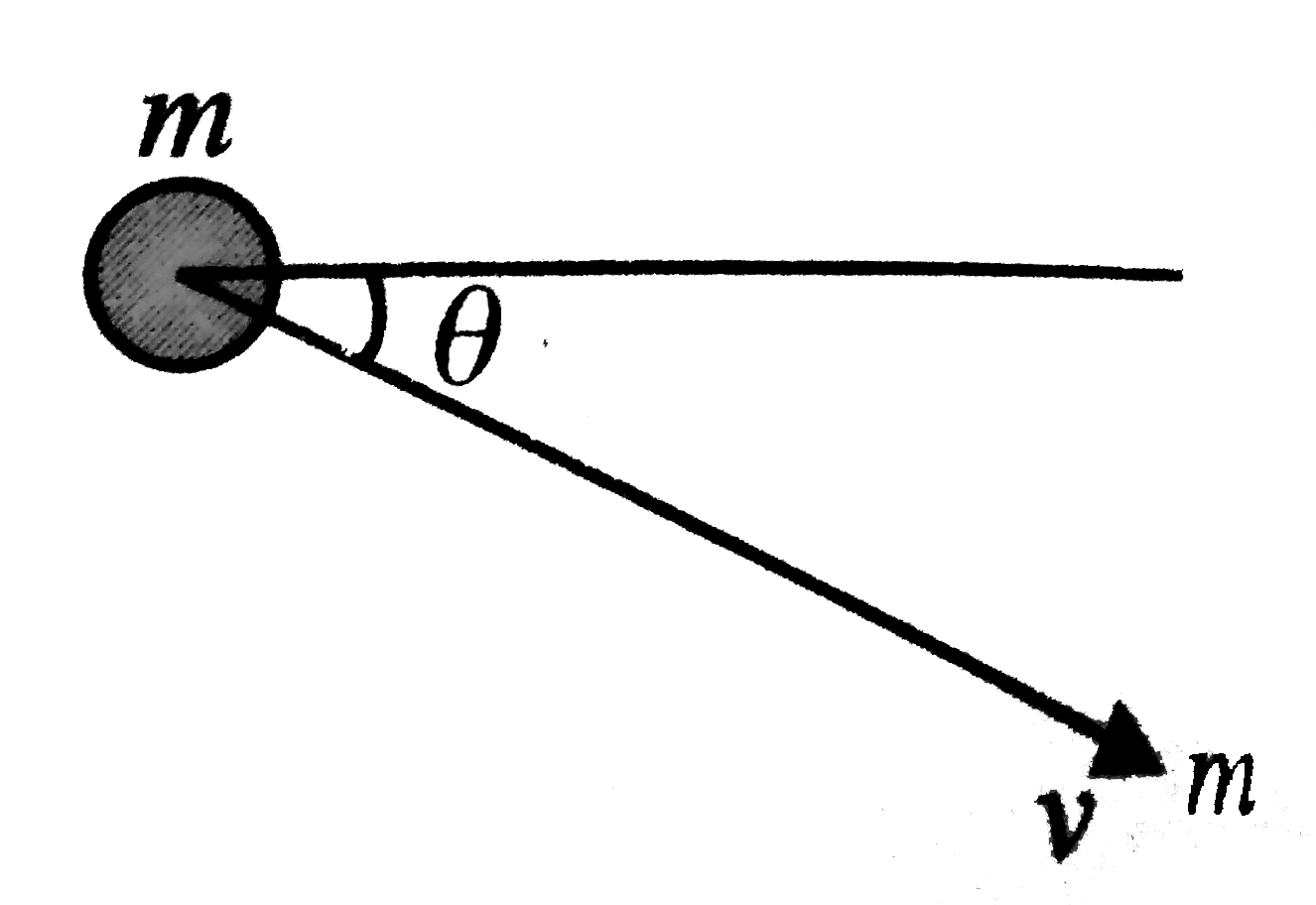
A particle at rest is constrained to move on a smooth horizontal surface. Another identical particle hits the fractional particle with a velocity v at an angle θ=60∘ with horizontal. If the particles move together, the velocity of the combination just after impact is equal to
v
v2
√3v4
v4
The correct Answer is:D
The impact takes place along the line joining them. Since the particle at rest is constrained to move on the horizontal surface, we cannot conserve the momentum along the line joining them. Since no net force is acting on the system of particle parallel to the surface, conservation of linear momentum along the direction yields the following result
mvcosθ=(m+m)v'⇒v'=v2cos60∘=v4
Topper's Solved these Questions
- CENTRE OF MASSBOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Multiple Correct
25 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Assertion - Reasoning
2 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Subjective
23 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICSCHAPTER - CALORIMETRYEXERCISE - Solved Example
13 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
Similar Questions
A charge "Q" of mass "m" is at rest on a smooth horizontal plane.Another identical particle is projected with a velocity v towards it from long distance then
View SolutionA particle is projected with velocity u at angle θ with horizontal. Find the time when velocity vector is perpendicular to initial velocity vector.
View Solution
Knowledge Check
A particle at rest is constrained to move on a smooth horizontal surface. Another identical particle hits the stationary particle with a velocity v at an angle θ=60∘ with horizontal. If the particles move together, the velocity of the combination just after the impact is equal to
AvBv/2C√3v/4Dv/4A particle is projected with velocity v at an angle θ aith horizontal. The average angle velocity of the particle from the point of projection to impact equals
AgcosθθvBgvsinθCgvθDgθvsinθA particle is projected with velocity v at an angle of θ with horizontal. The average angular velocity of the particle from the point of projection to impact equals:
AgcosθθvBgvsinθCgvθDgθvsinθ
A particle of mass m is projected with a velocity μ at an angle of θ with horizontal.The angular momentum of the particle about the highest point of its trajectory is equal to :
View SolutionA particle is projected from a point P with a velocity v at an angle θ with horizontal. At a certain point Q it moves at right angles to its initial direction. Then
View SolutionA particle projected with velocity u at angle θ with horizontal at t=0. What is the magnitude of change in the velocity of the particle when it is at maximum height?
View SolutionTwo particle are projected with same initial velocities at an angle 30∘ and 60∘ with the horizontal .Then
View SolutionA particle is projected in air with an initial velocity u directed at an angle θ above horizontal. After time t0 the velocity of the particle is directed at angle ϕ above horizontal.
View Solution
