The amplitude and maximum velocity will be respectively X=3sin2t+4cos2tThe amplitude and maximum velocity will be respectively
A
5, 10
B
3, 2
C
4, 2
D
3, 4
Video Solution
Text Solution
The correct Answer is:A
x=3sin2t+4cos2t.
From given equation, a1=3,a2=4andx=3sin2t+4cos2t
√32+42[3√32+42.sin2t+4√32+42cos2t]
5[53sin2t+45cos2t]
=5[sin2tcosα+sinαcos2t]
∴ cosα=35andsinα=45

∴ x=5.[sin2t.cosα+sinα.cos2t]
=5sin(2t+α)
∴ ω=2andα=5
∵ [sin(A+B)=sinAcosB+cosAsinB]
⇒ Vmax=aω=5×2=10
|
Topper's Solved these Questions
- OSCILLATIONSBook:MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSChapter:OSCILLATIONSExercise:EXERCISE 2
Explore 49 Videos
- OSCILLATIONSBook:MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSChapter:OSCILLATIONSExercise:MHT CET CORNER
Explore 29 Videos
- OSCILLATIONSBook:MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSChapter:OSCILLATIONSExercise:MHT CET CORNER
Explore 29 Videos
- MOCK TEST 5Book:MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSChapter:MOCK TEST 5Exercise:MCQs
Explore 42 Videos
- PRACTICE SET 01Book:MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSChapter:PRACTICE SET 01Exercise:PAPER 1 (PHYSICS & CHEMISTRY)
Explore 50 Videos
Similar Questions
Knowledge Check
If S=9t−3t3, then maximum velocity is
A0B−9 units / secC9 units/ SecD10 units /secA SHO has amplitude A and time period T. The maximum velocity will be
A4ATB2ATC2π√A/TD2πA/TFor a particle in SHM the amplitude and maximum velocity are A and V respectively. Then its maximum acceleration is
AV22ABV2ACV2ADVA
If S=16+64t−t3, then maximum velocity is
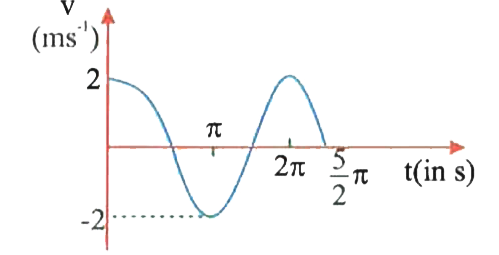
View SolutionThe velocity-time graph of a particle executing SHM is as shown in the figure. The amplitude and maximum acceleration of the particle respectively are
View SolutionEquation of displacement of a harmonic oscillator is x=15sin20πt, amplitude and angular velocity are respectively
View SolutionA simple harmonic oscillator has amplitude 2 A and maximum velocity 2 V. Then its displacement at which its velocity is V and the velocity at displacement A are
View SolutionThe displacement ξ in centimetres of a particle is ξ=3sin314t+4cos314t. Amplitude and initial phase are
View Solution