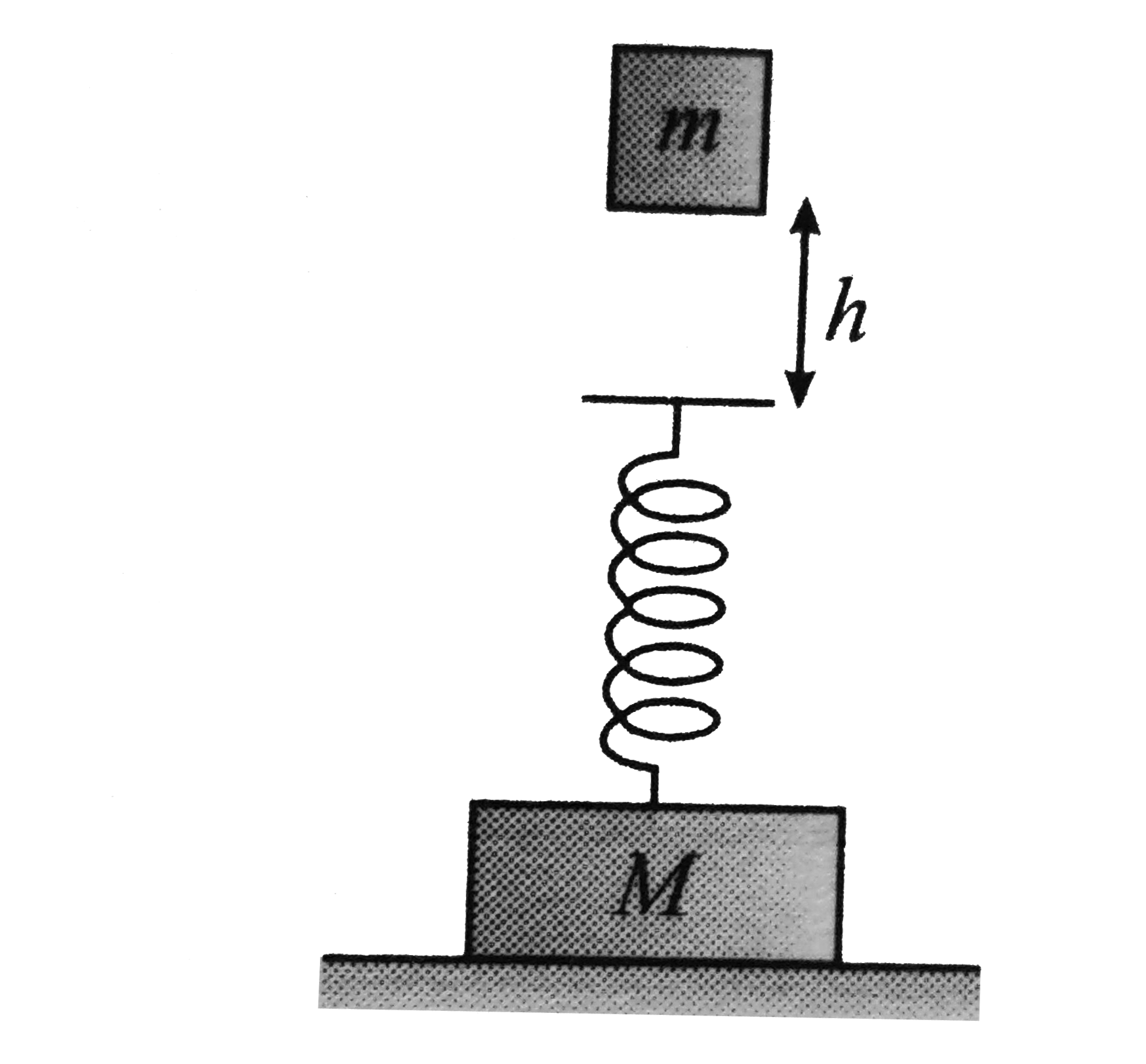
A block of mass $m$ is dropped onto a spring of constant $k$ from a height $h$ . The second end of the spring is attached to a second block of mass M as shown in figure. Find the minimum value of h so that the block M bounces off the ground. If the block of mass m sticks to the spring immediately after it comes into contact with it.

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $\frac{(M^{2} + 2 m M) g}{2 k m}$

The block $m$ drops a height h from A compresses the spring and then goes up to $B x$ above the initial level of spring. Applying work energy theorem between A and B
$W = Δ P E_{s} + Δ P E_{g} + Δ K E + w_{N C}$
$0 = - m g (h - x) + \frac{1}{2} k x^{2} + 0 + 0$
$m g (h - x) = \frac{1}{2} k x^{2}$
The minimum value of x required so that the block bounces is when the N will become zero.
$N + k x = M g$ , Here, $N = 0$

$\Rightarrow x = \frac{M g}{k}$
$m g (h - \frac{M g}{k}) = \frac{1}{2} k \cdot \frac{M^{2} g^{2}}{k^{2}}$
$\Rightarrow m g h = \frac{M^{2} g^{2}}{2 k} + \frac{m M g^{2}}{k}$
$h = \frac{(M^{2} + 2 m M) g}{2 k m}$
This is the required minimum value of h.

|

Updated on:21/07/2023

Class 11 PHYSICS WORK, ENERGY AND POWER

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A block of mass m is suspended by different springs of force constant shown in figure.

A $T_{1} = T_{2} = T_{4}$
B $T_{1} = T_{2} and T_{3} = T_{4}$
C $T_{1} = T_{2} = T_{3}$
D $T_{1} = T_{3}$ and $T_{2} = T_{4}$
Question 2 - Select One

A block of mass m initially at rest is dropped from a height h on to a spring of force constant k . the maximum compression in the spring is x then
A $m g h = \frac{1}{2} k x^{2}$
B $m g (h + x) = \frac{1}{2} k x^{2}$
C $m g h = \frac{1}{2} k {(x + h)}^{2}$
D $m g (h + x) = \frac{1}{2} k {(x + h)}^{2}$
Question 3 - Select One
A block of mass $' m'$ is attached to a spring in natural length of spring constant $' k'$ . The other end $A$ of the spring is moved with a constat velocity $v$ away from the block . Find the maximum extension in the spring.

A $\frac{1}{4} \sqrt{\frac{m v^{2}}{k}}$
B $\sqrt{\frac{m v^{2}}{k}}$
C $\frac{1}{2} \sqrt{\frac{m v^{2}}{k}}$
D $2 \sqrt{\frac{m v^{2}}{k}}$

CENGAGE PHYSICS-WORK, POWER & ENERGY-Subjective

Home
Ask Doubt
Profile