A chain of length L is placed on a horizontal surface as shown in figure. At any instant x is the length of chain on rough surface and the remaining portion lies on smooth surface. Initially x=0. A horizontal force p is applied to the chain (as shown in figure) in the duration from x=0→x=L. For chain to move with constant speed
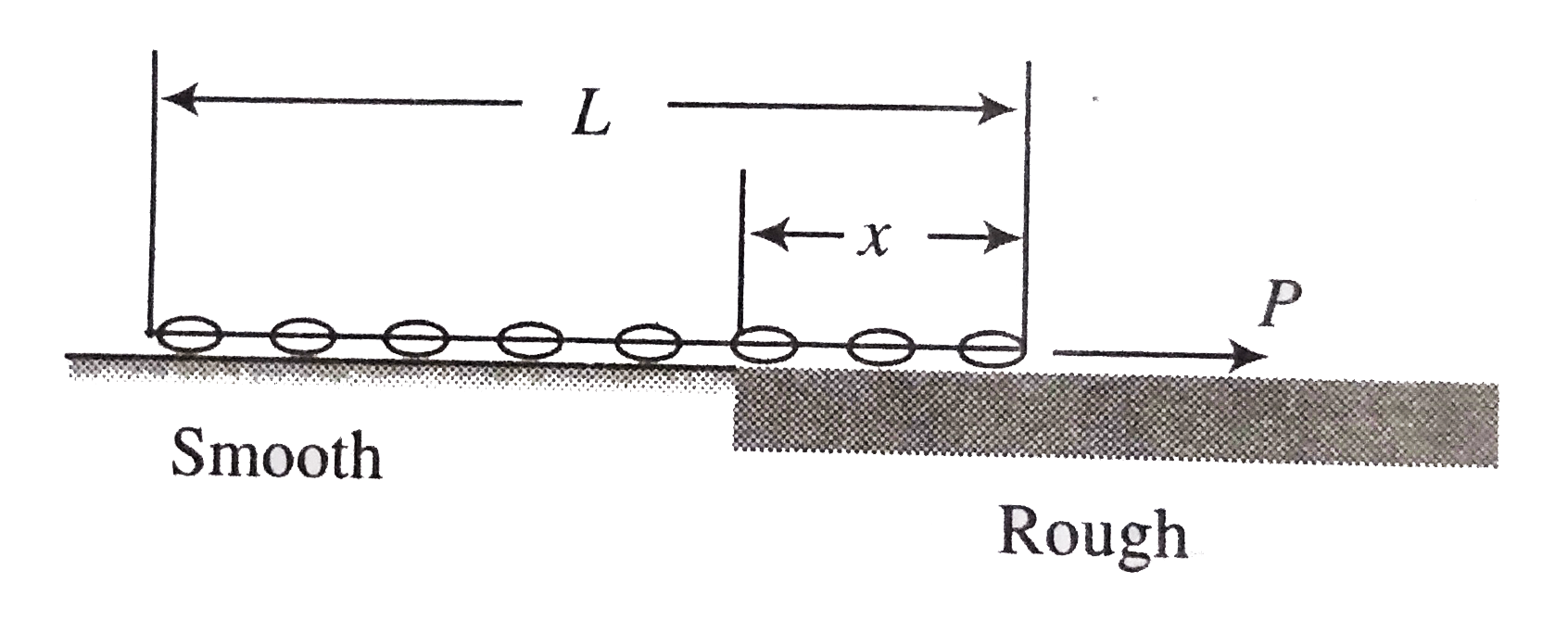
The magnitude of P should increases with time
The magnitude of P should decreases with time
The magnitude of P should increases first and then decrease with time
The magnitude of P should decrease first and then increases with time
The correct Answer is:A
For chain to move with constant speed P need to be equal to friction force on the chain As the length of chain on the rough surface increase Hence the friction force f1=μsN increase
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Multiple Correct
2 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Linked Comprehension
52 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Subjective
21 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer
5 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICSCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
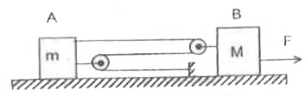
Two blocks A and B of masses m and M are attached with ideal string and pulley, and placed on smooth horizontal surface. Force F is applied horizontally as shown in figure. Find the ratio of the acceleration of A to that of B.
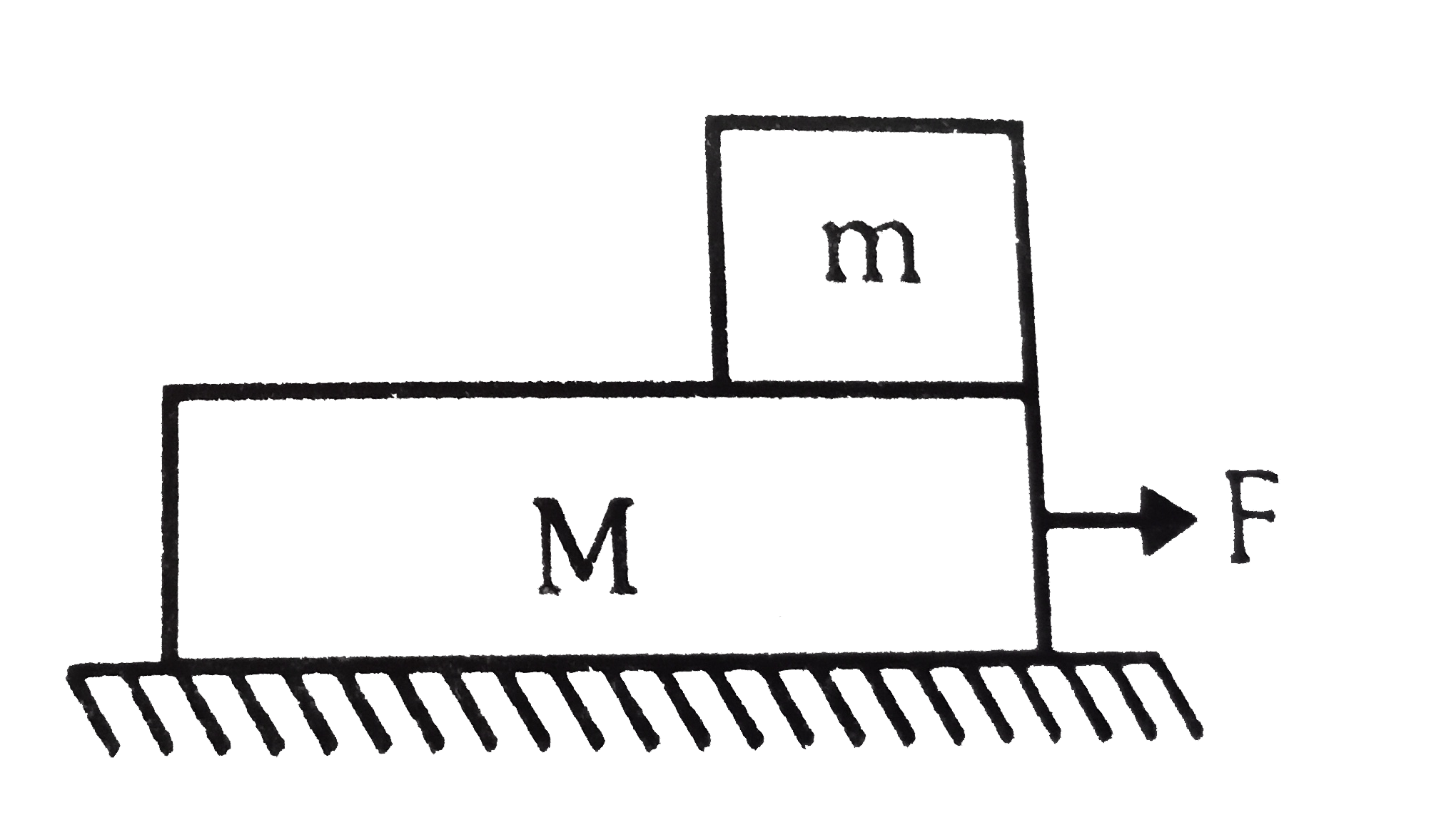
View SolutionA block of mass'm' it kept over the smooth surface of the plank of mass M. the plank of length l is kept over the smooth horizontal surface. A constant horizontal force F is applied onto the plank as shown in figure. Calculate the time after which the block falls off the plank?
View Solution
Knowledge Check
A chain of length L and mass m is placed upon a smooth surface. The length of BA is (L−b). Calculate the velocity of the chain when its and reaches B
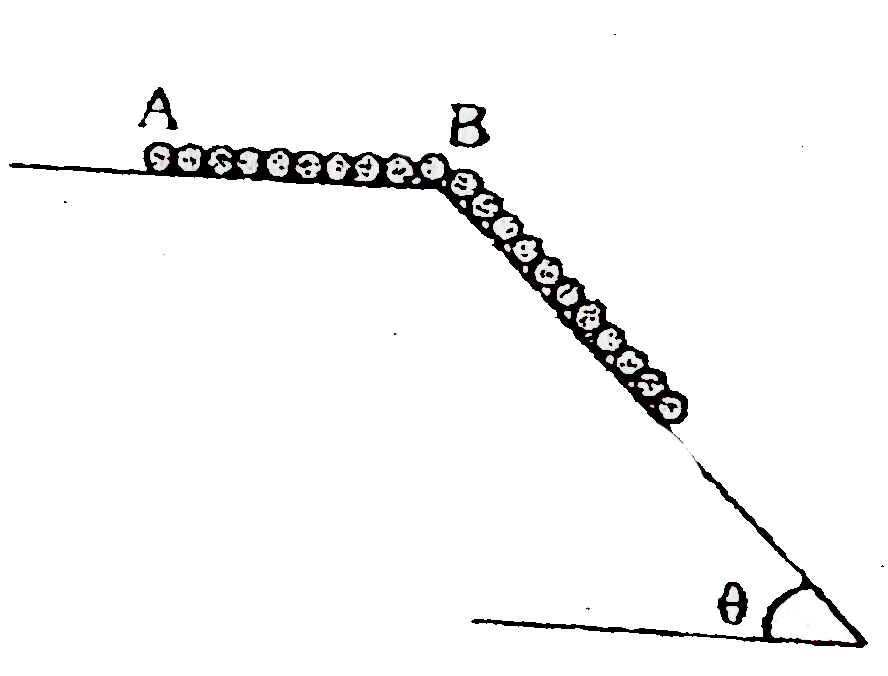 A√2gsinθL(L2−b2)B2√gsinθL(L2−b2)C√gsinθL(L2−b2)D√gsinθ2L(L2−b2)
A√2gsinθL(L2−b2)B2√gsinθL(L2−b2)C√gsinθL(L2−b2)D√gsinθ2L(L2−b2)A chain of mass M is placed on a smooth table with 1/n of its length L hanging over the edge. The work done in pulling the hanging portion of the chain back to the surface of the table is:
AMgL/nBMgL/2nCMgL/n2DMgL/2n2A rod AB of mass M and length L lies on smooth horizontal table impulse J is applied to end A as shown in the figure immediately after imparting the impulse :
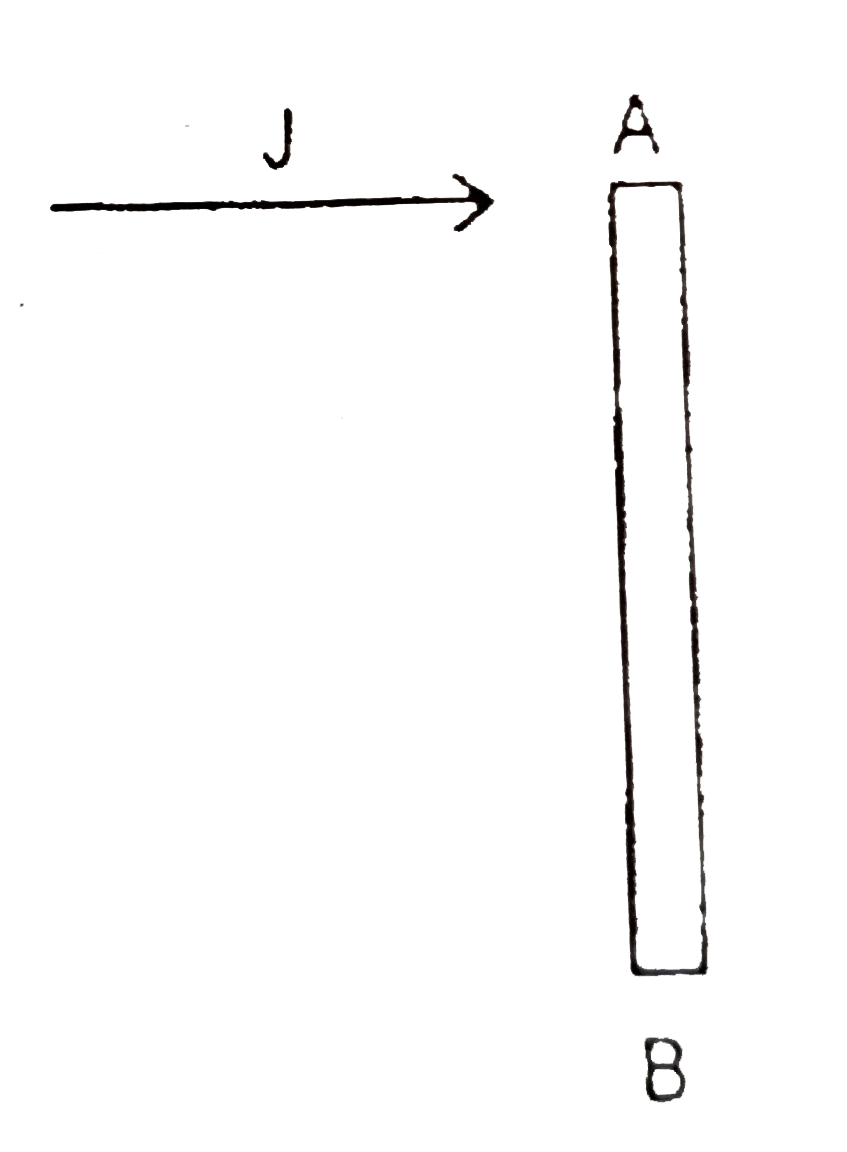 AThe radius of curvature of trajectory of A as seen from the ground is 89L.BThe radius of curvature of trajectory of B as seen from the ground is 29L.CThe instantaneous axis of rotation is at a distance of L/6 from the mid point of the rod.Dthe mid point of the rod will move along a straight line.
AThe radius of curvature of trajectory of A as seen from the ground is 89L.BThe radius of curvature of trajectory of B as seen from the ground is 29L.CThe instantaneous axis of rotation is at a distance of L/6 from the mid point of the rod.Dthe mid point of the rod will move along a straight line.
A uniform rope of length of length L is pulled by a force F on a smooth surface. Find tension in the rope at a distance x from the end where force is applied.
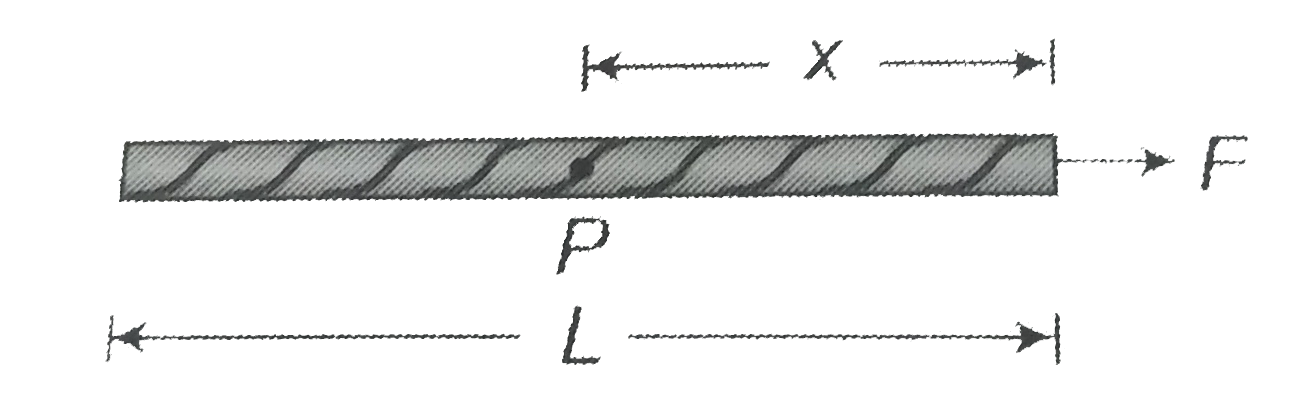
View SolutionA chain AB of length l is located in a smooth horizontal tube so that its fraction of length h hangs freely and touches the surface of the table with its end B(figure). At a certain moment the end A of the chain is set free. With what velocity will this end of the chain slip out of the tube?

View SolutionA chain of length L and mass per unit length ρ is piled on a horizontal surface. One end of the chain is lifted vertically with constant velocity by a force P.
View SolutionA uniform chain of length l is placed on a smooth horizontal table, such that half of its length hangs over one edge. It is releasedfrom rest, the velocity with which it leaves the table is
View SolutionA strip of wood mass M and length l is placed on a smooth horizontal surface. An insect of mass m starts from rest at one end of the strip and walks to the other end in time t, moving with a constant speed.
View Solution
