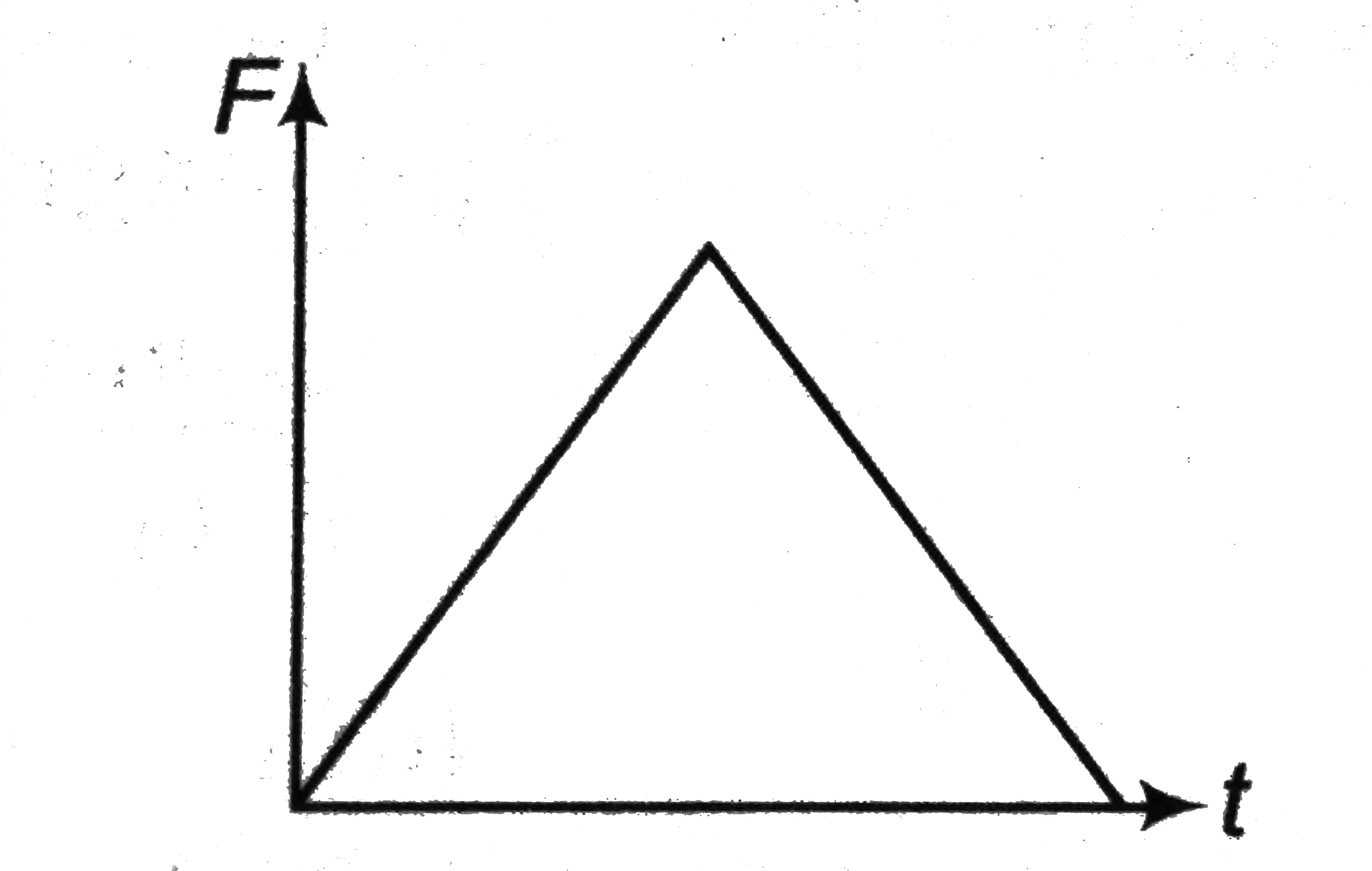
The magnitude of the net force exerted in the x direction on a 2.50-kg particle varies with the time as shown in fig., find (a) the impules of the force. (b) the final velocity the particle attains if it is originally at rest,
(c ) its final velocity if its original velocity is −2.0ms−1, and
(d) the average force exerted on the particle for the time interval between 0 and 5 s.
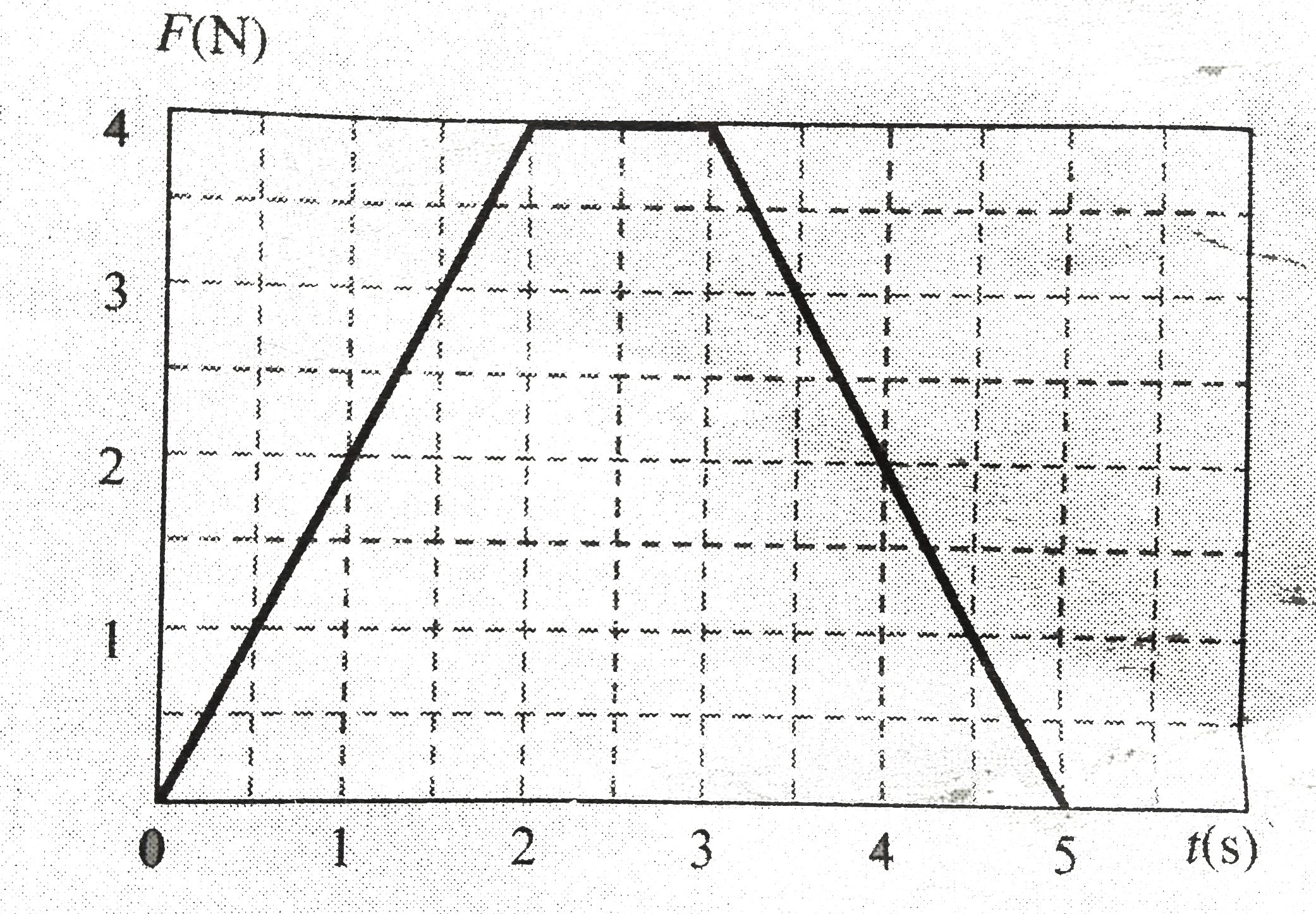
(a) The impulse is to the right and equal to the area under the F−t graph I={[5+1]/2}×4=12Ns
(b) m→vi+→Ft=m→vf
⇒2.5×0+12=(2.5)v⇒v=4.8ms−1
( c) From the same equation,
⇒2.5×(−2)+12=(2.5)v⇒v=4.8ms−1
(d) F_(avg)Delta t=12.0implies F_(avg)=2.40N.
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION 1Book:CENGAGE PHYSICSChapter:NEWTON'S LAWS OF MOTION 1Exercise:Exercise 6.2
Explore 35 Videos
- NEWTON'S LAWS OF MOTION 1Book:CENGAGE PHYSICSChapter:NEWTON'S LAWS OF MOTION 1Exercise:Exercise 6.3
Explore 19 Videos
- NEWTON'S LAWS OF MOTION 1Book:CENGAGE PHYSICSChapter:NEWTON'S LAWS OF MOTION 1Exercise:Solved Examples
Explore 11 Videos
- MISCELLANEOUS VOLUME 2Book:CENGAGE PHYSICSChapter:MISCELLANEOUS VOLUME 2Exercise:INTEGER_TYPE
Explore 10 Videos
- NEWTON'S LAWS OF MOTION 2Book:CENGAGE PHYSICSChapter:NEWTON'S LAWS OF MOTION 2Exercise:Integer type
Explore 1 Video
Similar Questions
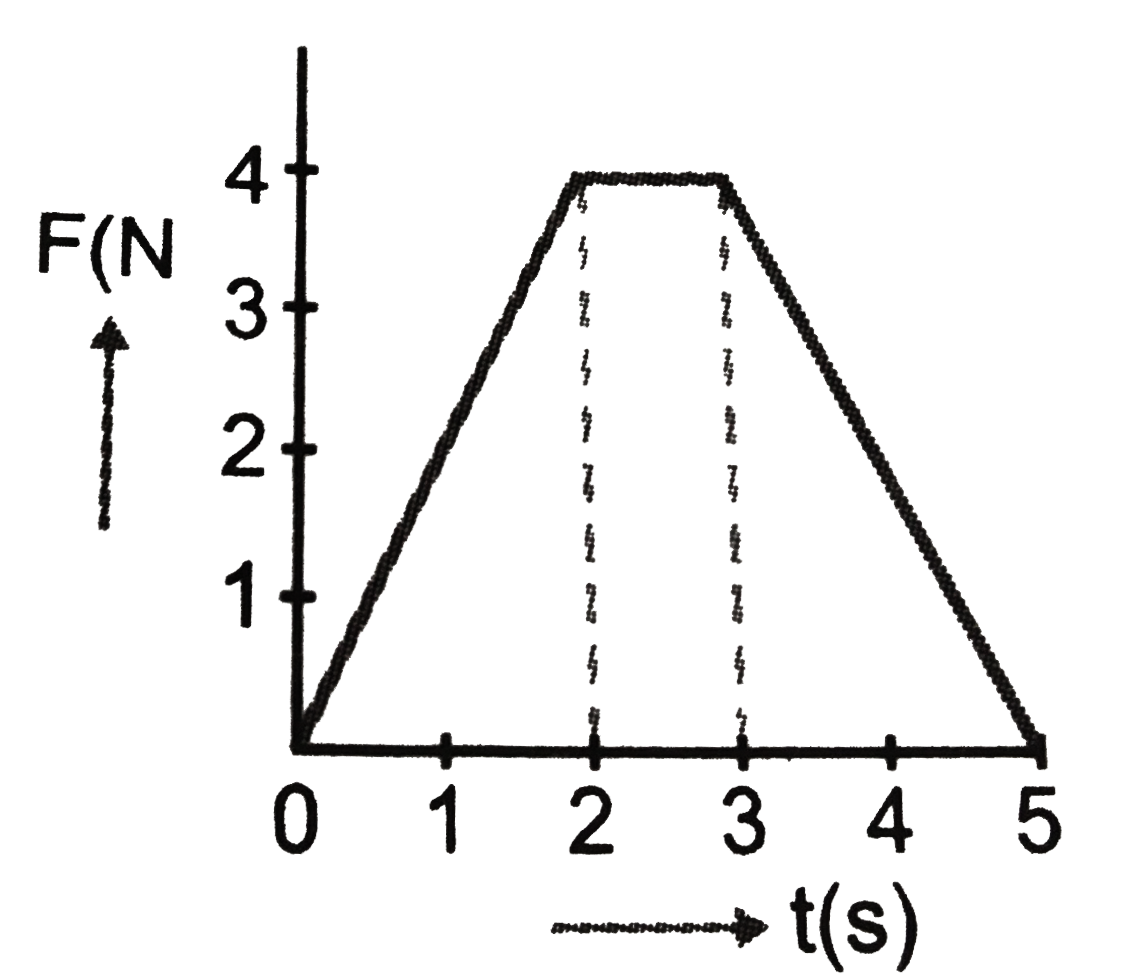
A force acting on a body of mass 2 kg varies with time as shown in fig . 20 find impulse of the force and final velocity of the body.
View SolutionThe magnitude of force acting on a particle moving along x-axis varies with time (t) as shown in figure. If at t = 0 the velocity of particle is v0, then its velocity at t=T0 will be

View Solution
Knowledge Check
Power of a force acting on a block varies with time t as shown in figure. Then, angle between force acting on the block and its velocity is
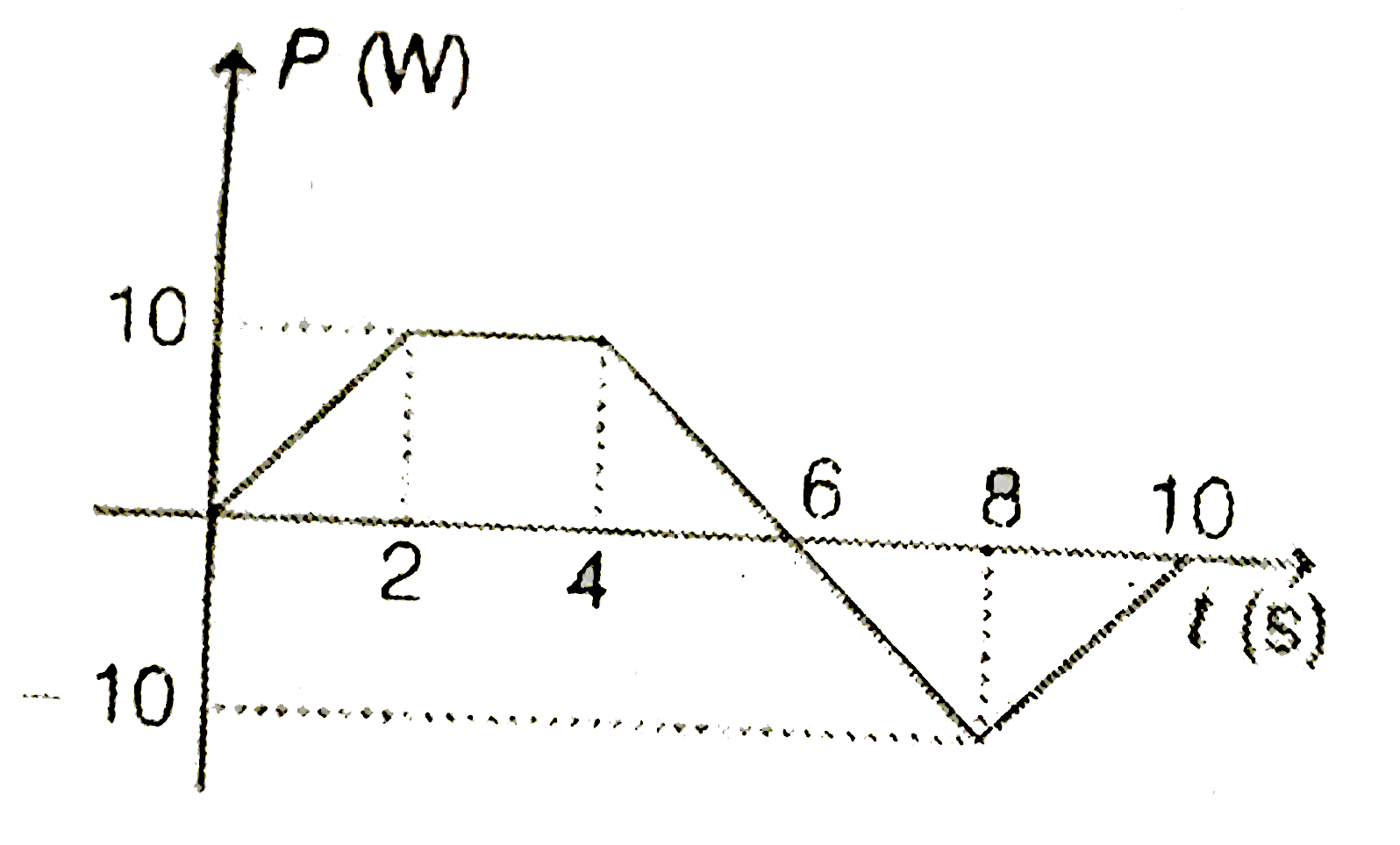 Aacute at = 1 sB90∘ at t = 3 sCobtuse at t = 7 sDchange in kinetic energy from t = 0, to t = 10 s is 20 J
Aacute at = 1 sB90∘ at t = 3 sCobtuse at t = 7 sDchange in kinetic energy from t = 0, to t = 10 s is 20 JThe velocity of a particle varies with its displacement as v=(√9−x2)ms−1.
Find the magnitude of the maximum acceleration of the particle.A3ms−2B4ms−2C3.5ms−2D5ms−2A particle starting from rest attains a velocity of 50ms−1 in 5 s. The acceleration of the particle is
A5ms2B10ms2C50ms2D100ms2
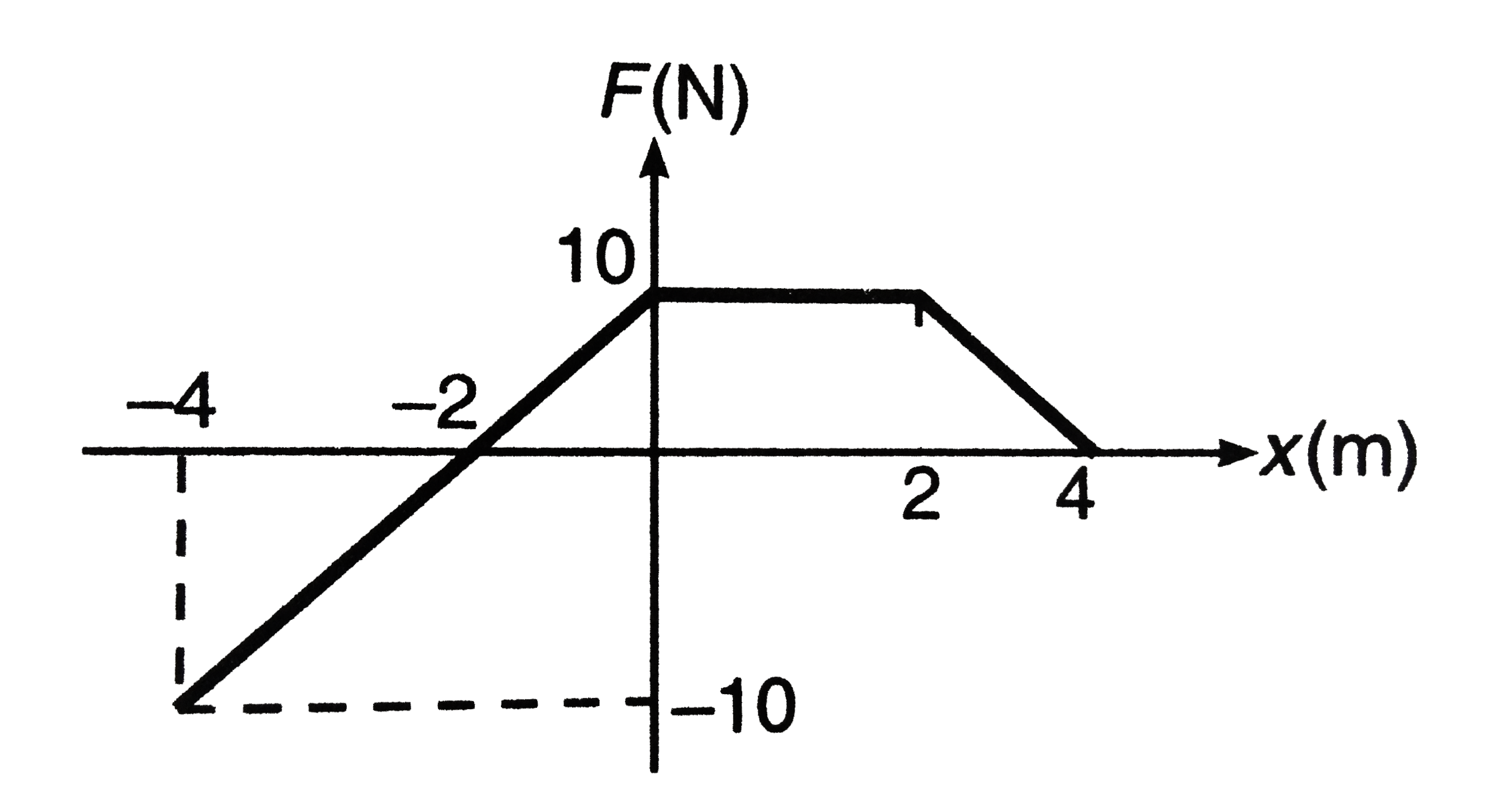
Force acting on a particle varies with displacement as shown is Fig. Find the work done by this force on the particle from x=−4m to x = + 4m.
 .
.
View SolutionA particle of mass 2kg is initially at rest. A force starts acting on it in one direction whose magnitude changes with time. The force time graph is shown in figure. Find the velocity of the particle at the end of 10s.

View SolutionA particle of mass 2kg is initially at rest. A force starts acting on it in one direction whose magnitude changes with time. The force time graph is shown in figure. Find the velocity of the particle at the end of 10s.

View SolutionThe net force acting on a particle moving along a straight line varies with time as shown in the diagram. Force is parallel to velocity. Which of the following graph is best representative of its speed with time? (Initial velocity of the particle is zero)
View SolutionThe velocity of a particle varies with time as →v=3ˆi+(4−5t)ˆjms−1. Find the average velocity of the particle for a time interval between t=0 and a time when the speed of the particle becomes minimum.
View Solution
