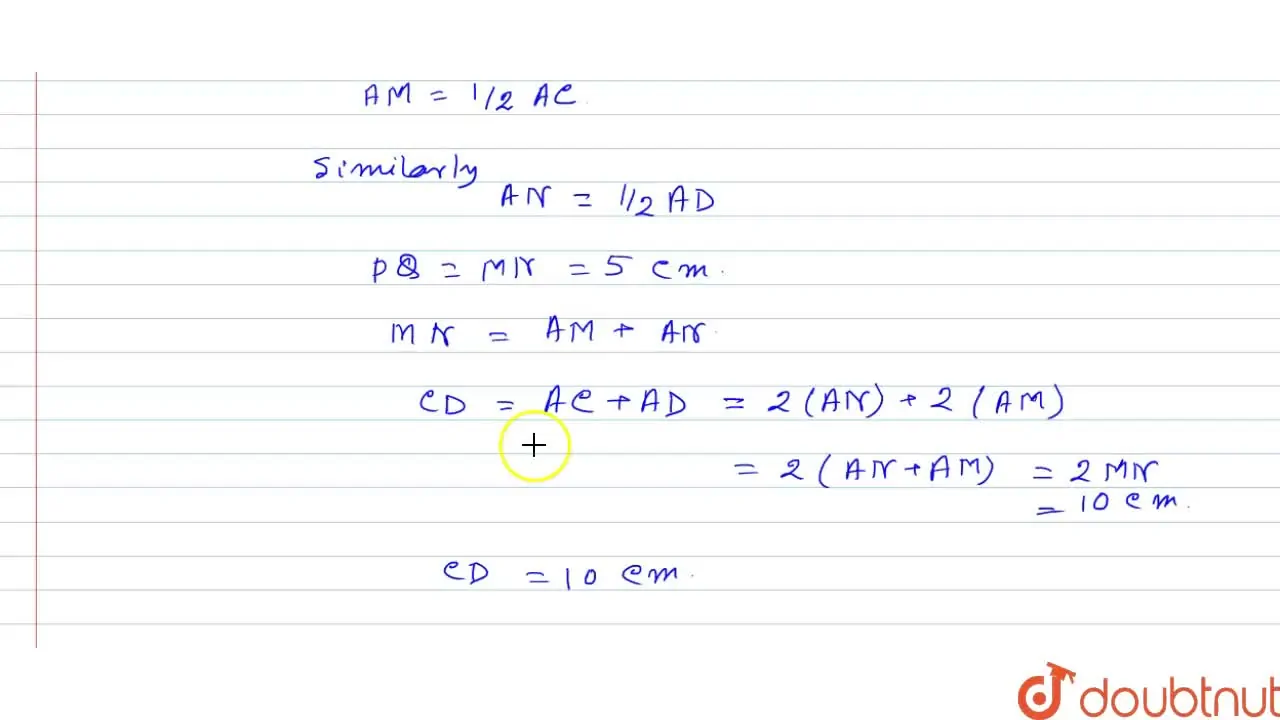
Two two circle with their centre at P and Q intersect each other, at the point A and B . Through the point A , a straight line parallel to PQ intersects the two circles at the points C and D respectively . If PQ = 5 cm , then determine the lenght of CD . Solution in Bengali
Let PM⊥CDand QN⊥CD
∵PQ∣∣CD,∴PQ=MN
Now , PQ=5cm,∴MN=5cm
Also MN = AM + AN
or MN=12AC+12AD[∵PM⊥AC⇒AM=12ACandQN⊥AD⇒AN=12AD.]orMN=12(AC+AD)
or 5=12×CD[∵AC+AD=CD]orCD=5×2=10
∴ lenght of hte chord CD = 10 .

Related Playlists
- BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO CIRCLE EXERCISE - Example (Long-Answer Type Questions)10videos
THEOREMS RELATED TO CIRCLE
- BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO CIRCLE EXERCISE - Example (B. Write true or false )3videos
THEOREMS RELATED TO CIRCLE
- BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO CIRCLE EXERCISE - EXERCISE - 1 (Long - answer type question )9videos
THEOREMS RELATED TO CIRCLE
- BOOK - CALCUTTA BOOK HOUSECHAPTER - SPHEREEXERCISE - EXERCISE36videos
SPHERE
- BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO CYCLIC QUADRILATERAL EXERCISE - Long -answer type questions (L.A.)12videos
THEOREMS RELATED TO CYCLIC QUADRILATERAL
Similar Questions
The length of the radius of circle is 5 cm and length of its chord AB is 8 cm . Calculate the distance of the chord AB form the centre O.
04:59View SolutionOnly one circle can be throught three collinear points .
05:07View SolutionProve that diameter is the greatest chord of a circle.
04:49View SolutionIf the volume of a solid hemisphere be 19404 cc, then the radius of the hemisphere will be
04:44View SolutionIn a cyclic quadrilateral, the ratio of three consecutive angle is 1 : 2 : 3 Find the value of first and third angles.
07:47View SolutionTwo circles intersect each other at the points P and Q. Two straight lines through P and Q intersect one circle at the points A and C and the other circle at B and D. Prove the AC|| BD
09:27View SolutionThe centre of two circle are P and Q they intersect at the points A and B. The straight line parallel to the line segment PQ through the point A intersects the two circles at the point C and D Prove that CD=2PQ
06:44View SolutionAnkita drew two circles which intersect each other at the points P and Q. Through the point P two straight lines are draw so that they intersect one of the circle at the points. A and B and the ohte circle at the points C and D respectively. Prove that ∠AQC=∠BQD.
View SolutionTwo circles intersect each other at the points P and Q. A straight line passing through P intersects one circle at A the other circle at .A straight line passing through Q intersect the first circle at C and the second circle at D. Prove that AC∣∣BD.
19:41View SolutionTwo circles intersect each other at the points P and Q. Two straight lines through P and Q intersect on circle at the points A and C and the other circle at B and D . Prove that AC||BD.
08:51View SolutionTwo circles intersect each other at the points G and H . A straight line is drawn through the point G which intersect two circles at the points P and Q and the straight line through the point H parallel to PQ intersects the two circles at the points R and S . Prove that PQ=RS .
05:07View SolutionIn two circles , one circle passes through the centre O of the other circle and they intersect each other at the points A and B . A straight line passing through A intersect the circle passing through O at the point P and the circle with centre at O at the point R . BY joining P , B and R , B prove that PR= PB.
06:31View SolutionAs shown in the adjioning figure, two circles interset each other in points A and B. Two tangents touch these in point these circles in points P,Q and R, S as shown . Line AB intersects seg PQ in C and seg RS in D. Show that Cand D are midpoints of seg PQ and seg RS respectively.
02:59View Solutionகொடுக்கப்பட்ட படத்தில் O மையமாக உடைய வட்டத்தின் ஆரம் 5 செ .மீ ஆகும் .T ஆனது OT =13 செ .மீ என அமைந்த ஒரு புள்ளி மற்றும் OT ஆனது வட்டத்தை E ல் வெட்டுகிறது,வட்டத்தின் E என்ற புள்ளியின் வழியாக செல்லும் ஒரு தொடுகோடு AB எனில் ,AB -யின் நீளம் காண்க ?
03:51View SolutionTwo equal circles are centered on each other and the two circles intersect at points A and B. By intersecting two straight line circles at points C and points D, we prove that, triangleBCD Equilateral triangle.
03:53View Solution