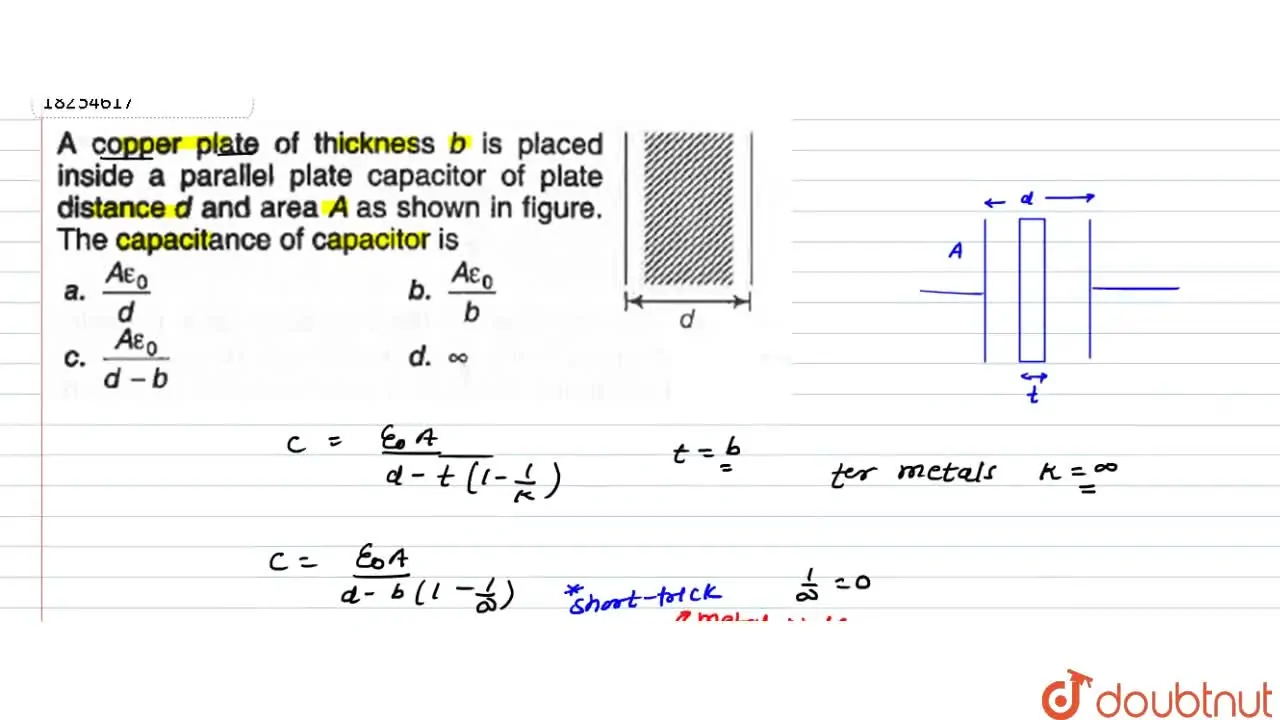
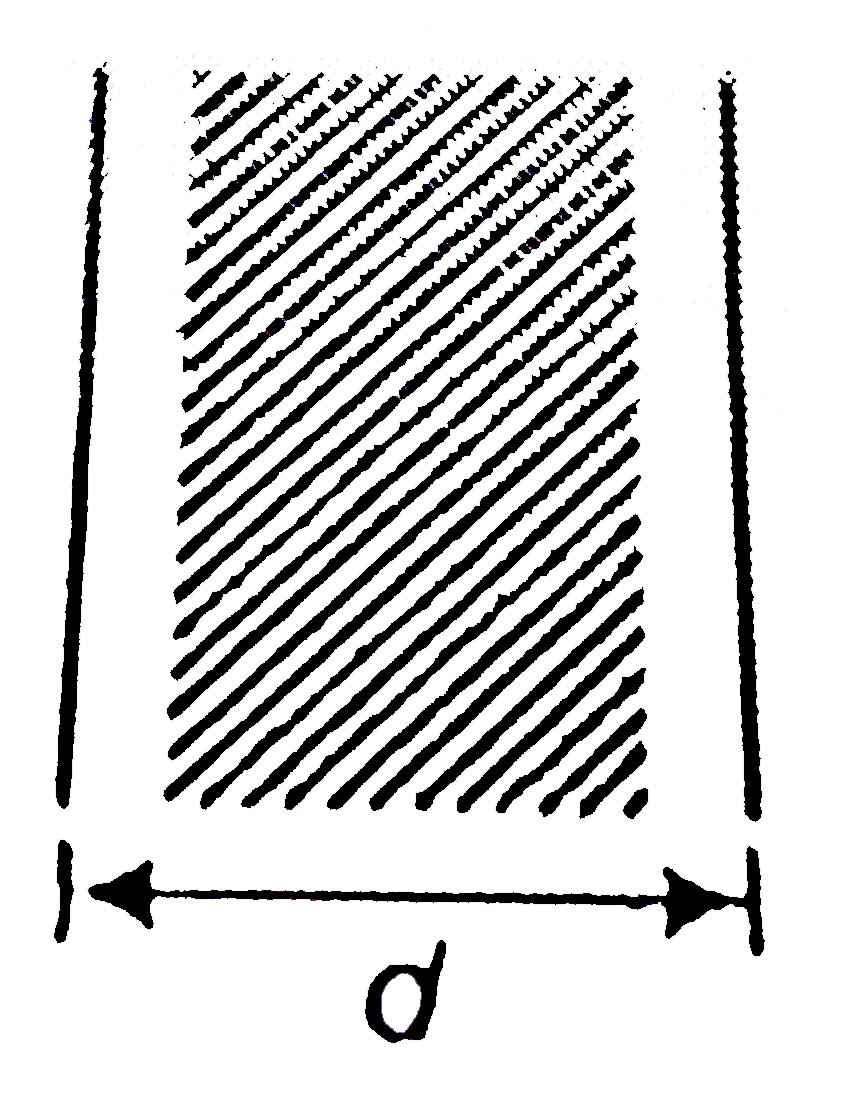
A copper plate of thickness b is placed inside a parallel plate capacitor of plate distance d and area A as shown in figure. The capacitance of capacitor is
ε0Ad+b2
ε0A2d
ε0Ad−b
2ε0Ad+b2
The correct Answer is:C
As capacitance Co=ε0Ad
∴ after inserting copper plate C=ε0Ad−b
Related Playlists
- BOOK - NCERT FINGERTIPSCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Combination Of Capacitors14videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
- BOOK - NCERT FINGERTIPSCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Energy Stored In Capacitor18videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
- BOOK - NCERT FINGERTIPSCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - The Parallel Plate Capacitor5videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
- BOOK - NCERT FINGERTIPSCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Assertion And Reason15videos
ELECTROMAGNETIC WAVES
- BOOK - NCERT FINGERTIPSCHAPTER - MAGNETISM AND MATTER EXERCISE - Assertion And Reason15videos
MAGNETISM AND MATTER
Similar Questions
In a parallel plate capacitor, the capacitance
View SolutionA sheet of aluminium foil of negligible thickness is placed between the plates of a capacitor of capacitance C as shown in the figure, the capacitance of capacitor becomes
 View Solution
View SolutionA sheet of aluminium foil of negligible thickness is placed between the plates of a capacitor of capacitance C as shown in the figure then capacitance of capacitor becomes
 View Solution
View SolutionFind out the capacitance of parallel plate capacitor of plate area A and plate separation d.
View SolutionA dielectric slab is placed between the plates of a parallel plate capacitor. Its capacitance
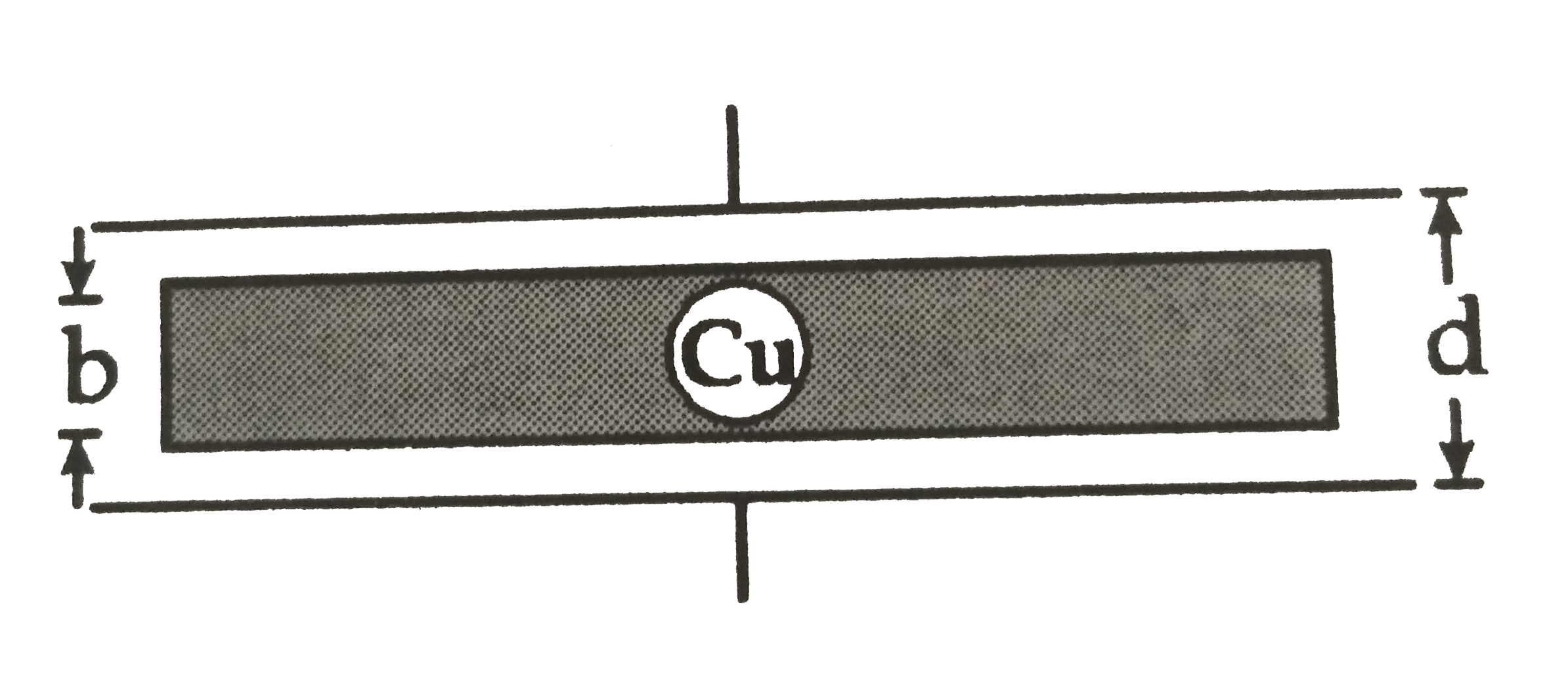
View SolutionA slab of copper of thickness b is inserted in between the plates of a parallel plate capacitor as shown in figure. The separation of the plates is d. If b=d/2, then the ratio of capacities of the capacitor after and before inserting the slab will be
 View Solution
View SolutionA slab of copper of thickness b is inserted in between the plates of parallel plate capacitor as shown in figure. The separation of the plate is d. If b=d/2, then the ratio of capacities of the capacitor after and before inserting that slab will be
 View Solution
View SolutionA sheet of copper is inserted in the air gap of a parallel plate capacitor, without touching any of the two plates of the capacitor. The capacitance of the capacitor is
View SolutionA conducting slab of thickness ‘t’ is introduced between the plates of a parallel plate capacitor, separated by a distance d (t<d). Derive an expression for the capacitance of the capacitor. What will be its capacitance when t = d ?
View SolutionA conducting slab of thickness 't' is introduced without touching between the plates of a parallel plate capacitor , separated by a distance 'd' (t<d) . Derive an expression for the capacitance of the capacitor .
View Solution