A compound formed by elements X and Y has a cubic structure in which X atoms are at the corner of the cube and also at the face centers. Y atoms are present at the body centre and at the edge centre of the cube.
a. Celculate (i) Zeff' (ii) total number of atoms in the cube, and (iii) formula of the compound.
b. If all the atoms are removed from one of the body diagonals of the cube, calculate (i) Zeff' (ii) total number of atoms in the cube, and (iii) formula of the compound.
c. If all the atoms from the diagonals of the one of the face of the cube are removed, calculate (i) Zeff' (ii) total number of atoms in the cube, and (iii) formula of the compound.
d. If all the atoms are removed from one of the plane passing through the middle of t he cube, calculate (i) Zeff' (ii) total number of atoms in the cube, and (iii) formula of the compound.
e. If all the atoms are removed from one of the axes passing through one of the face centres of the cube, calculate (i) Zeff' (ii) total number of atoms in the cube, and (iii) formula of the compound.
a. Zeff(X)=nc8+nf2=88+62=1+3=4
Zeff(Y)=nb8+ne4=11+124=1+3=4.
Zeff(X+Y)=4+4=8
ii. Total number of atoms in the cube
ii. =8 (corners) +6(face centre)+1 (body centre)
+12 (edge centre)
=27 atoms/units cube
(iii). Formula: Zeff(X)=4,Zeff(Y)=4
implies X4Y4=4XY
b. i.
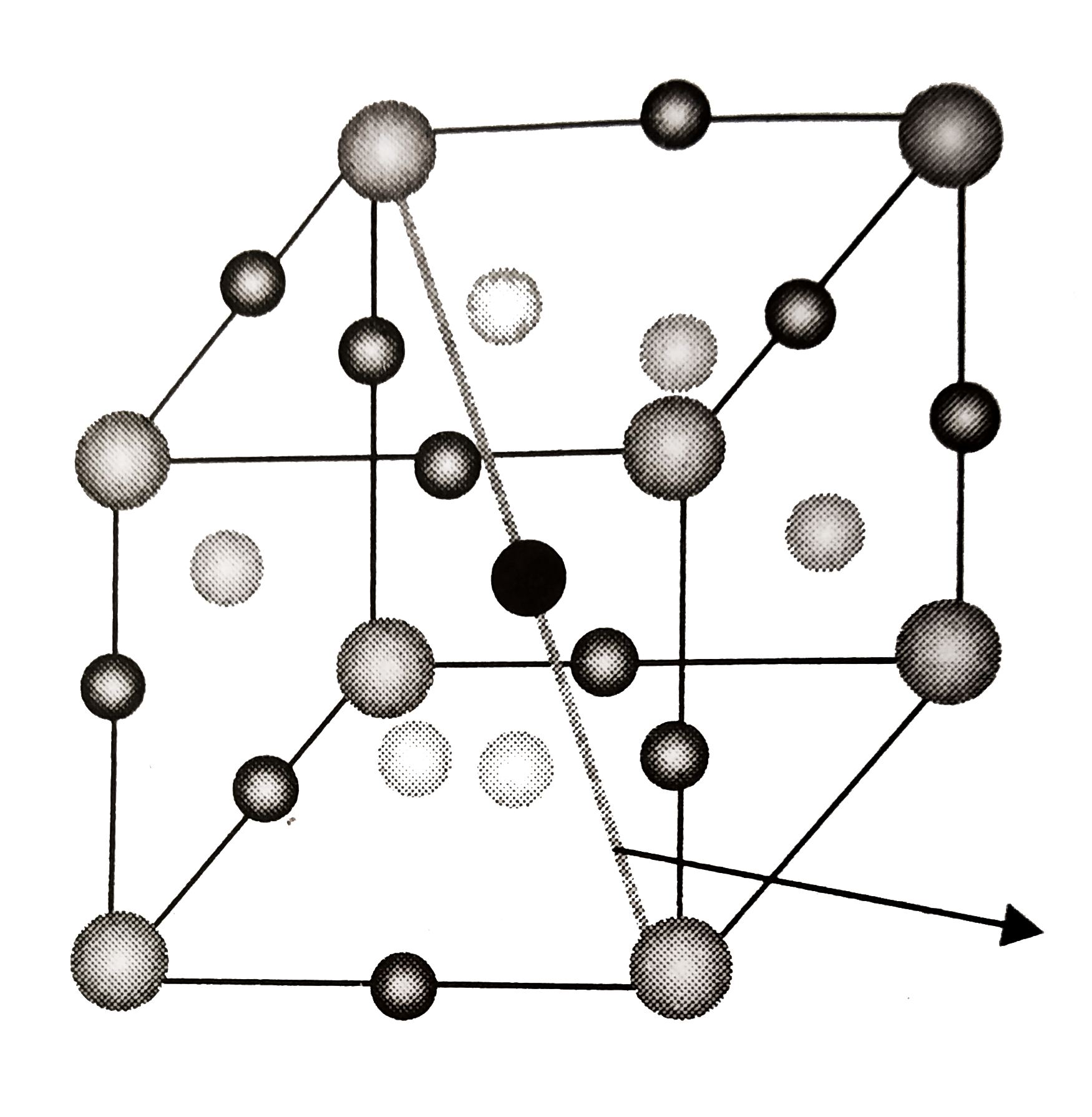
Atoms removed from one body diagonal
=2 atom form corner +1 atom form body centre
=3 atoms. Zeff(X)=nc8+nf2=8−28+62=154
Zeff(Y)=nb8+nc4=1−11+124=0+3=3
Zeff(X+Y)=154+3=274
ii. Total number of atoms in the cube
=6(corner)+6(face centre)+ Zero (body centre) +12 (edge centres)
=24 atoms/unit cube
iii. Zeff(X)=154,Zeff(Y)=3
⇒X(154)Y3.
Simplifying X15Y12
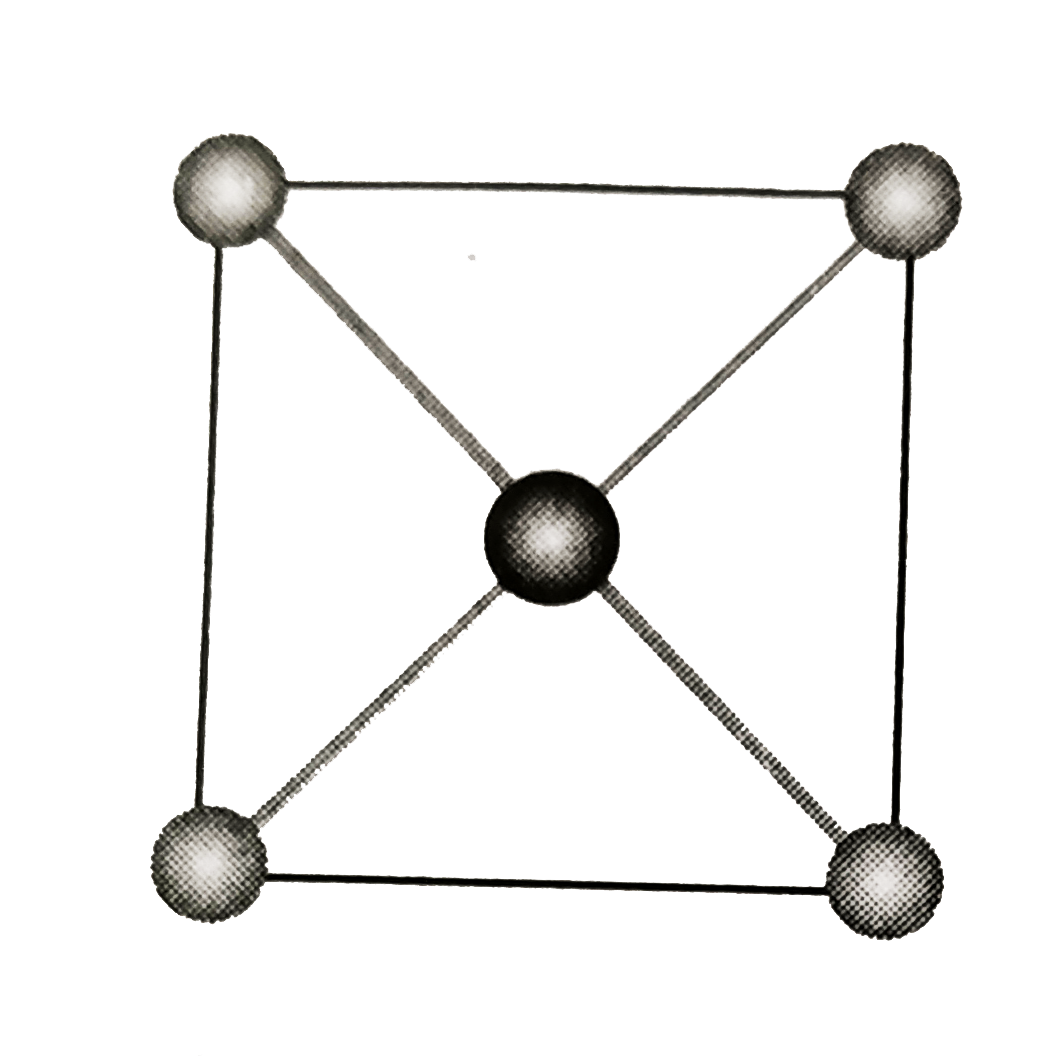
Zeff(X)=nc8+nf2=8−48+6−12=1+2=3.
Zeff(Y)=nb8+nc4=1−11+124=1+3=4
Zeff(X)=3+4=7
ii. Total number of atoms in a cube
=4(corner)+5(face centre)+1 (body centre)
+12 (edge centre)
=22 atoms/unit cube
iii. Formula: Zeff(X)=3,Zeff(Y)=4
⇒X3Y4.
d. Plane passing through the middle of cube (other atoms are not shown)
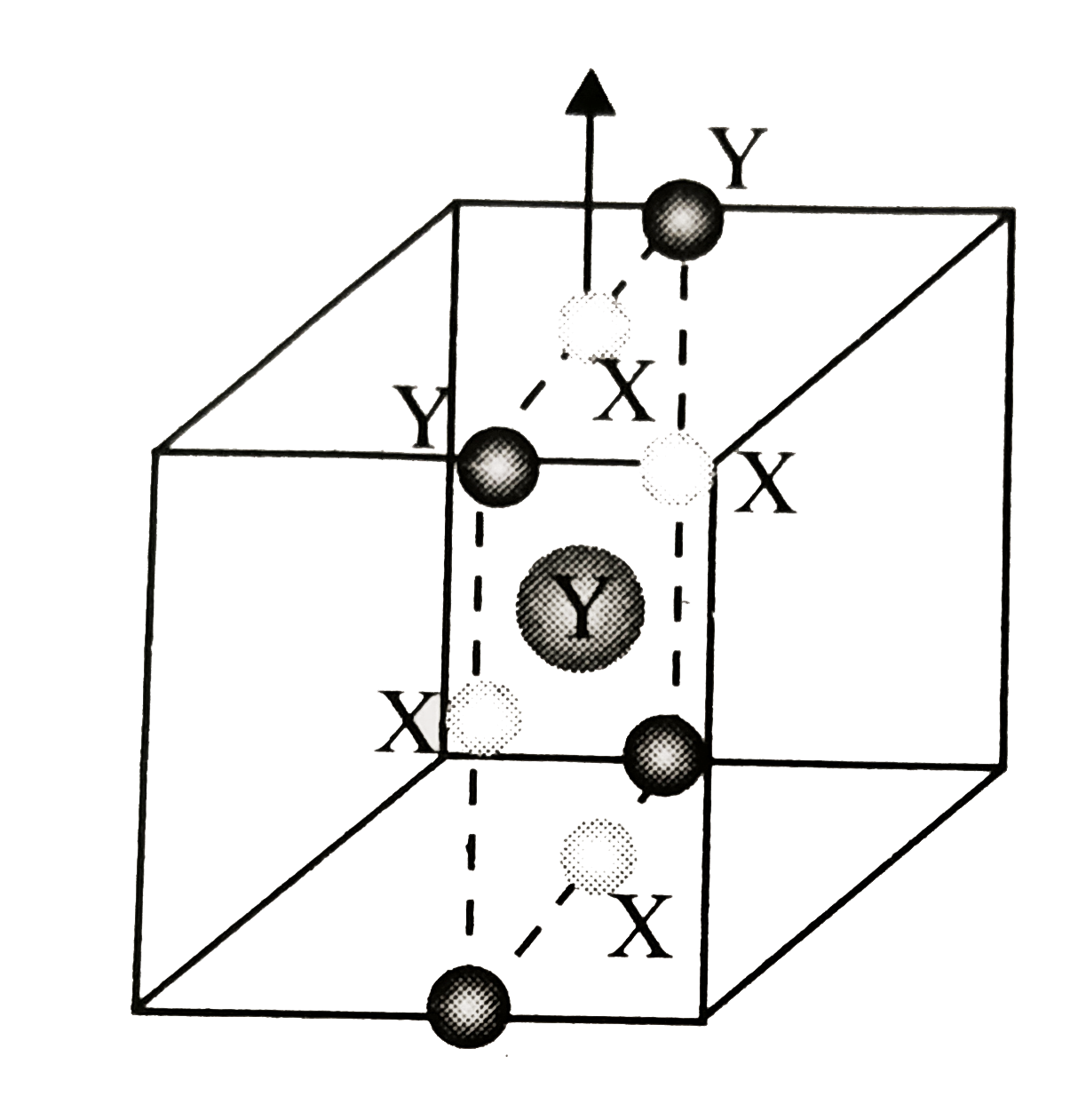
i. Zeff(X)=nc8+nf2=88+6−42=1+1=2
Zeff(Y)=nb8+nc4=(1−1)1+(12−4)4=2.
Zeff(X+Y)=2+2=4
ii. Total number of the atoms in the cube
=8(corner)+2(face centres)+ zero (body centre) +8 (edge centres)
=18 atoms/unit cube
iii. Formula: Zeff(X)=2,Zeff(Y)=2
e. 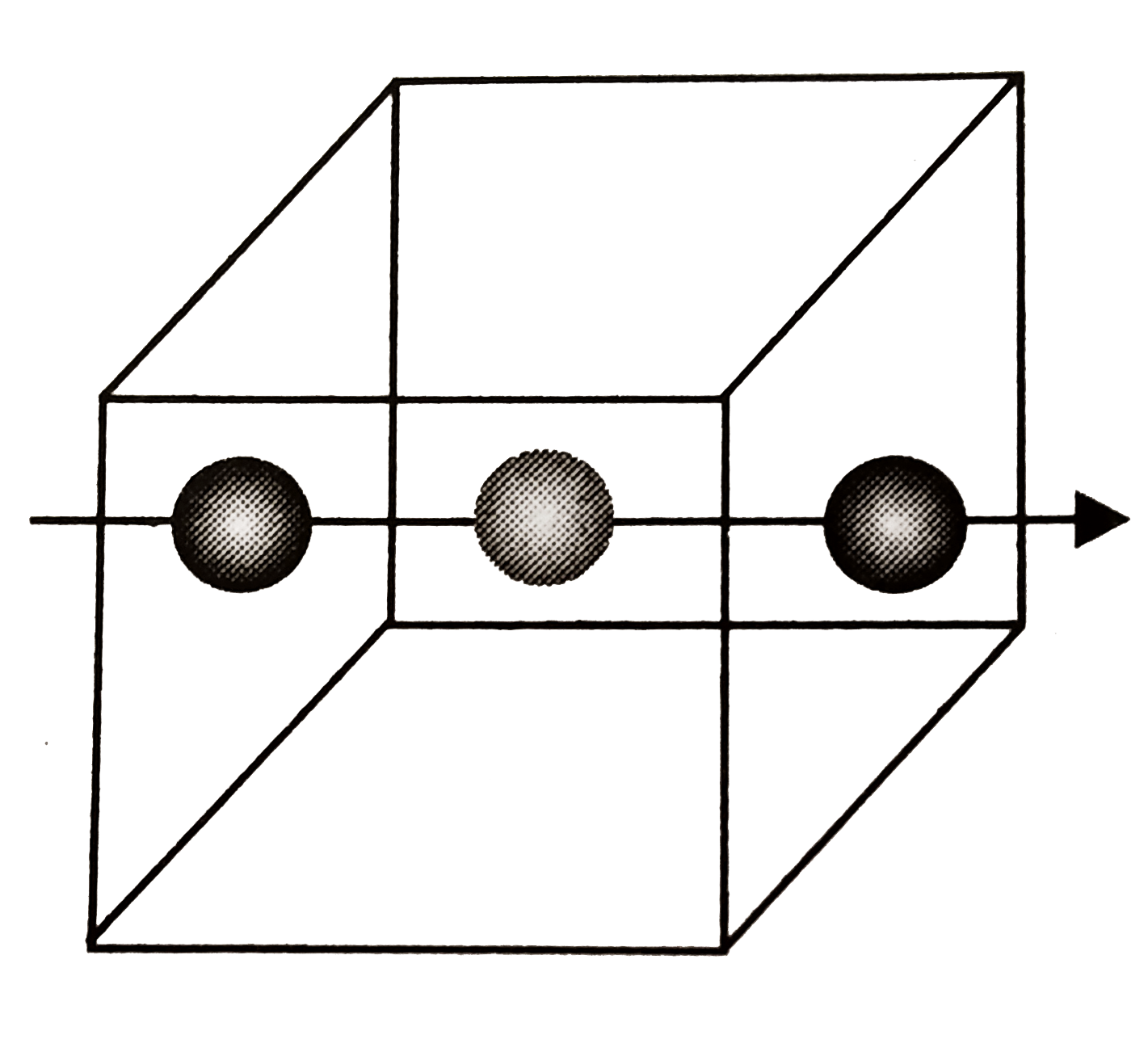
Zeff(X)=nc8+nf2=88+(6−2)2=1+2=3
Zeff(Y)=nb8+nc4=(1−1)1+124=0+3=3.
Zeff(X+Y)=3+3=6.
ii. Total number atom in a cube =8 (corner) +6 (face centre)
zero (body centre) +12 (edge centre)
=24 atoms/unit cube
iii. Formula: Zeff(X)=3,Zeff(Y)=3
Formula is X3Y3 or 3XY.
Topper's Solved these Questions
- SOLID STATEBook:CENGAGE CHEMISTRYChapter:SOLID STATEExercise:Solved Examples
Explore 13 Videos
- SOLID STATEBook:CENGAGE CHEMISTRYChapter:SOLID STATEExercise:Exercises (Linked Comprehension)
Explore 13 Videos
- REDUCTION AND OXIDATION REACTION OF ORGANIC COMPOUNDSBook:CENGAGE CHEMISTRYChapter:REDUCTION AND OXIDATION REACTION OF ORGANIC COMPOUNDSExercise:SUBJECTIVE TYPE
Explore 3 Videos
- SOLUTIONSBook:CENGAGE CHEMISTRYChapter:SOLUTIONSExercise:Ex 2.3 (Objective)
Explore 9 Videos
Similar Questions
A compound formed by elements X and Y has a cubic structure in which X atoms are at the corner of the cube and Y atoms are at the face centres. One atoms X is misssing from the corner.
a. Calculate (i). Zeff' (ii). total number of the atoms in the cube, and (iii). formula of the compound.
b. If alll the atoms are removed from one of the faces of the cube containing atoms at corners, as in (a) above, calculate (i) Zeff' (ii) total number of atoms in a cube, and (iii) formula of the compound.
View SolutionA compound formed by elements A and B has a cubic structure in which A atoms are at the corner of the cube and B atoms are at the face centres. Derive the fomula of the compound.
View Solution
Knowledge Check
A compound formed by elements X and Y has a cubic structure in whch X atoms are at the corner of the cube and also at the face centers. Y atoms are present at the body center and at the edge center of the cube.
If all the atoms from the diagonals of one of the face of the cube are removed, then Zeff isA3B4C7D8A compound formed by elements A and B has a cubic structure in which A atoms are at the corners of the cube and B atoms are at the face centres. The formula of the compound will be
AA8B6=A4B3BA8B4=A2BCAB3DA2B3A compound formed by elements X and Y has a cubic structure in whch X atoms are at the corner of the cube and also at the face centers. Y atoms are present at the body center and at the edge center of the cube.
If all the atoms are removed from one of the axis passing through one of the face centers of the cube, then formula of the compound isAXYBXY2CX2YDXY3
A compound formed by elements X and Y has a cubic structure in which X atoms are at the face centres.
a. Calculate: (i) Zeff' (ii) total number of atoms in a cube, and (iii) formula of the compound.
b. If all the atoms are removed from a single axis passing through the centre of the cube, calculate
(i) Zeff' (ii) total number of atoms in a cube, and (iii) formula of the compound.
View SolutionA compound formed by elements X and Y has a cubic structure in which X atoms are at the corner of the cube and also at alternate face entres. Y atoms are present at the body centre and also at the alternate edge centre of the cube.
a. Calculate (i) Zeff' (ii) total number of atoms in the cube, and (iii) formula of the compound.
b. If all the atoms are removed from one of the plane passing through the middle of the cube of the cube which contains atoms both on the edge entre as well as on the face centre, calculate (i) Zeff' (ii) total number of atoms in the cube, (iii) formula of the compound.
c. If all the atoms are removed from the plane passing through the middle of the cube which contains atoms only on the edge centre but not on face centre, calculate (i) Zeff' (ii) total number of atoms in t he cube, and (iii) fomula of the compound.
d. If all the atoms are removed from one of the plane passing through the middle of the cube which neither contains atoms on the edge centre nor on the face centre, calculate (i) Zeff' (ii) total number of atoms in the cube, and (iii) formula of the compound.
e. If all the atoms are removed from on e of the axes passing through the middle of the cube containing face centre atoms, calculate (i) Zeff' (ii) total number of atoms in a cube, and (iii) formula of the compound.
f. If all the atoms are remove from one of the axes passing through the middle of the cube nor containing face centre atoms, calculate (i) Zeff' (ii) total number of atoms in a cube, and (iii) formula of the compound.
View SolutionA compound formed by elements A and B has a cubic structure in which A atoms are at the corner of the cube and B atoms are at the face centres. Derive the fomula of the compound.
View SolutionA compound formed by elements A and B has cubic structure in which atoms are at the corners of the cube and B atoms are at the face centre. Derive the formula of the compound.
View SolutionA compound formed by elements X and Y has a cubic structure in which X atoms are at the corners of the cube of and two atoms (Y) are at each body diagonal of the cube.
a. Calculate: (i) Zeff' (ii) total number of atoms in a cube, and (iii) formula of the compound.
b. If all atoms form one body diagonal of the cube except corners are removed, calculate: (i) Zeff' (ii) total number of atoms in the cube, and (iii) formula of the compound.
View Solution