If f0=5c,λ6000Å,a=1cm for a microscope, then wht will be its resolving power
11.9×105/m
10.9×105/m
10.9×104/m
10.9×103/m
The correct Answer is:B
R.P=2μsinθ1.22λ
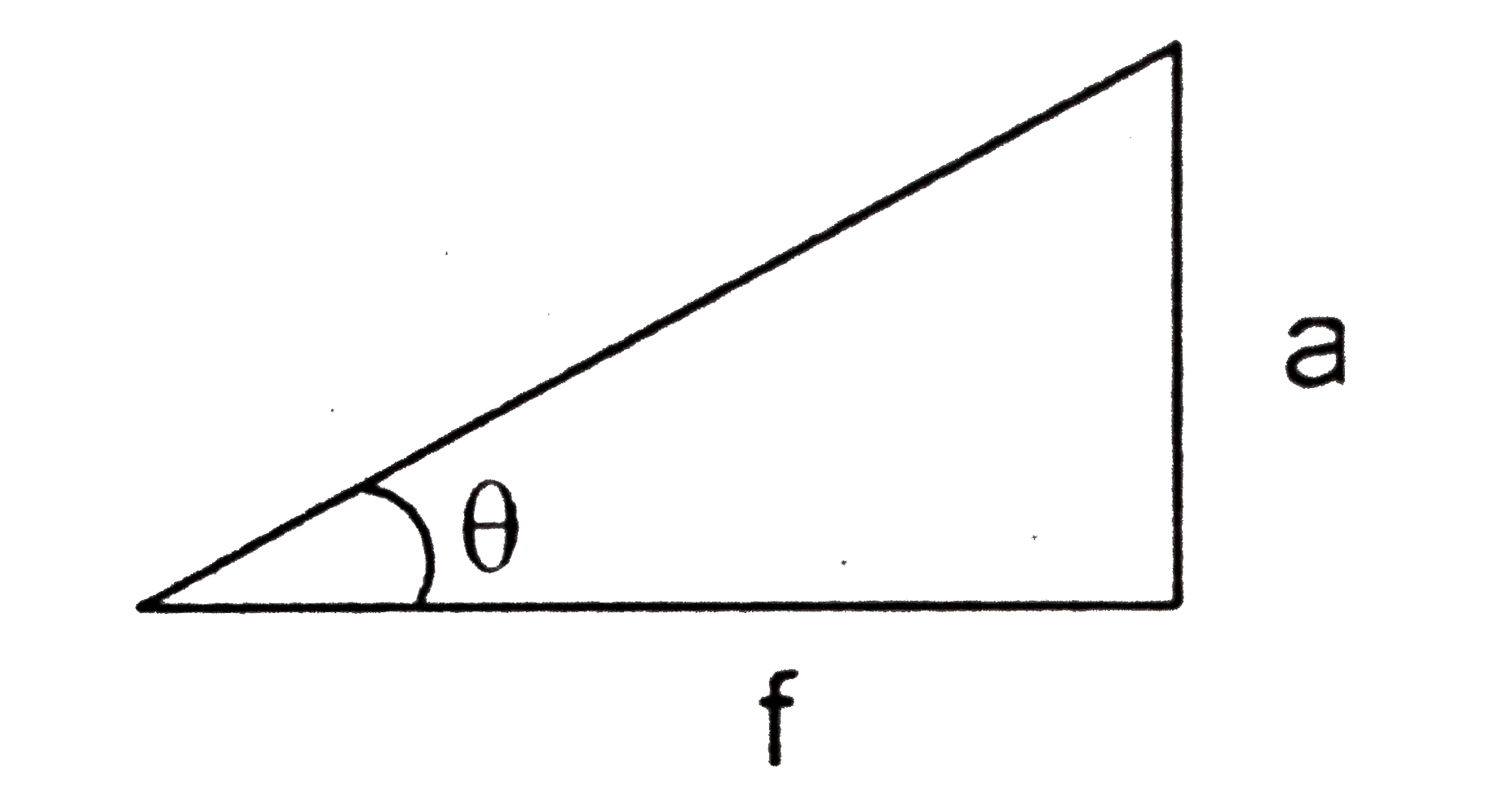
tanθ≅sinθ=af=15=0.2 ⇒ R.D=2×1×0.21.22×6×10−7=4×1063.66
RP=10.9×105/m
Topper's Solved these Questions
Similar Questions
When an object is viewed with a light of wavelength 6000Å under a microscope, its resolving power is 104. The resolving power of the microscope when the same object is viewed with a light of wavelength 4000Å, is n×103. The vlaue of n is
View SolutionWhen an object is viewed with a light of wavelength 6000Å under a microscope, its resolving power is 104. The resolving power of the microscope when the same object is viewed with a light of wavelength 4000Å, is n×103. The vlaue of n is
View Solution
Knowledge Check
Wavelength of light used in an optical instrument are λ1=400Å and λ2=5000Å, then ratio of their respective resolving power (corresponding to λ1 and λ2) is
A16:25B9:1C4:5D5:4Wavelength of light used in an optical instrument are λ1=4500 Å and λ2=6000 Å. What is the ratio of the resolving powers corresponding to λ1andλ2?
A34B43C916D169Wavelength of light used in an optical instrument are λ1=4000Å and λ2=5000Å then ratio of their respective resolving resolving powers (corresponding to λ1 and λ2) is
A16:25B9:1C4:5D5:4
If light of 6000Å is coming from a star, what will be resolving power of a telescope, if diameter of objective is 100 inch ?
View SolutionLight of wavelength 5,000Å is used in a microscope of numerical aperture 0.10. Calculate the resovlving power of the microscope.
View SolutionLight of wavelength 5,000Å is falling on a microscope objective lens with a cone angle of 60∘. Calculate the resolving power of the microscope.
View SolutionIn a YDSE experiment, d = 1mm, λ= 6000Å and D= 1m. The minimum distance between two points on screen having 75% intensity of the maximum intensity will be
View SolutionBy using light of wavelength 5200 Å, two points separated by a distance of 0.12 mm can just be resolved by a microscope. What will be the limit of resolution, if light of wavelength 6500 Å is used?
View Solution