A double slit of separation 0.1 mm is illuminated by white light. A colored interference pattern is formed on a screen 100 cm away. If a pin hole is located in this screen at a distance of 2 mm from the central fringe, the wavelength in the visible spectrum (4000 Å to 7000Å) which will be absent in the light transmitted through the pin hole is (are)
4000 Å
5000Å
6000Å
7000Å
The correct Answer is:A
To solve the problem, we need to determine the wavelengths of light that will be absent in the light transmitted through the pinhole located at a distance of 2 mm from the central fringe of the interference pattern formed by the double slit.
1. Identify Given Values:
- Slit separation (d) = 0.1 mm = 0.1×10−3 m
- Distance from slits to screen (D) = 100 cm = 1 m
- Distance from central fringe to pinhole (y) = 2 mm = 2×10−3 m
2. Understand the Condition for Minima:
The condition for minima in a double-slit interference pattern is given by:
Δx=y⋅dD
where Δx is the path difference, and for minima, it is given by:
Δx=(2n−1)λ2
where n is the order of the minimum.
3. Set Up the Equation:
Equating the two expressions for path difference:
y⋅dD=(2n−1)λ2
4. Rearranging for Wavelength (λ):
Rearranging the equation gives:
λ=2y⋅dD(2n−1)
5. Substituting Known Values:
Substitute y, d, and D into the equation:
λ=2⋅(2×10−3)⋅(0.1×10−3)1⋅(2n−1)
Simplifying this gives:
λ=4×10−6⋅10−4(2n−1)=4×10−10(2n−1) m
6. Convert to Angstroms:
Since 1 m=1010 Å, we convert λ to Angstroms:
λ=4×10−10×1010(2n−1)=4000(2n−1) Å
7. Finding Wavelengths in the Visible Spectrum:
We need to find values of n such that λ falls within the visible spectrum range (4000 Å to 7000 Å):
- For n=1:
λ=40001=4000 Å
- For n=2:
λ=40003≈1333.33 Å(Not in visible range)
- For n=3:
λ=40005=800 Å(Not in visible range)
- For n=4:
λ=40007≈571.43 Å(Not in visible range)
- For n=5:
λ=40009≈444.44 Å(Not in visible range)
- For n=6:
λ=400011≈363.64 Å(Not in visible range)
8. Conclusion:
The only wavelength that will be absent in the light transmitted through the pinhole is:
λ=4000 Å
- Slit separation (d) = 0.1 mm = 0.1×10−3 m
- Distance from slits to screen (D) = 100 cm = 1 m
- Distance from central fringe to pinhole (y) = 2 mm = 2×10−3 m
The condition for minima in a double-slit interference pattern is given by:
Δx=y⋅dD
where Δx is the path difference, and for minima, it is given by:
Δx=(2n−1)λ2
where n is the order of the minimum.
Equating the two expressions for path difference:
y⋅dD=(2n−1)λ2
Rearranging the equation gives:
λ=2y⋅dD(2n−1)
Substitute y, d, and D into the equation:
λ=2⋅(2×10−3)⋅(0.1×10−3)1⋅(2n−1)
Simplifying this gives:
λ=4×10−6⋅10−4(2n−1)=4×10−10(2n−1) m
Since 1 m=1010 Å, we convert λ to Angstroms:
λ=4×10−10×1010(2n−1)=4000(2n−1) Å
We need to find values of n such that λ falls within the visible spectrum range (4000 Å to 7000 Å):
- For n=1:
λ=40001=4000 Å
- For n=2:
λ=40003≈1333.33 Å(Not in visible range)
- For n=3:
λ=40005=800 Å(Not in visible range)
- For n=4:
λ=40007≈571.43 Å(Not in visible range)
- For n=5:
λ=40009≈444.44 Å(Not in visible range)
- For n=6:
λ=400011≈363.64 Å(Not in visible range)
The only wavelength that will be absent in the light transmitted through the pinhole is:
λ=4000 Å
Topper's Solved these Questions
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEYCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - LEVEL 2 (single correct option)
1 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEYCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Level 2 More Than One Correct
6 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEYCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Subjective Questions
5 Videos
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - All Questions
120 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEYCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Medical entrance s gallery
59 Videos
Similar Questions
A double slit of separation 1.5 mm is illuminated by white light (between 4000 and 8000 Å). On a screen 120 cm away colored interference pattern is formed. If a pinhole is made on this screen at a distance of 3.0 mm from the central white fringe, some wavelengths will be absent in the transimitted light. Find the second longest wavelength (in Å) which will be absent in the transmitted light.
View SolutionA double slilt experiment is performed with sodium (yellow) light of wavelength 589.3 nm and the interference pattern is observed on a screen 100 cm away. The tenth bright fringe has its centre at a distance of 12 mm from the central maximum. Find the separation betwen the slits.
View Solution
Knowledge Check
A single slit of width 0.1mm is illuminated by parallel light of wavelength 6000Å, and diffraction bands are observed on a screen 40cm from the slit. The distance of third dark band from the central bright band is:
A7.2 mmB3.6 mmC2.4 mmD0.6 mmIn Young's double-slit experiment, the slit are 0.5 mm apart and the interference is observed on a screen at a distance of 100 cm from the slits, It is found that the ninth bright fringe is at a distance of 7.5 mm from the second dark fringe from the center of the fringe pattern. The wavelength of the light used is
A5000ÅB50007ÅC2500ÅD25007ÅIn Yonung's double-slit experiment, two slits which are separated by 1.2 mm are illuminated with a monochromatic light of wavelength 6000Å The interference pattern is observed on a screen placed at a distance of 1 m from the slits. Find the number of bright fringes formed over 1 cm width on the screen.
A25B12C15D20
The two slits in Young's double slit experiment are separated by a distance of 0.03 mm. An interference pattern is produced on a screen 1.5 m away. The 4th bright fringe is at a distance of 1 cm from the central maximum. Calculate the wavelength of light used.
View SolutionThe two slits in Young's double slit experiment are separated by a distance of 0.03 mm. An interference pattern is produced on a screen 1.5 m away. The 3rd bright fringe is at a distance of 1 cm from the central maximum. Calculate the wavelength of light used.
View SolutionIn YDSE, the slits are separated by 0.5mm and screen is placed 1.0m away. It is found that the ninth bright fringe is at a distance of 8.835mm from the second dark fringe. Find the wavelength of light used.
View SolutionIn Young's experiment the slits, separated by d=0.8mm, are illuminated with light of wavelength 7200 Å, Interference pattern is obtained on a screen D=2m from the slits. Find minimum distance from central maximum at which the a average intensity is 50% if of maximum?
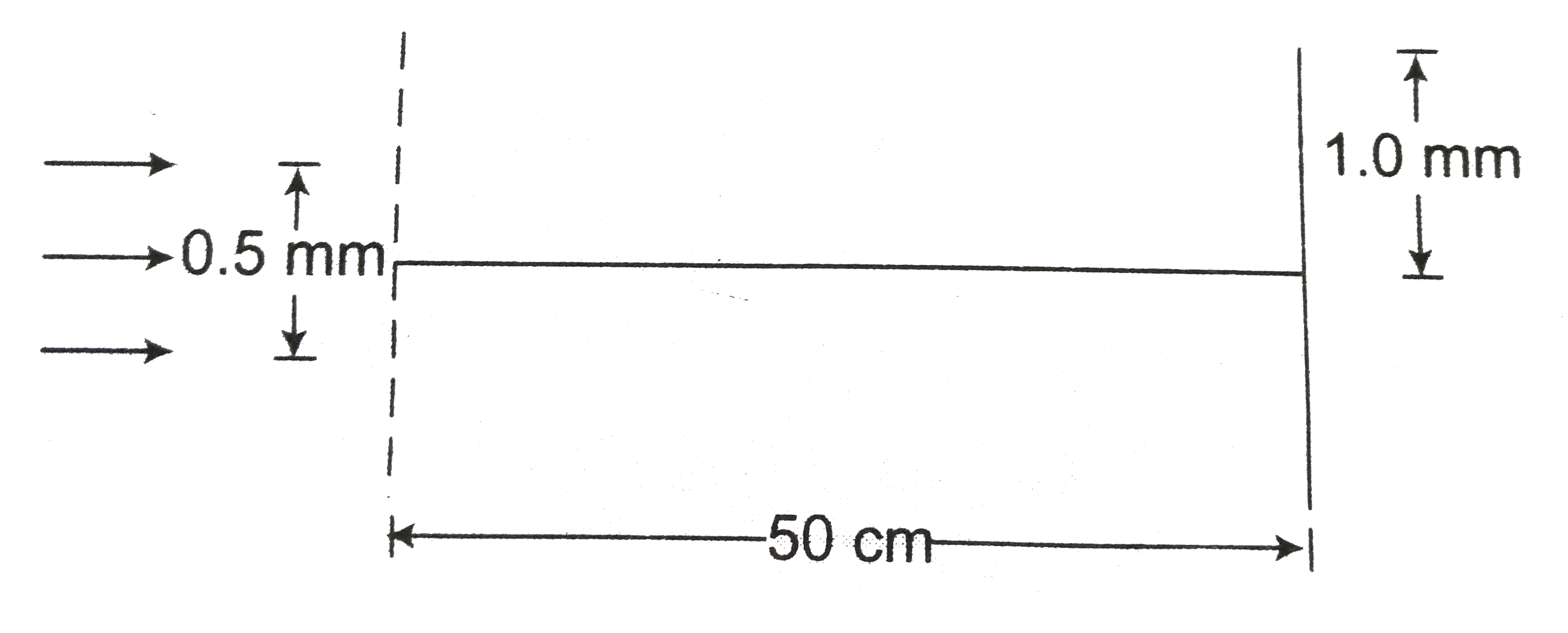
View SolutionWhite coherent light (400nm−700nm) is sent through the slits of a YDSE. D=0.5mm, D=50 cm. There is a hole in the screen at a point 1.0mm away (along the width of the fringes) from the central line.
(a) Which wavelength will be absent in the light coming from the hole?
(b) Which wavelength(s) will have a strong intensity?
View Solution
