Two plane mirrors AB and AC are inclined at an angle θ=20∘. A ray of light starting from point P is incident at point Q on the mirror AB, then at R on mirror AC and again on S on AB. Finally the ray ST goes parallel to mirror AC. The angle which the ray makes with the normal at point Q on mirror AB is
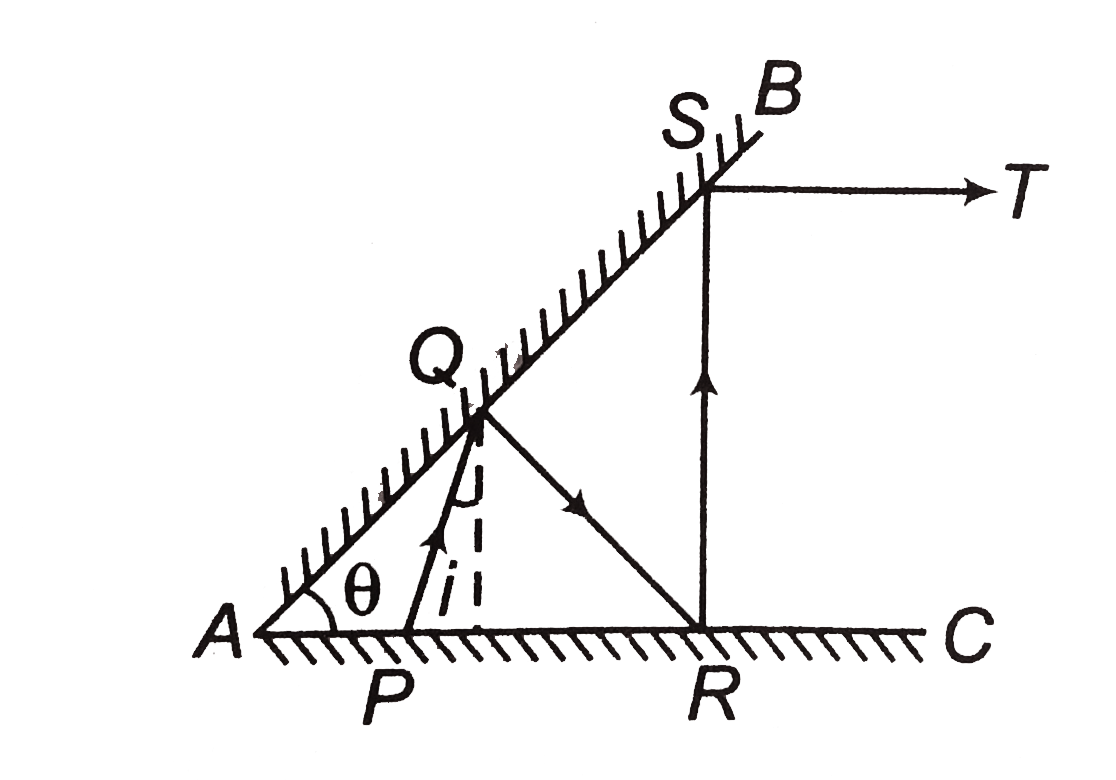
20∘
30∘
40∘
60∘
The correct Answer is:B
90∘−i(θ)+2r=180∘
∴r−(90∘+i−θ2)
p=180∘−(90∘−r)−2i
=180∘−90∘+(90∘+i+θ2)−2i
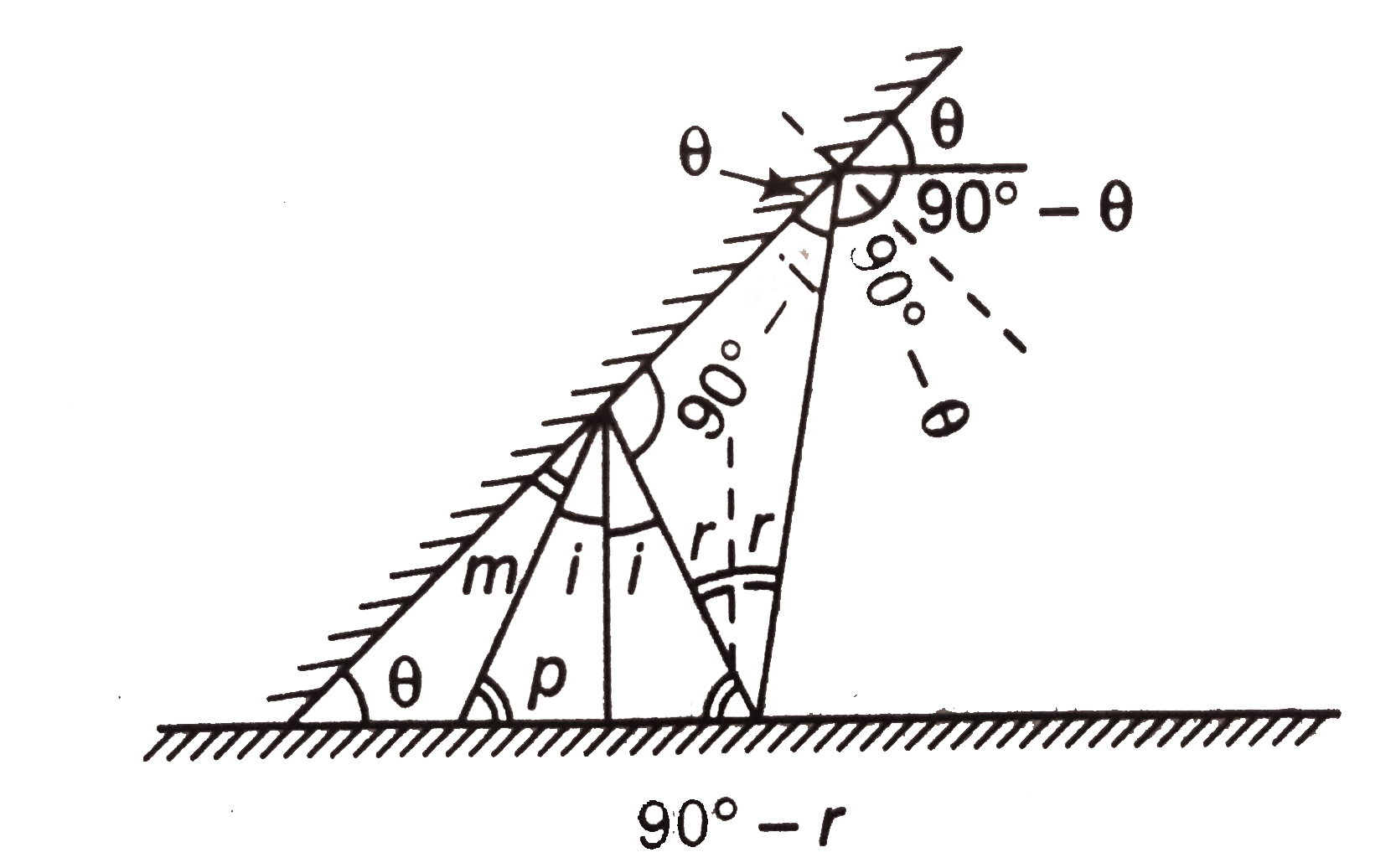
90∘−r
=180∘−4i+90∘+i−θ2
=270∘−3i−θ2
m=p−(θ)=180∘−(90∘−i)−2i
∴(270∘−3i−(θ)2−θ=90∘−i
Substituting (θ=20∘, we get
i=30∘.
Topper's Solved these Questions
- REFLECTION OF LIGHTBOOK - DC PANDEYCHAPTER - REFLECTION OF LIGHTEXERCISE - Level 2 More Than One Correct
6 Videos
- REFLECTION OF LIGHTBOOK - DC PANDEYCHAPTER - REFLECTION OF LIGHTEXERCISE - Level 2 Comprehensive Based
4 Videos
- REFLECTION OF LIGHTBOOK - DC PANDEYCHAPTER - REFLECTION OF LIGHTEXERCISE - Exercise Level 1 Subjective
17 Videos
- RAY OPTICSBOOK - DC PANDEYCHAPTER - RAY OPTICSEXERCISE - Medical entrance gallary
76 Videos
- REFRACTION OF LIGHTBOOK - DC PANDEYCHAPTER - REFRACTION OF LIGHTEXERCISE - Level 2 Subjective
21 Videos
Similar Questions
Two plane mirrors are inclined to each other at an angle θ. A ray of light is reflected at one
mirror and then at the other. Find the total deviation of the ray.
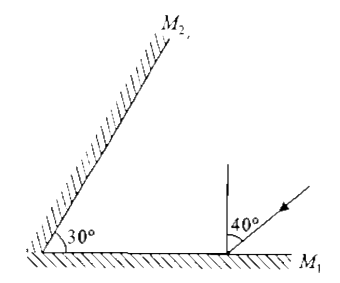
View SolutionTwo plane mirrors are inclined to each other at an angle 30∘ to each other. A ray of light is incident at an angle of 40∘ to the mirror (M1). Find the total angle of deviation of the ray after the third successive due to mirrors.
View Solution
Knowledge Check
The angle which the incident ray makes with the mirror is called
Aangle of incidenceBangle of reflectionCright angleDglancing angle of incidenceTwo plane mirror are inclined to each other at 90∘. A ray of light is incident on one mirror. The ray will undergo a total deviation of
A180∘B90∘C45∘Ddata insufficientTwo plane mirros are inclined to each other at an angle 60∘ if a ray of light incident on firs tmirros paralell to the second mirror, it is reflected formt the second mirror
APerpendicular to the first mirrorBParallel to the first mirrorCParallel to the second mirrorDPerpenicualr to the second mirror
Two plane mirrors are inclined at angleθ.A ray of light is incident on one mirror at an angle of incidence.i.The rayis refelected from this mirror,falls on the second mirror from where it is refleced parallel to the first mirror .What is the value of i,the angle of incident in term of θ?
View SolutionA ray of light is incident at an angle of 35∘ on a plane mirror. What is angle θ ?

View SolutionTwo mirrors are inclined at angle θ as shown in figure. Light rays are incident parallel to one of mirrors. Light will start retracing its path after the reflection if
View SolutionTwo plane mirrors are inclined to each other such that a ray of light incident on the first mirror and parallel to the second is reflected from the second mirror parallel to the first mirror. Determine the angle between the two mirrors:
View SolutionTwo plane mirrors are inclined at an angle θ to one another A ray of light incident on the first mirror and parallel ot the second mirror is reflected from the second mirror parallel to the first mirror ,
What is the value of θ
View Solution
