The figure show four situations in which charged particles are at equal distances from the origin. If E1,E2,E3andE4 be the magnitude of the net electic field at the origin in four situations i, ii, iii and iv respectively then
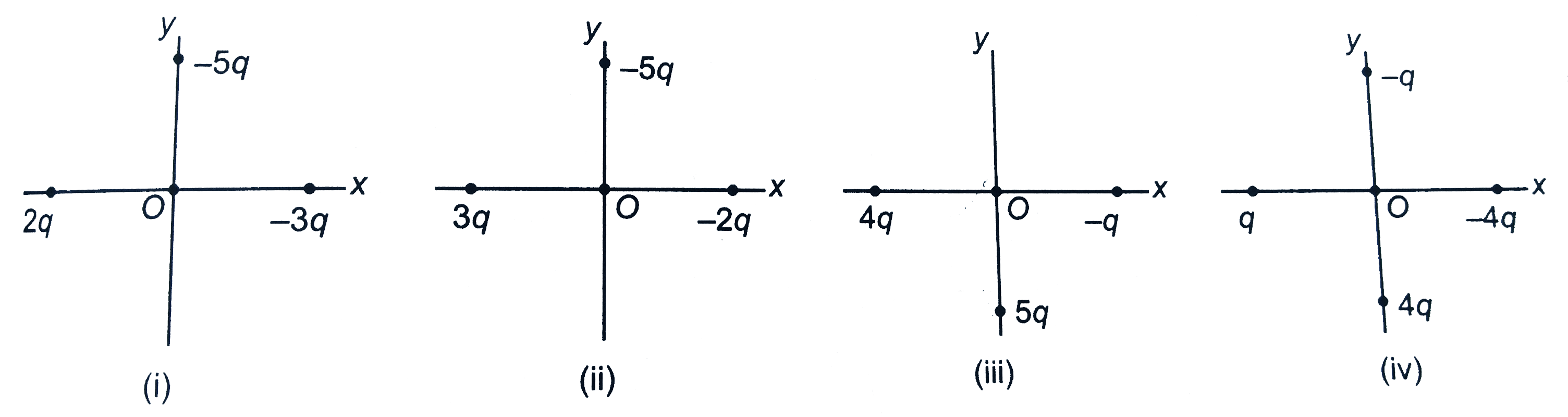
E1=E2=E3=E+4
E1=E2>E3>E4
E1<E2<E3=E4
E1>E2=E3>E4
The correct Answer is:A
Let E=magnitude of electric field at origin due to change ±q. Then
i.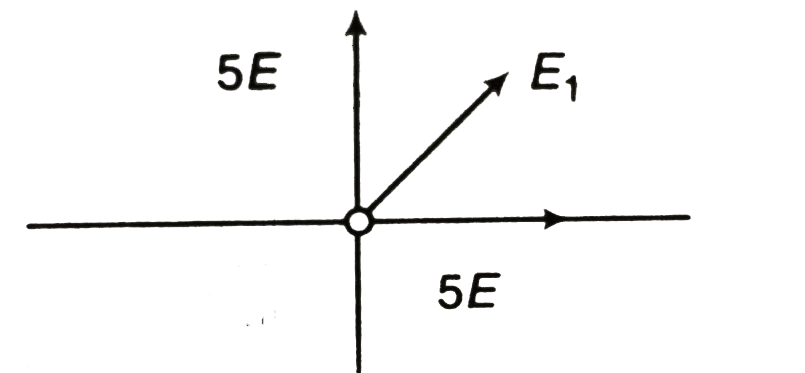 
E1=√(5E)2+(5E)2=5√2E
ii.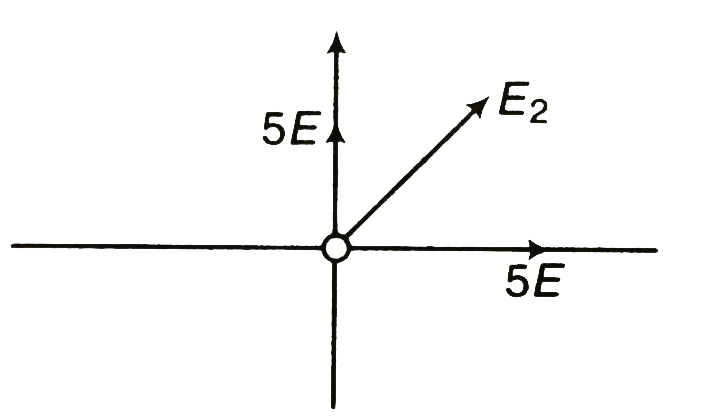 
E2 is again 5√2E.
Similarly, we can find E3 and E−4 also
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.1
4 Videos
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.2
9 Videos
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Example Type 8
3 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEYCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - All Questions
120 Videos
Similar Questions
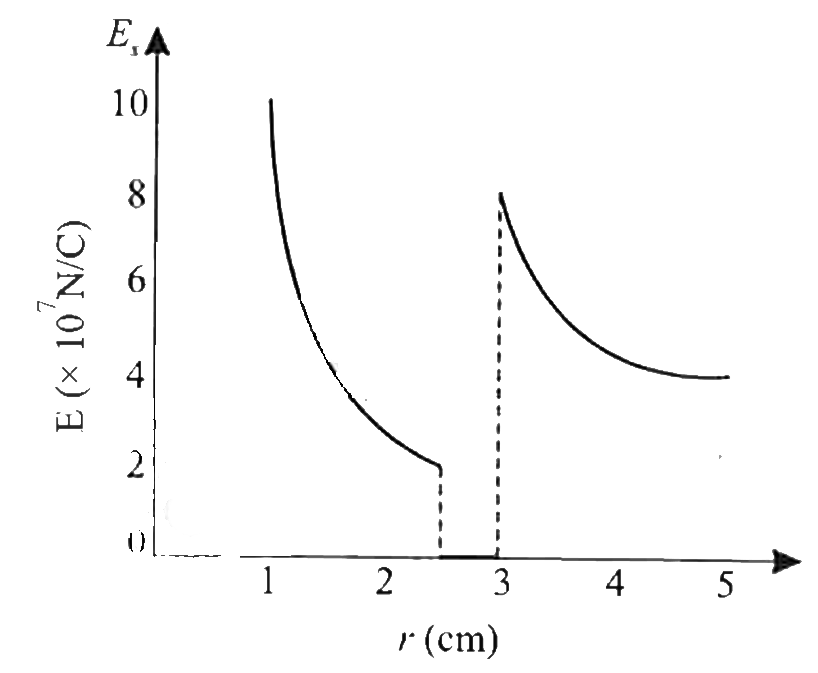
A charged particle is held a the centre of a spherical shell. The following figure gives the magnitude E of the electric field versus radial distance r. what is the net charge on the shell ?
View SolutionAll the particles are situated at a distance R from the origin. The distance of centre of mass of the body from the origin will be
(1) More than R
(2) Less than R
(3) Equal to R
(4) At the origin
View Solution
Knowledge Check
In the following four situations charged particles are equal distance from the origin. Arrange them the magnitude of the net electric field at origin greatest first
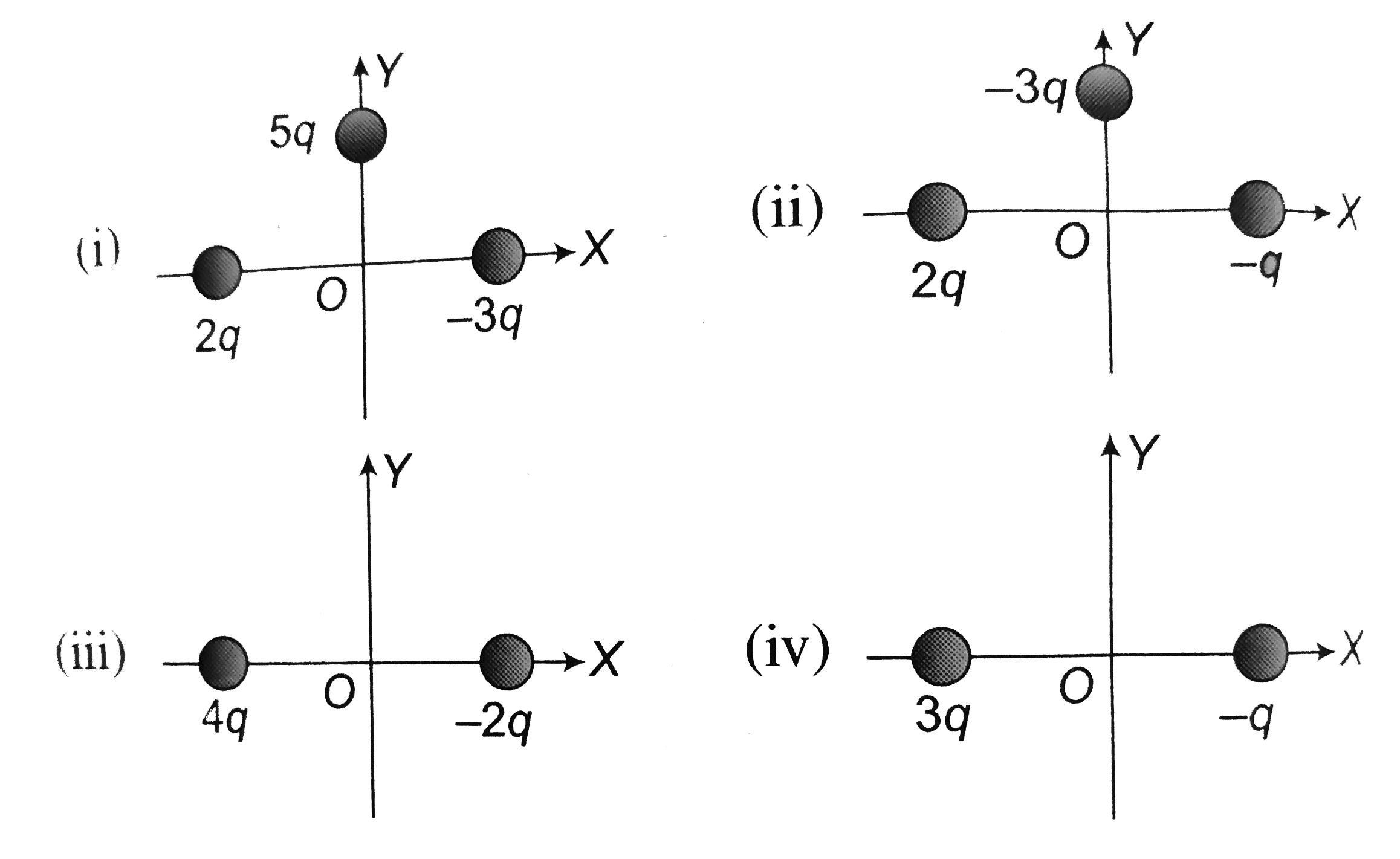A(i)>(ii)>(iii)>(iv)B(ii)>(i)>(iii)>(iv)C(i)>(iii)>(ii)>(iv)D(iv)>(iii)>(ii)>(i)The figure shows two large, closely placed, parallel, nonconducting sheets with identical (positive) uniform surface charge densities, and a sphere with a uniform (positive) volume charge density. Four points marked as 1,2,3, and 4 are shown in the space in between. If E1,E2,E3,andE4 are magnitude of net electric fields at these points, respectively, then
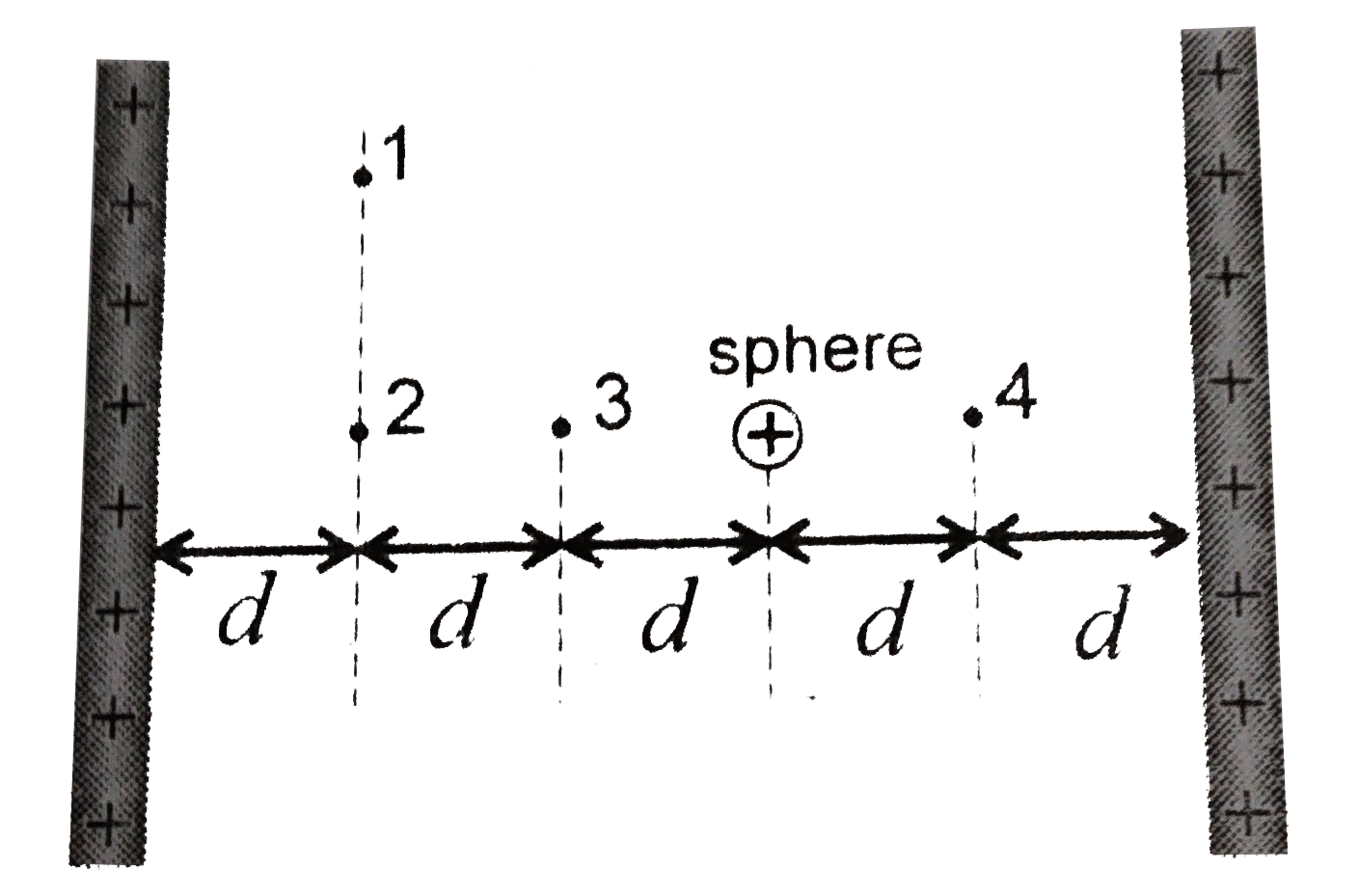AE1>E2>E3>E4BE1>E2>E3=E4CE3=E4>E2>E1DE1=E2=E3=E4The figure shows two large, closely parallel, nonconducting sheets with identical (positive) uniform surface charge densities and a sphere with a uniform (positive) volume charge density. Four points marked as 1,2,3 and 4 are shown in the space in between. If E1,E2,E3 and E4 are magnitude of net electric fields at these points respectively then :
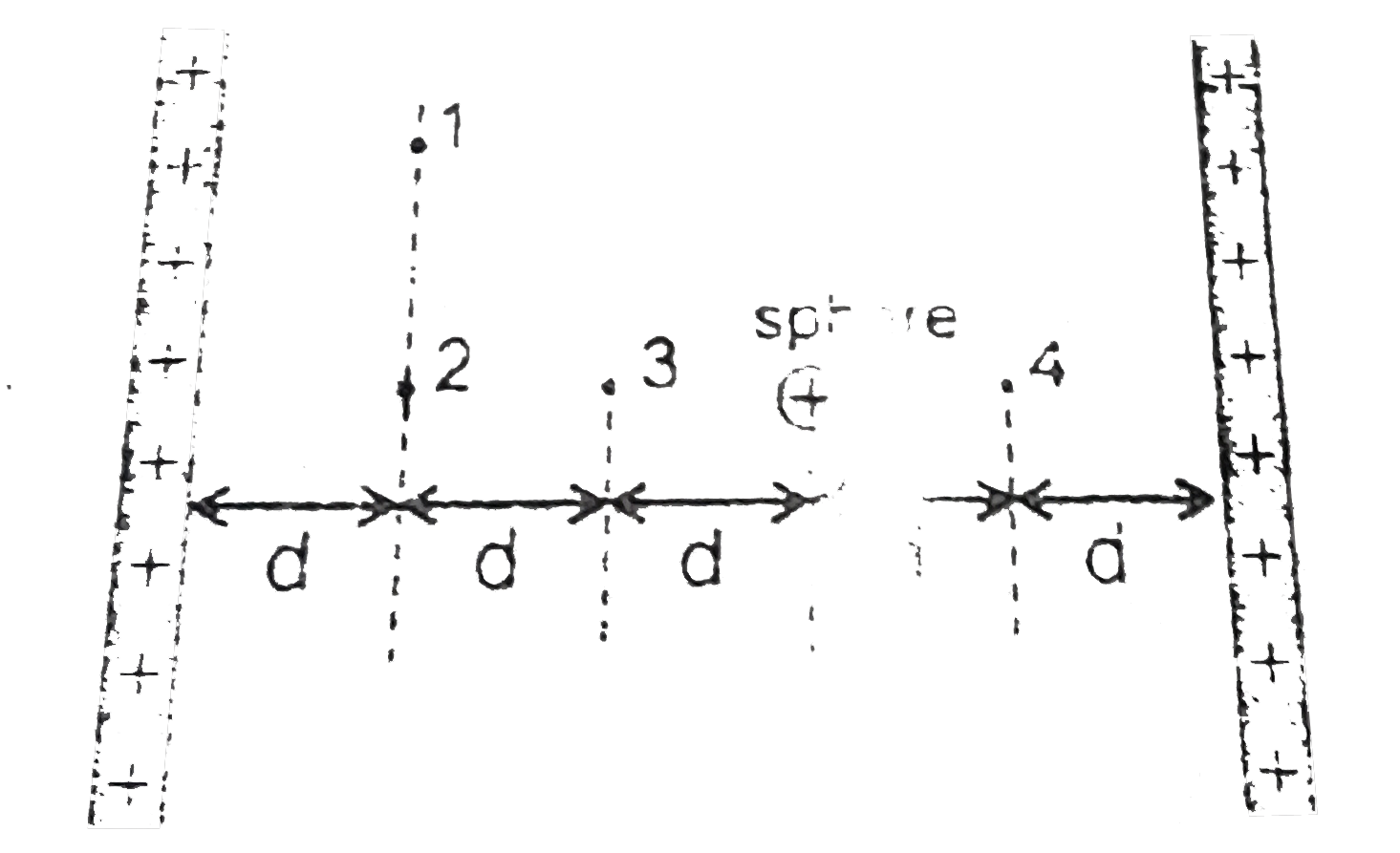AE1>E2>E3>E4BE1>E2>E3=E4CE3=E4>E2>E1DE1=E2=E3=E4
In Fig., the four particles fixed in palce and have charges q1=q2=+5e,q3=+3e and q4=−1e. Distance d=8.0μm. What is the magnitude of the net electric field at point P due to the particles ?
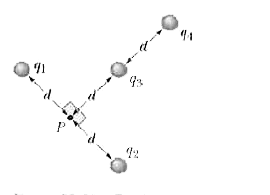
View SolutionConsider the situation shown In Figure 2.3.Are the two distances of the particle from the particle from the origin the same ?
View SolutionCharge is uniformly distributed with density ρ in a cylindrical region of radius R and infinite length. The magnitude of electric field at distance R2 and 2R from the axis of the cylinder is E1 and E2 respectively. The ratio E1E2 is equal to ___________.
View SolutionFigure, shown above, shows three situations involving a charged particle and a uniformly charged spherical shell. The charges and radii of the shells are indicated in the figure. If F1,F2 and F3 are the magnitudes of the force on the particle due to the shell in situations (I),(II) and (III) then
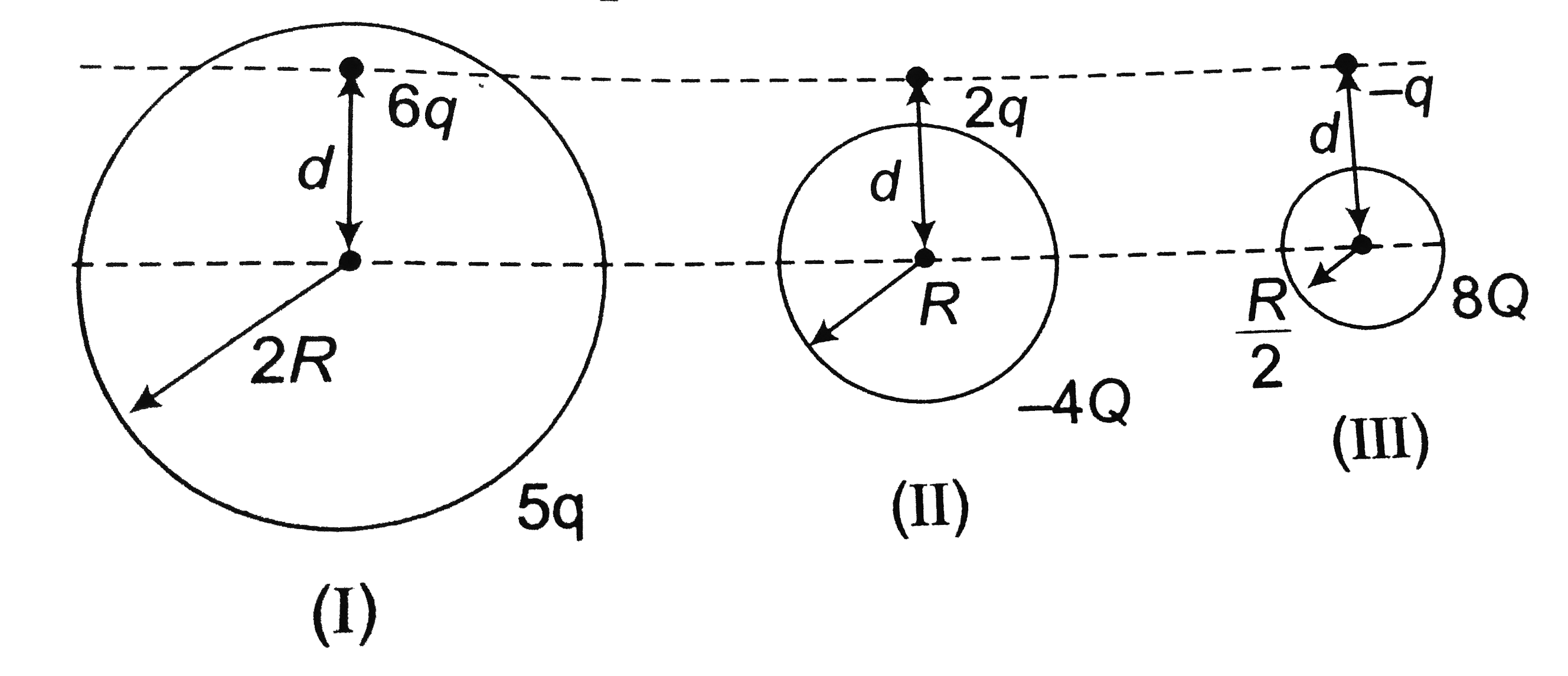
View SolutionA figure below shows the forces that three charged particles exert on each other. Which of the four situations shown can be correct .
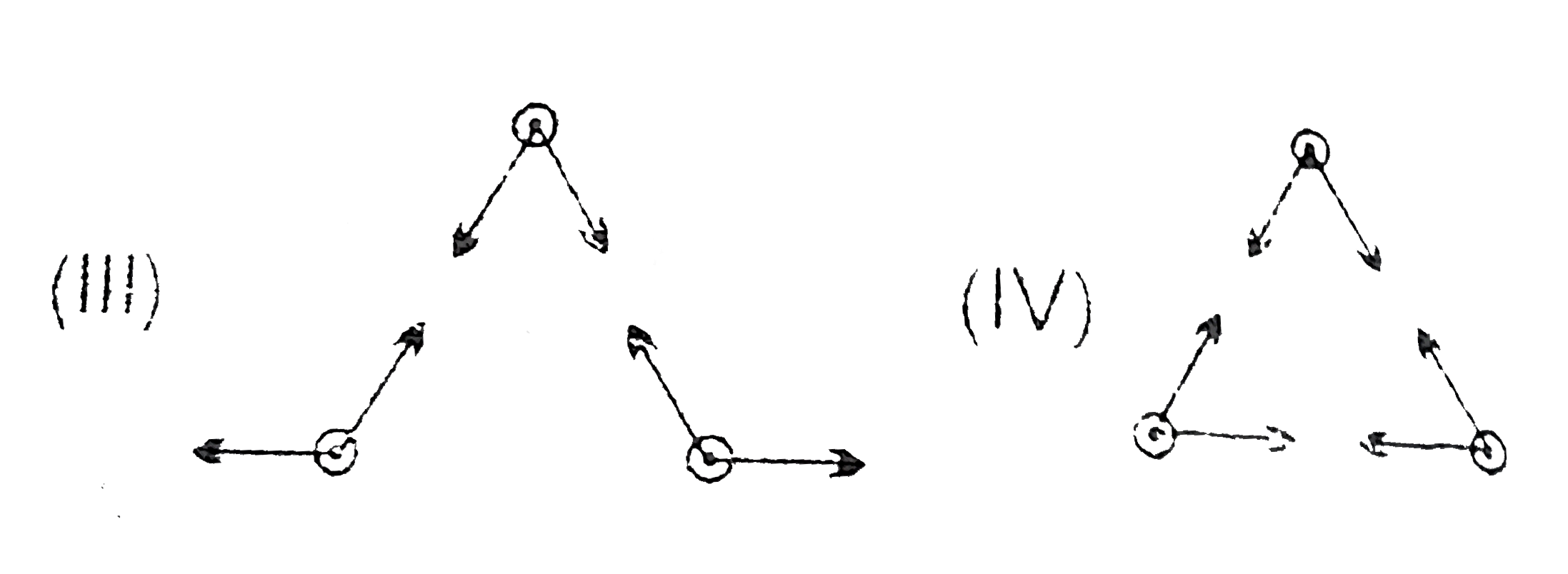
View Solution