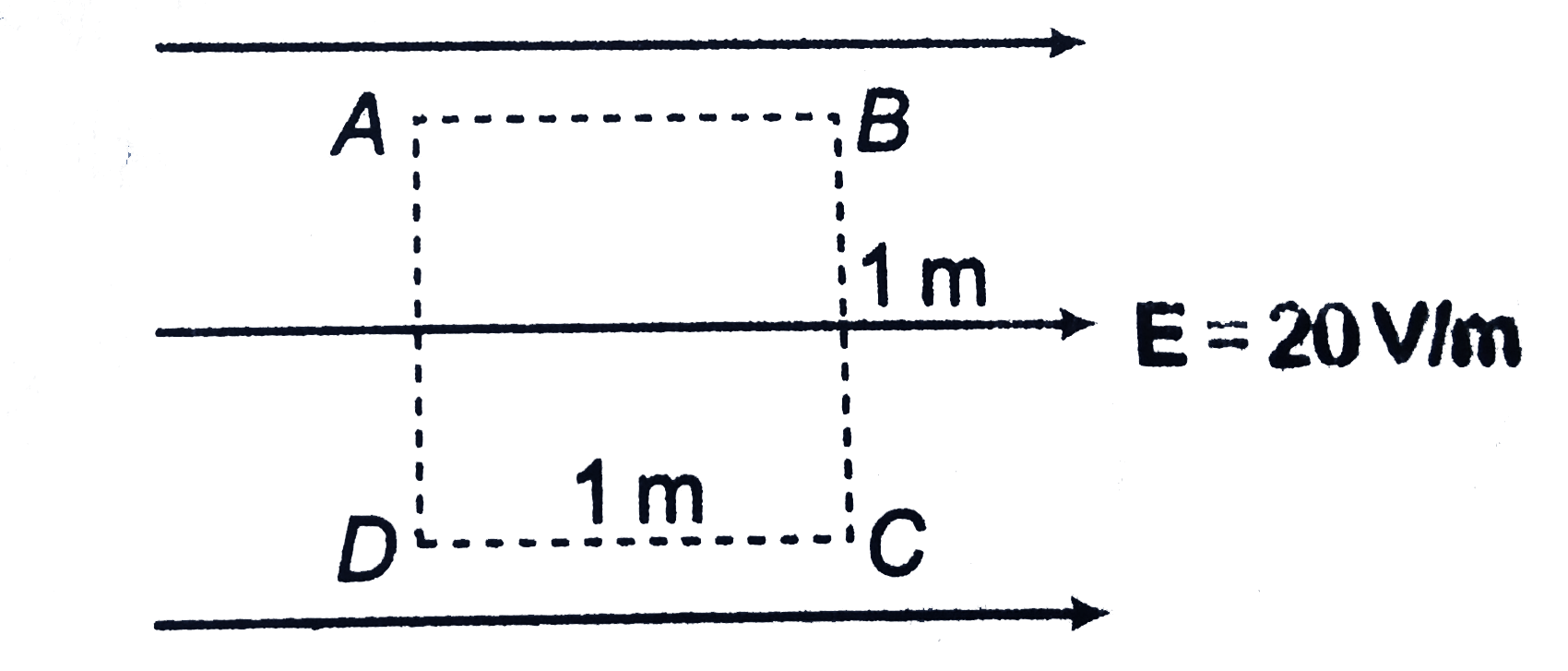
In uniform electric field E=10N/C, find

a. VA−VB , b. VB−VC
−10V and 20V
10V and −10V
30V and 20V
−10V and 40V
The correct Answer is:A
a.VB>VA, So, VA−VB will be negative.
Further dAB=2cos60∘=1m
∴VA−VB=−EdAB=(−10)(1)=−10volt
b. VB>VC,soVB−VC will be positive
Further, dBC=2.0m
∴VB−VC=(10)(2)=20volt
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Example Type 5
5 Videos
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Example Type 6
4 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEYCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - All Questions
120 Videos
Similar Questions
Knowledge Check
In uniform electric field E=10N/C, find

a. VA−VB , b. VB−VCA−10V and 20VB10V and −10VC30V and 20VD−10V and 40VVA−VB will be
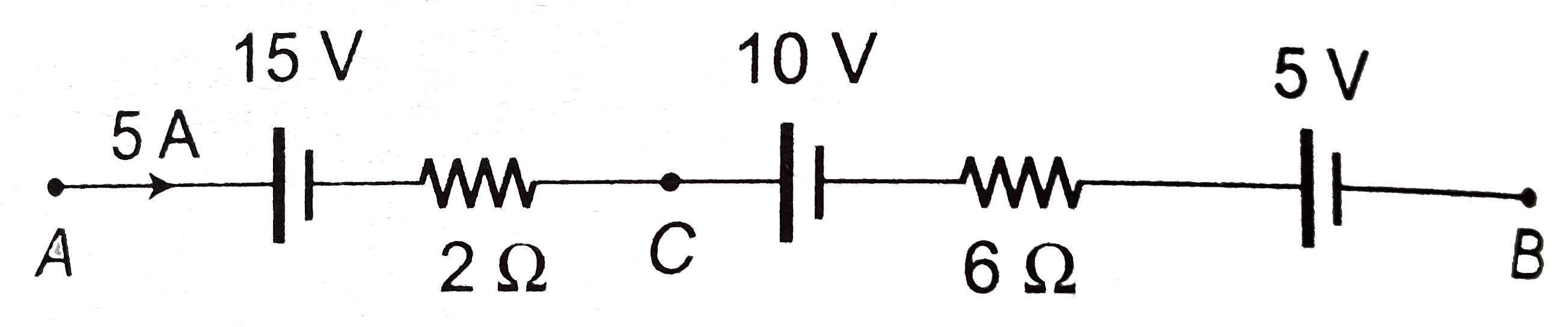 .A20 VB40 VC70 VD100 V
.A20 VB40 VC70 VD100 VA uniform electric field E0 exists in a region at angle 45∘ with the x - axis. There are twom point A(a,0)andB(0,b) having potential VAandVB, respectively, then
 .Aa<bBa>bCa>bDa=b
.Aa<bBa>bCa>bDa=b
A uniform electric field E exists in a region of space. Refer to the given figure for the location of three points A, B and C.
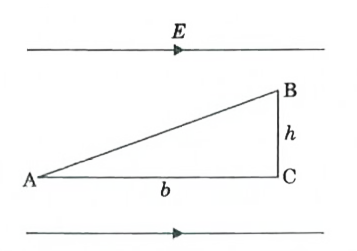
Find: (a)VB−VA (b)VC−VA
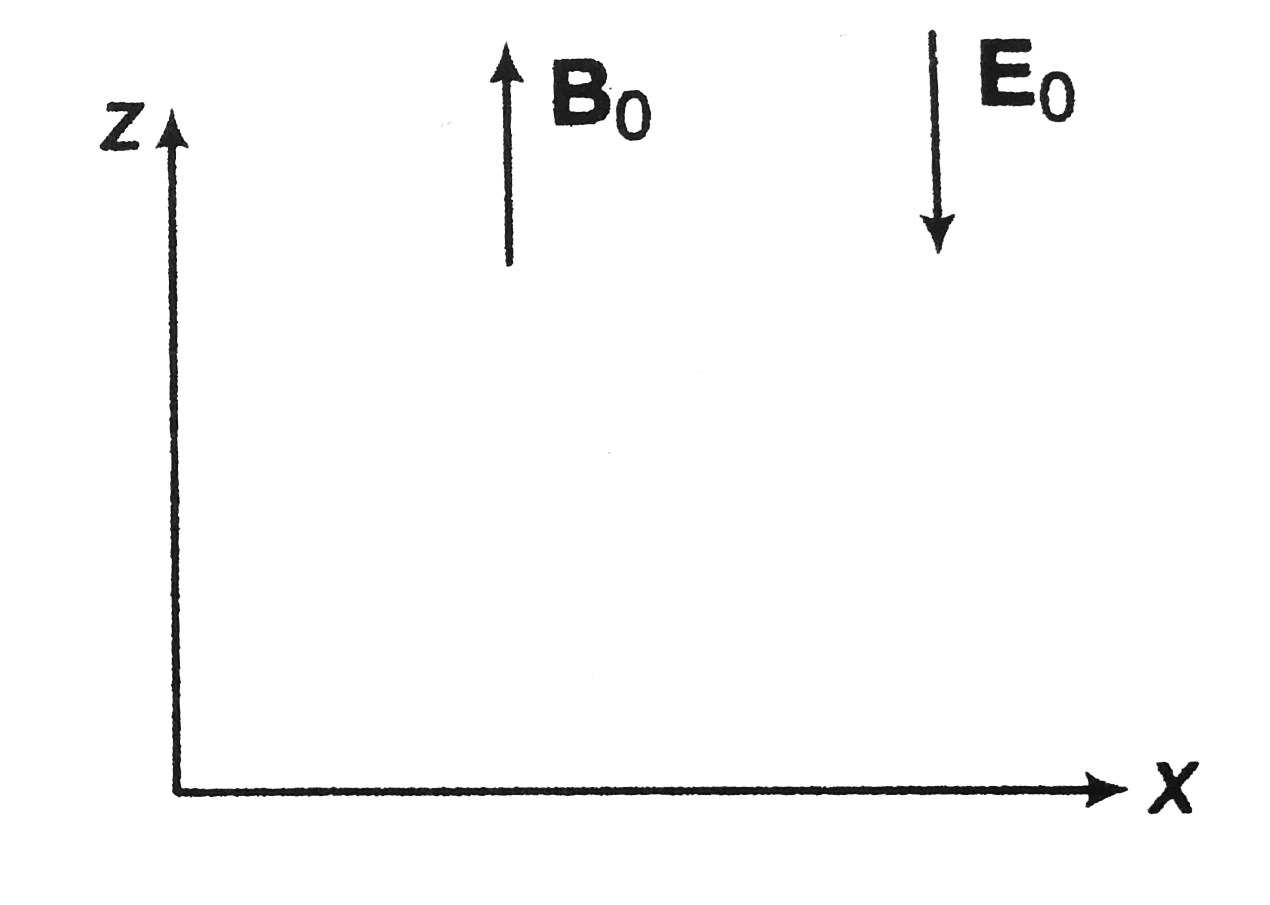
View SolutionIn a certain region, uniform electric field E=−E0ˆk and magnetic field B=B0ˆk are present. At time t=0, a particle of mass in and charge q is given a velocity v=v−0ˆj+v−0ˆk. Find the minimum speed of the particle and the time when it happens so.
View SolutionTwo points A and B are 2 cm apart and a uniform electric field E acts along the straight line AB directed A to B with E=200N/C. A particle of charge +10−6C is taken from A to B along AB, Calculate
a. the force on the charge
b. the potential difference VA−VB and
c.the work done on the charge by E
View SolutionTwo point charge qA and qB are placed in a uniform external electric field →E at point A and B respectively. The position vector of A is →rA and that of B is →rB. if V(→rA) and V(→rB) be the electric potentials at point A and B due to the external electric field →E, then potential energy of the system will be
View SolutionAssertion : A conducting equilateral loop abc is moved translationally with constant speed v in uniform inward magnetic field B as shown. Then : Va−Vb=Vb−Vc
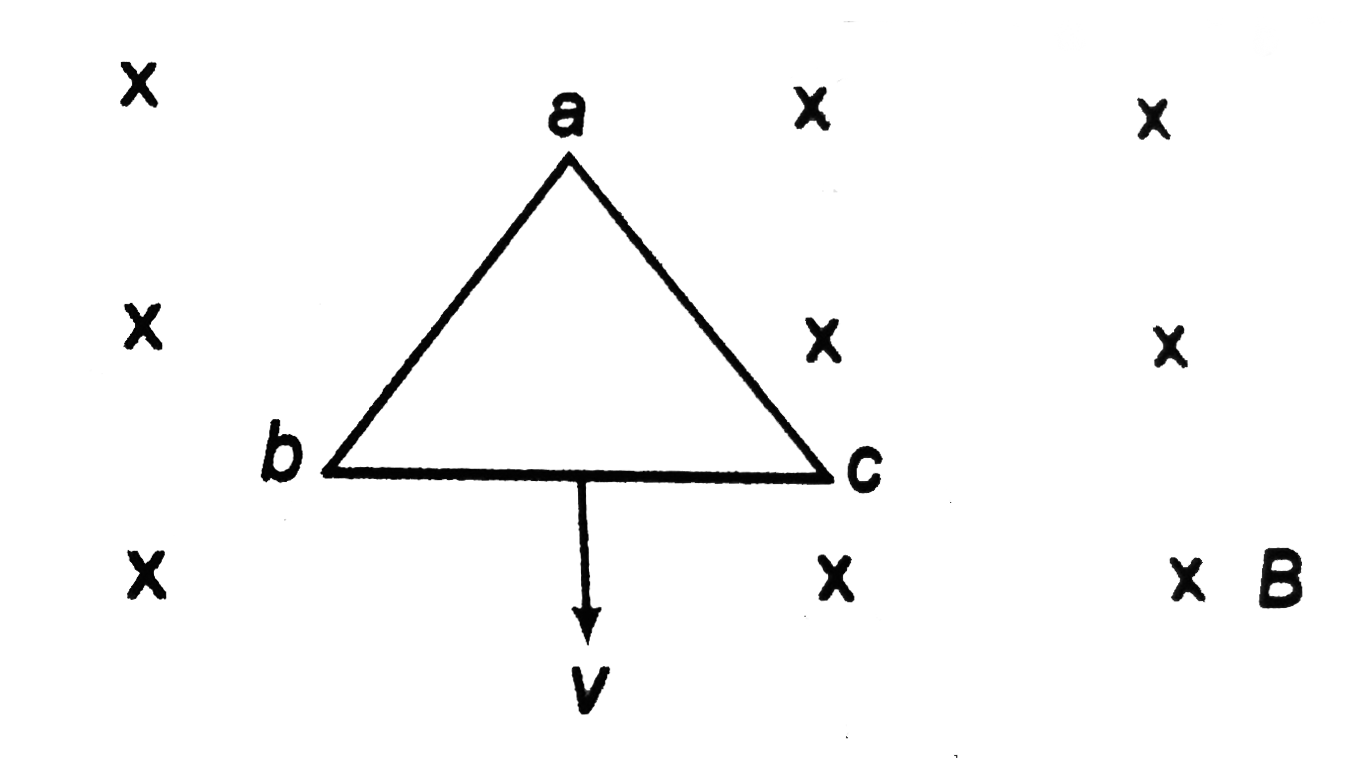
Reason : Point a is at higher potential than point b.
View Solution

 .
.