The electric potential at point A is 20V and at B is −40V. Find the work done by an external and electrostatic force in moving an electron slowly from B to A.
The correct Answer is:A, B
To solve the problem of finding the work done by an external force and the electrostatic force in moving an electron from point B to point A, we can follow these steps:
1. Identify the Charge of the Electron:
The charge of an electron (Q₀) is given as:
Q₀=−1.6×10−19 C
2. Identify the Electric Potentials:
The electric potential at point A (V_A) and point B (V_B) are given as:
VA=20 V
VB=−40 V
3. Calculate the Work Done by the External Force:
The work done (W_ext) by the external force in moving the charge from B to A can be calculated using the formula:
Wext=Q₀×(VA−VB)
Substituting the values:
Wext=−1.6×10−19×(20−(−40))
=−1.6×10−19×(20+40)
=−1.6×10−19×60
=−9.6×10−18 J
4. Calculate the Work Done by the Electrostatic Force:
The work done by the electrostatic force (W_elec) is equal to the negative of the work done by the external force:
Welec=−Wext
Substituting the value we found:
Welec=−(−9.6×10−18)=9.6×10−18 J
Final Results:
- Work done by the external force:
Wext=−9.6×10−18 J
- Work done by the electrostatic force:
Welec=9.6×10−18 J
The charge of an electron (Q₀) is given as:
Q₀=−1.6×10−19 C
The electric potential at point A (V_A) and point B (V_B) are given as:
VA=20 V
VB=−40 V
The work done (W_ext) by the external force in moving the charge from B to A can be calculated using the formula:
Wext=Q₀×(VA−VB)
Substituting the values:
Wext=−1.6×10−19×(20−(−40))
=−1.6×10−19×(20+40)
=−1.6×10−19×60
=−9.6×10−18 J
The work done by the electrostatic force (W_elec) is equal to the negative of the work done by the external force:
Welec=−Wext
Substituting the value we found:
Welec=−(−9.6×10−18)=9.6×10−18 J
- Work done by the external force:
Wext=−9.6×10−18 J
- Work done by the electrostatic force:
Welec=9.6×10−18 J
Topper's Solved these Questions
- ELECTROSTATICSBook:DC PANDEYChapter:ELECTROSTATICSExercise:Example Type 5
Explore 5 Videos
- ELECTROSTATICSBook:DC PANDEYChapter:ELECTROSTATICSExercise:Example Type 6
Explore 4 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBook:DC PANDEYChapter:ELECTROSTATIC POTENTIAL AND CAPACITORSExercise:(C) Chapter exercises
Explore 50 Videos
- GRAVITATIONBook:DC PANDEYChapter:GRAVITATIONExercise:All Questions
Explore 120 Videos
Similar Questions
The potential at a point A is – 500 V and that at another point B is + 500 V. What is the work done by external agent to take 2 units (S.I.) of negative charge from B to A.
View SolutionThe electrical potential at point in an electric field is 6 V. Find the work done in bringing 12 C of charge from infinity to that point.
View Solution
Knowledge Check
The electric potential at. Point A is 20 V and B is – 20 . The work done by an external force in moving electron slowly from B to A is
A−6.4×10−18JB+6.4×10−18JC4×10−20JD−4×10−20JA charge Q is placed at the origin. The electric potential due to this charge at a given point in space is v. The work done by an external force in bringing another charge q from infinity up to the point is
AVqBVqCV+qDV
A spherical shell of radius r with a uniform charge q has point charge q0 at its centre. Find the work performed by the electric forces during the shell expansion slowly from radius R to 2R. Also find out work done by external agent against electric forces.
View Solution1μC charge is shifted from A to B, and it is found that work done by an external force is 40μJ. In doing so against electrostatic forces, find potential difference VA−VB.
View SolutionA charge of 20μC produces an electric field. Two points are 10 cm and 5 cm from this charge. Find the values of potentials at these points and calculate work done to take an electron from one point to the other.
View SolutionExpress electric potential (V) in terms of work done (W).
View SolutionFigures (a) and (b) show the field lines of a positive and negative point charge respectively
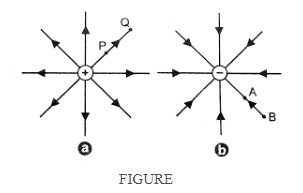
(a) Give the signs of the potential difference VP−VQ,VB–VA.
(b) Give the sign of the potential energy difference of a small negative charge between the points Q and P, A and B.
(c) Give the sign of the work done by the field in moving a small positive charge from Q to P.
(d) Give the sign of the work done by the external agency in moving a small negative charge from B to A.
(e) Does the kinetic energy of a small negative charge increase or decrease in going from B to A?
View Solution1μC charge is shifted from A to B and it is found that work done by external force is 80μJ against electrostic forces, find VA−VB
View Solution
