If resistance R1 in resistance box is 300Ω then the balanced length is found to be 75.0cm from end A. The diameter of unknown wire is 1 mm and length of the unknown wire is 31.4cm. Find the specific resistance of the unknown wire
To find the specific resistance (resistivity) of the unknown wire, we will follow these steps:
Step 1: Understand the given data
- Resistance in the resistance box, R1=300Ω
- Balanced length from end A, LA=75.0cm
- Length of the unknown wire, L=31.4cm=0.314m
- Diameter of the unknown wire, d=1mm=1×10−3m
Step 2: Calculate the length from end B
Since the total length of the meter bridge is 100 cm, the length from end B is:
LB=100cm−LA=100cm−75.0cm=25.0cm
Step 3: Use the balance condition to find the unknown resistance X
According to the meter bridge principle, we have:
R1X=LALB
Substituting the known values:
300ΩX=75.0cm25.0cm
This simplifies to:
300X=3
Cross-multiplying gives:
X=3003=100Ω
Step 4: Calculate the area A of the wire
The area A of the wire can be calculated using the formula for the area of a circle:
A=πd24
Substituting the diameter:
A=π(1×10−3)24=π(1×10−6)4=π4×10−6m2
Step 5: Calculate the specific resistance ρ
Using the formula for specific resistance:
ρ=R⋅AL
Substituting the values:
ρ=100Ω⋅(π4×10−6m2)0.314m
Calculating this gives:
ρ=100⋅3.14×10−640.314
ρ=100⋅0.785×10−60.314=78.5×10−60.314≈2.5×10−4Ωm
Final Answer
The specific resistance of the unknown wire is approximately:
ρ≈2.5×10−4Ωm
- Resistance in the resistance box, R1=300Ω
- Balanced length from end A, LA=75.0cm
- Length of the unknown wire, L=31.4cm=0.314m
- Diameter of the unknown wire, d=1mm=1×10−3m
Since the total length of the meter bridge is 100 cm, the length from end B is:
LB=100cm−LA=100cm−75.0cm=25.0cm
According to the meter bridge principle, we have:
R1X=LALB
Substituting the known values:
300ΩX=75.0cm25.0cm
This simplifies to:
300X=3
Cross-multiplying gives:
X=3003=100Ω
The area A of the wire can be calculated using the formula for the area of a circle:
A=πd24
Substituting the diameter:
A=π(1×10−3)24=π(1×10−6)4=π4×10−6m2
Using the formula for specific resistance:
ρ=R⋅AL
Substituting the values:
ρ=100Ω⋅(π4×10−6m2)0.314m
Calculating this gives:
ρ=100⋅3.14×10−640.314
ρ=100⋅0.785×10−60.314=78.5×10−60.314≈2.5×10−4Ωm
The specific resistance of the unknown wire is approximately:
ρ≈2.5×10−4Ωm
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - DC PANDEYCHAPTER - CURRENT ELECTRICITYEXERCISE - Example Type 1
2 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEYCHAPTER - CURRENT ELECTRICITYEXERCISE - Example Type 2
1 Video
- COMMUNICATION SYSTEMBOOK - DC PANDEYCHAPTER - COMMUNICATION SYSTEMEXERCISE - Subjective
11 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrances gallery
25 Videos
Similar Questions
If resistanceR1 in resistance box is 300Ω, then the balanced length is found to be 75.0cm from end A.The diameter of known wire is 1mm and length of the unknown wire is 31.4cm. Find the specific resistance of the unknown wire.
View SolutionWith an unknown resistance X in the left gap and a resistance R in the right gap of a meter bridge, the neutral point is obtained at a distance of 75cm from the left end. The shift of null point if the unknown resistance is shunted by an equal resistance X and keeping same resistance in right gap is
View Solution
Knowledge Check
In meter bridge experiment if, resistance S in resistance box =300Ω then the balanced length is found to be 25.0cm from end A. The diameter of unknown wire is 1mm and length of unknown wire is 31.4cm. The specific resistivity of the wire should be
A2.5×10−4Ω−mB3.5×10−4Ω−mC4.5×10−4Ω−mDNone of theseIf resistance S in RB=300Ω, then the balanced length is found to be 25.0cm from end A. The diameter of unknown wire is 1mm and length of the unknown wire is 3.14cm. The specific resistivity of the wire should be
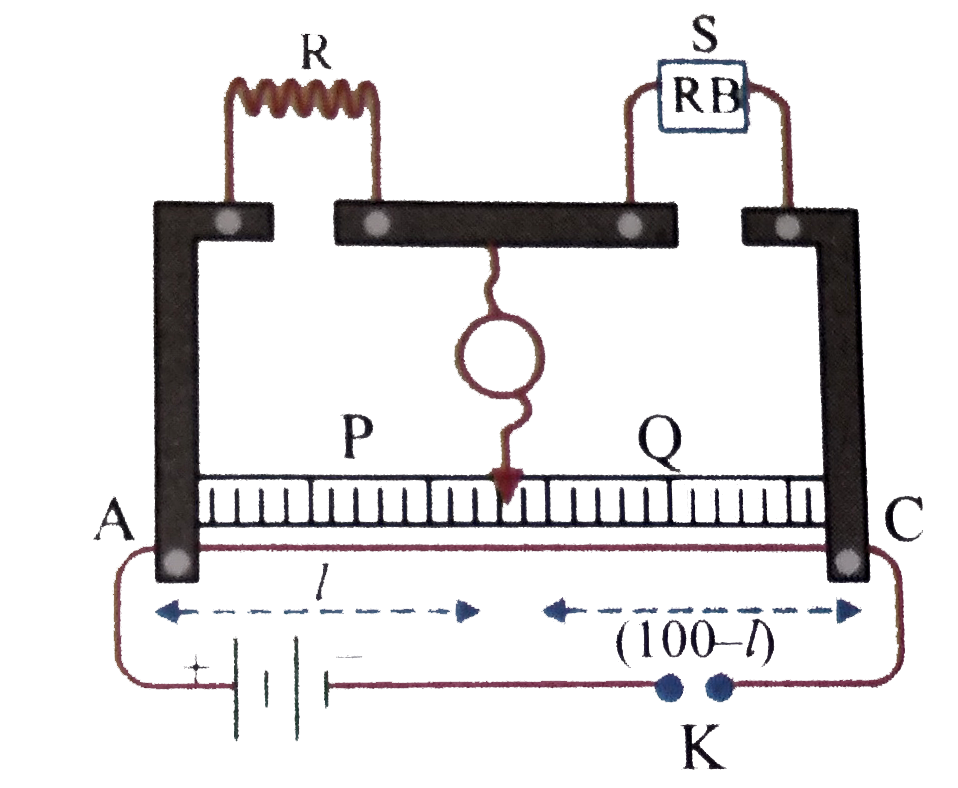 A2.5×10−4Ω−mB3.5×10−4Ω−mC4.5×10−4Ω−mD1.5×10−4Ω−m
A2.5×10−4Ω−mB3.5×10−4Ω−mC4.5×10−4Ω−mD1.5×10−4Ω−mIn meter bridge , the balancing length from left is found to be 20 cm when standard connected of 1Ω is in right gap . The value of unknown resistance is
A0.25ΩB0.4ΩC0.5ΩD4Ω
In a meter bridge when the resistance in the left gap is 4Ω and an unknown resistance in the right gap the balance point is obtained at 40cm from the zero end. On shunting the unknown resistance with 4Ω, find the shift of the balance point on the bridge wire
View SolutionIn a metre bridge when the resistance in the left gap is 2Ω and an unknown resistance in the right gap, the balance point is obtained at 40 cm from the zero end. On shunting the unknown resistance with 2Ω find the shift of the balance point on the bridge wire.
View SolutionThe resistance of a 50 cm long wire is 10Ω. The wire is stretched of uniform wire of length 100 cm. The resistance now will be
View SolutionIf the length of wire is (100.0 cm) and radius of wire as measured from screw gauge is (1.00mm) then the specific resistance of wire material is .
View SolutionIn a metere bridge, the balance length from left end (standard resistance of 1Ω is in the right gap) is found to be 20 cm, the length of resistance wire in left gap) is found to be 20 cm the length of resistance wire in left gap is 12 m and radius is 2mm its specific resistance is
View Solution
