A body of density ρ is dropped from reat from a height h into a lake of density σ(σ>ρ). The maximum depth the body sinks inside the liquid is (neglect viscous effect of liquid)
hρσ−ρ
hσσ−ρ
hρσ
hσρ
The correct Answer is:A
To solve the problem of finding the maximum depth a body of density ρ sinks into a lake of density σ after being dropped from a height h, we can follow these steps:
Step 1: Calculate the velocity of the body just before it hits the surface of the lake.
When the body is dropped from height h, it accelerates due to gravity. The velocity v just before it hits the lake can be calculated using the equation of motion:
v=√2gh
Step 2: Analyze the forces acting on the body when it is submerged.
Once the body enters the water, two main forces act on it:
- The buoyant force (upward) given by Fb=σVg, where V is the volume of the body.
- The weight of the body (downward) given by Fw=ρVg.
Step 3: Determine the net force and acceleration.
The net force F acting on the body when it is submerged is:
F=Fb−Fw=σVg−ρVg=Vg(σ−ρ)
Using Newton's second law, the acceleration a of the body can be expressed as:
ma=Vg(σ−ρ)
Since m=ρV, we can substitute this into the equation:
ρVa=Vg(σ−ρ)
Dividing both sides by V (assuming V≠0):
a=g(σ−ρ)ρ
Step 4: Use kinematic equations to find the maximum depth.
The body will continue to sink until its velocity becomes zero. We can use the kinematic equation:
v2=u2+2as
Where:
- v=0 (final velocity at maximum depth),
- u=√2gh (initial velocity just before entering the water),
- a=−g(σ−ρ)ρ (negative because it is deceleration),
- s=hmax (the maximum depth we want to find).
Substituting the values:
0=(2gh)−2(−g(σ−ρ)ρ)hmax
This simplifies to:
2gh=2g(σ−ρ)ρhmax
Step 5: Solve for hmax.
Cancelling 2g from both sides:
h=(σ−ρ)ρhmax
Rearranging gives:
hmax=ρhσ−ρ
Final Answer:
The maximum depth hmax that the body sinks into the lake is:
hmax=ρhσ−ρ
---
When the body is dropped from height h, it accelerates due to gravity. The velocity v just before it hits the lake can be calculated using the equation of motion:
v=√2gh
Once the body enters the water, two main forces act on it:
- The buoyant force (upward) given by Fb=σVg, where V is the volume of the body.
- The weight of the body (downward) given by Fw=ρVg.
The net force F acting on the body when it is submerged is:
F=Fb−Fw=σVg−ρVg=Vg(σ−ρ)
Using Newton's second law, the acceleration a of the body can be expressed as:
ma=Vg(σ−ρ)
Since m=ρV, we can substitute this into the equation:
ρVa=Vg(σ−ρ)
Dividing both sides by V (assuming V≠0):
a=g(σ−ρ)ρ
The body will continue to sink until its velocity becomes zero. We can use the kinematic equation:
v2=u2+2as
Where:
- v=0 (final velocity at maximum depth),
- u=√2gh (initial velocity just before entering the water),
- a=−g(σ−ρ)ρ (negative because it is deceleration),
- s=hmax (the maximum depth we want to find).
0=(2gh)−2(−g(σ−ρ)ρ)hmax
This simplifies to:
2gh=2g(σ−ρ)ρhmax
Cancelling 2g from both sides:
h=(σ−ρ)ρhmax
Rearranging gives:
hmax=ρhσ−ρ
The maximum depth hmax that the body sinks into the lake is:
hmax=ρhσ−ρ
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Level 2 More Than One Correct10videos
FLUID MECHANICS
- BOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Level 2 Comprehension Based7videos
FLUID MECHANICS
- BOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Subjective Question2videos
FLUID MECHANICS
- BOOK - DC PANDEYCHAPTER - EXPERIMENTSEXERCISE - Subjective15videos
EXPERIMENTS
- BOOK - DC PANDEYCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE2videos
GENERAL PHYSICS
Similar Questions
Knowledge Check
- Question 1 - Select One
A body of density ρ is dropped from rest from height h (from the surface of water) into a lake of density of water σ(σ>ρ). Neglecting all disspative effects, the acceleration of body while it is in the take is
Ag(σρ−1) upwardsBg(σρ−1) downwardsCg(σρ) upwardsDg(σρ) downwards - Question 2 - Select One
body of density ρ is dropped from height h into a liquid having density σ(σ>ρ) .If the body just touches the of the container, then the distance of fallen would be proprotional to (Neglect viscous forces)
Ahσ−ρBhσ+ρCh×(σ−ρ)Dhρσ−ρ - Question 3 - Select One or More
A small body of density ρ' is dropped from rest at a height h into a lake of density ρ, where ρ>ρ’. Which of the following statements is/are correct if all dissipative effects are neglected (neglect viscosity)?
AThe speed of the body just entering the lake is √2ghBThe body in the lake experiences upward acceleration equal to {(ρ/ρ')−1}gCThe maximum depth to which the body sinks in the lake is hρ'(ρ−ρ')DThe body does not come back to the surface of the lake.
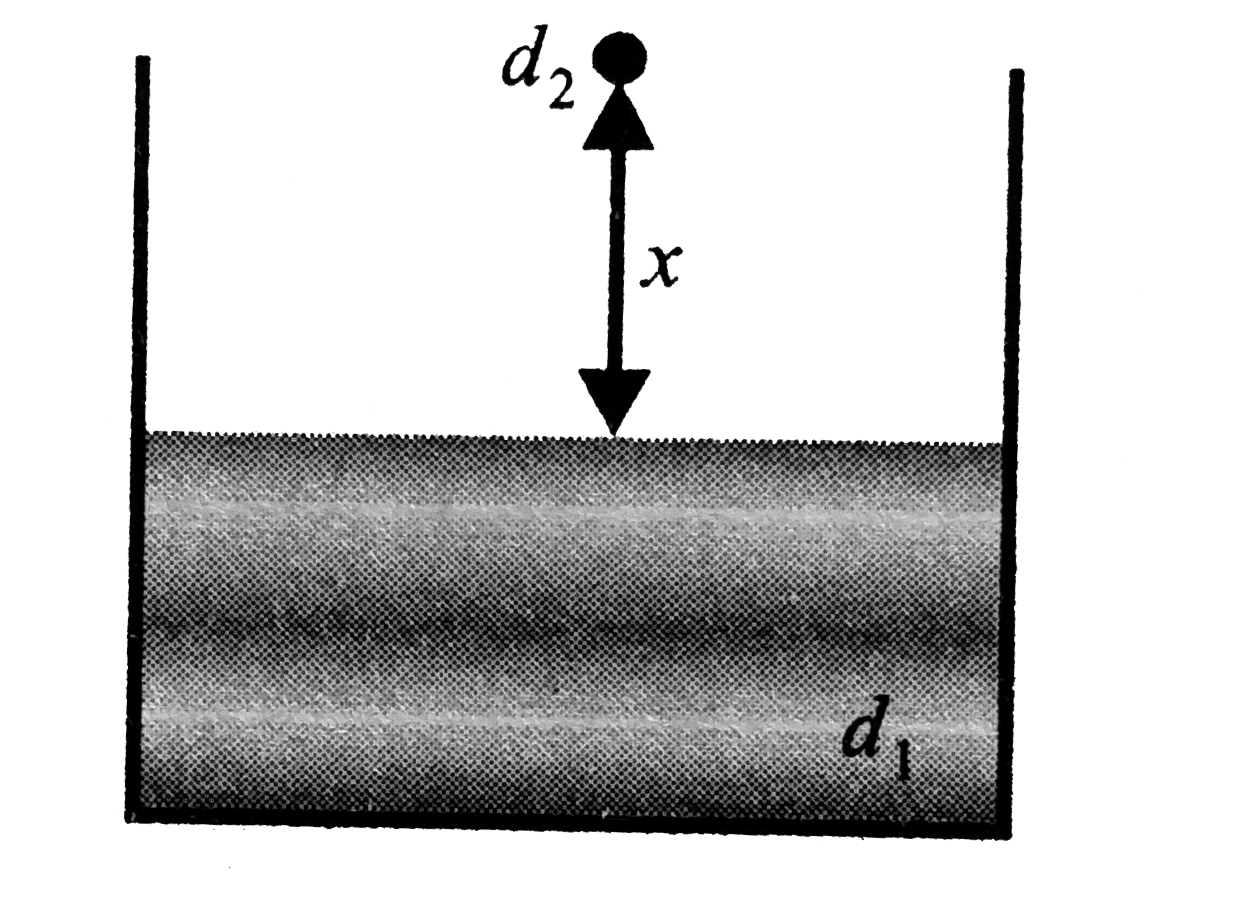
A body of density d2 is dropped from rest at a height x into a beaker having a liquid of density d1(d2>d1). Which of the following is/are true?
 View Solution
View SolutionA body of density ρ is dropped from reat from a height h into a lake of density σ(σ>ρ). The maximum depth the body sinks inside the liquid is (neglect viscous effect of liquid)
View SolutionA ball of density ρ is dropped from a height on the suraca of a non-visous liquid of dinsity 2ρ. Choose the correct options.
 View Solution
View SolutionAn object of density σ(<ρ) is dropped from rest at a height h into a liquid of density ρ kept in a tall vertical cylindrical container. Neglect all dissipative effects and assume that there is no spilling of the liquid.
The time taken by the object to reach the starting point (from which it is dropped) isView SolutionA ball of density ρ is released from deep inside of a liquid of density 2ρ. It will move up
View Solution

