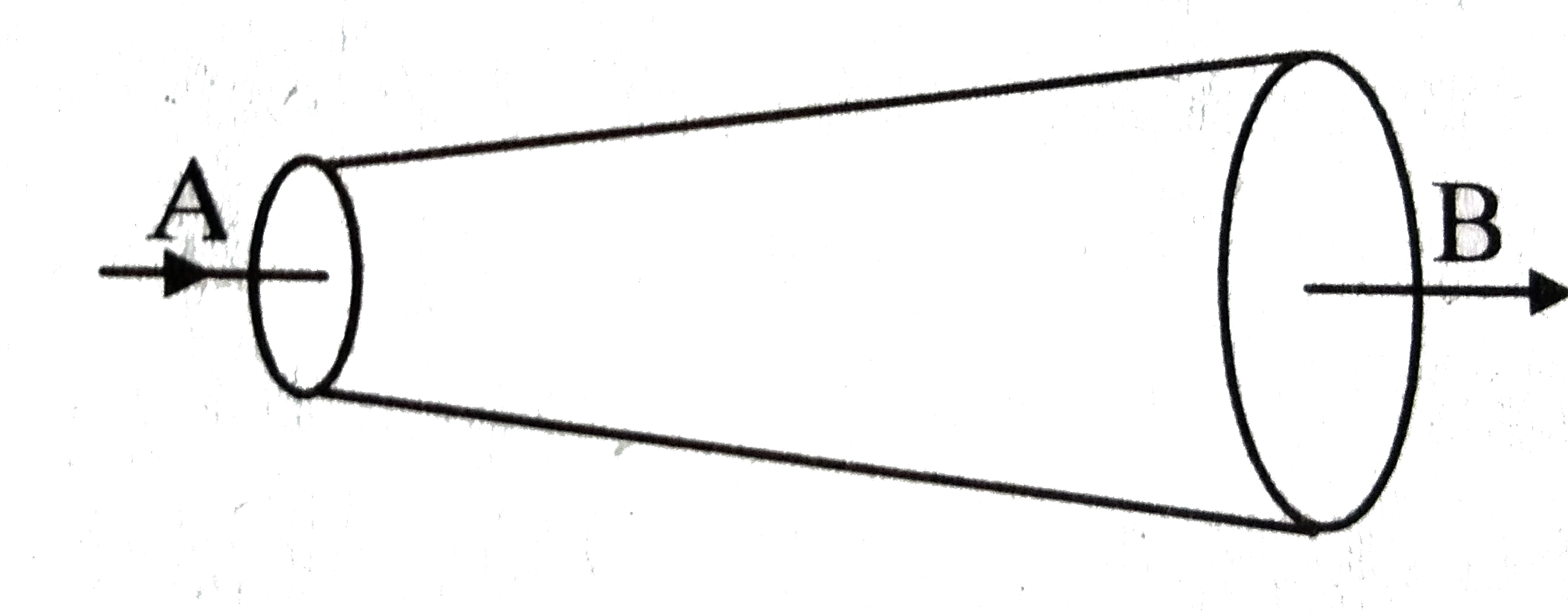
Assertion: An ideal fluid is flowing through a pipe. Speed of fluid particles is more at places where pressure is low.
Reason: Bernoulli's theoren can be derived from work-energy theorem.
If both Assertion and Reason are true and the Reason is correct explanation of the Assertion.
If both Assertion and Reason are true but Reason is not the correct explanation of Assertion.
If Assertion is true, but the Reason is false.
If Assertion is false but the Reason is true.
The correct Answer is:D
Speed will also depend on h.
Topper's Solved these Questions
- FLUID MECHANICSBOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Assertion and Reason
1 Videos
- FLUID MECHANICSBOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Level 1 Single Correct
49 Videos
- FLUID MECHANICSBOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Exercise16.8
4 Videos
- EXPERIMENTSBOOK - DC PANDEYCHAPTER - EXPERIMENTSEXERCISE - Subjective
15 Videos
- GENERAL PHYSICSBOOK - DC PANDEYCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
Knowledge Check
Assertion : When an ideal fluid flows through a pipe of non-uniform cross-section, then pressure is more at that section where area is more if the pipe is horizontal.
Reason : According to Bernoulli's theorem speed at broader cross-section will be less.AIf both Assertion and Reason are correct and Reason is the correct explanation of Assertion.BIf both Assertion and Reason are true but Reason is not the correct explanation of Assertion.CIf Assertion is true but Reason is false.DIf Assertion is false but Reason is true.Assertion: The velocity of flow of a liquid is smaller when pressure is larger and viceversa.
Reason: According to Bernoulli's theorem, for the stream line flow of an ideal liquid, the totla energy per unit mass remains constant.AIf both assertion and reason are true and reason is the correct explanation of assertionBIf both assertion and reason are true but reason is not the correct explanation of assertionCif assertion is true but reason is false.Dif both assertion and reason are false.Water is flowing through a horizontal pipe of non -uniform cross section. The speed of water is 30 cm/s at a place where pressure is 10 cm (of water). Calculate the speed of water at the other place where the pressure is half of that of the first place
A100.4 cm/sB101.4 cm/sC102.4 cm/sD103.4 cm/s
An ideal fluid flows through a pipe of circular cross-section made of two sections with diameters 2.5cm and 3.75cm. The ratio of the velocities in the two pipes is
View SolutionStoke's law states that the viscous drag force F experienced by a sphere of radius a, moving with a speed v through a fluid with coefficient of viscosity η, is given by F=6πη If this fluid is flowing through a cylindrical pipe of radius r, length l and a pressure difference of P across its two ends, then the volume of water V which flows through the pipe in time t can be written as
Vt=K(pl)aηbrc
where k is a dimensionless constant. Correct values of a, b and c are -
View SolutionAn ideal fluid flows through a pipe of circular cross section made of two section with diamters 2.5 cm and 9.76 cm. The ratio of the velocities in the two pipes is
View SolutionAn ideal fluid flows through a pipe of circular cross section made of two sections with diameters 2.5 cm and 3.75 cm. The ratio of the velocities in the two pipes is
View SolutionStoke’s law states that the viscous drag force F experienced by a sphere of radius a, moving with a speed V through a fluid with coefficient of viscosity η, is given by F=6πnav. If this fluid is flowing through a cylindrical pipe of radius r, length l and a pressure difference of P across its two ends, then the volume of water V which flows through the pipe in time t can be written as cVt=k(Pl)ηbrc , where k is a dimensional constant. Correct values of a, b and c are
View Solution