Find the period of oscillation of a pendulum of length l if its point of suspension is
(a) moving vertically up with acceleration a
(b) moving vertically down with acceleration a(<g)
(c) failing freely under gravity
moving horizontal with acceleration a.
The correct Answer is:A, B, C, D
(a) ge=g+a
(b) ge=g−a
( c) ge=0
(d) ge=√g2+a2
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEYCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 2 Single Correct
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEYCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 2 More Than One Correct
8 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEYCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 1 Single Correct
24 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEYCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEYCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
Similar Questions
Find the period of small oscillations of a mathematical pendulum of length l if its point of suspension O moves relative to the Earth's surface in an arbitrary directio with a constant acceleration w (figure). Calculate that period if l=21cm,w=g/2, and the angle between the vectors w and g equals beat=120∘.
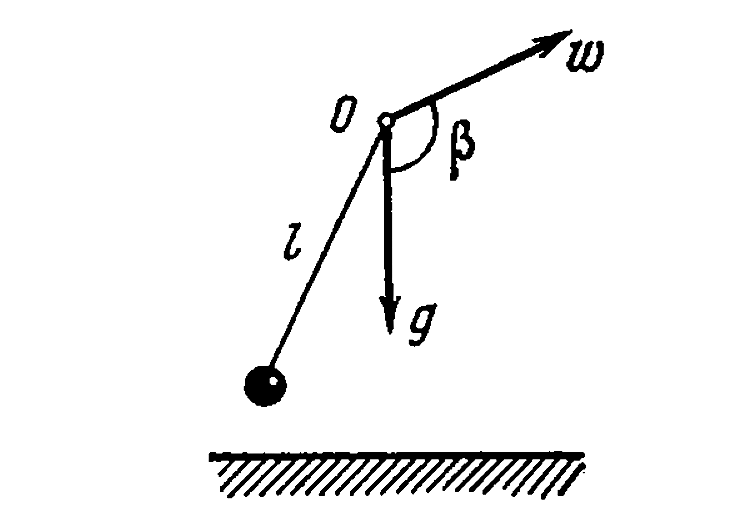
View SolutionHow will the frequency of small oscillation of a simple pendulum changes.
If it moves vertically upward with an acceleration 'a'
View Solution
Knowledge Check
An elevator is moving vertically up with a acceleration a the force exerted on the floor by a passenger of mass m is
AmgBmaCmg - maDmg + maIn an elevator moving vertically up with an acceleration g, the force exerted on the floor by a passanger of mass M is
AMgB12MgCzeroD2 MgThe period of oscillation of a simple pendulum at a given place with acceleration due to gravity 'g' depends on
Athe length of the pendulum 'l' onlyBboth 'l' and 'm' of the pendululmCmass of the bob of the pendulum onlyDl' and 'g'
How will the frequency of small oscillation of a simple pendulum changes.
If it moves vertically down with acceleration a<g or with acceleration a>g
View SolutionFind the period of oscillation of a simple pendulum of length L suspended from the roof of a vehicle which moves without friction down an inclined plane of inclination a.
View SolutionHow will the frequency of small oscillation of a simple pendulum changes.
a point of suspension moves horizontally in the plane of oscillation with acceleration a.
View SolutionFrom the ceiling of a train, a pendulum of length 'l' is suspended. The train is moving with an acceleration a0 on horizontal surface. What must be the period of oscillation of pendulum?
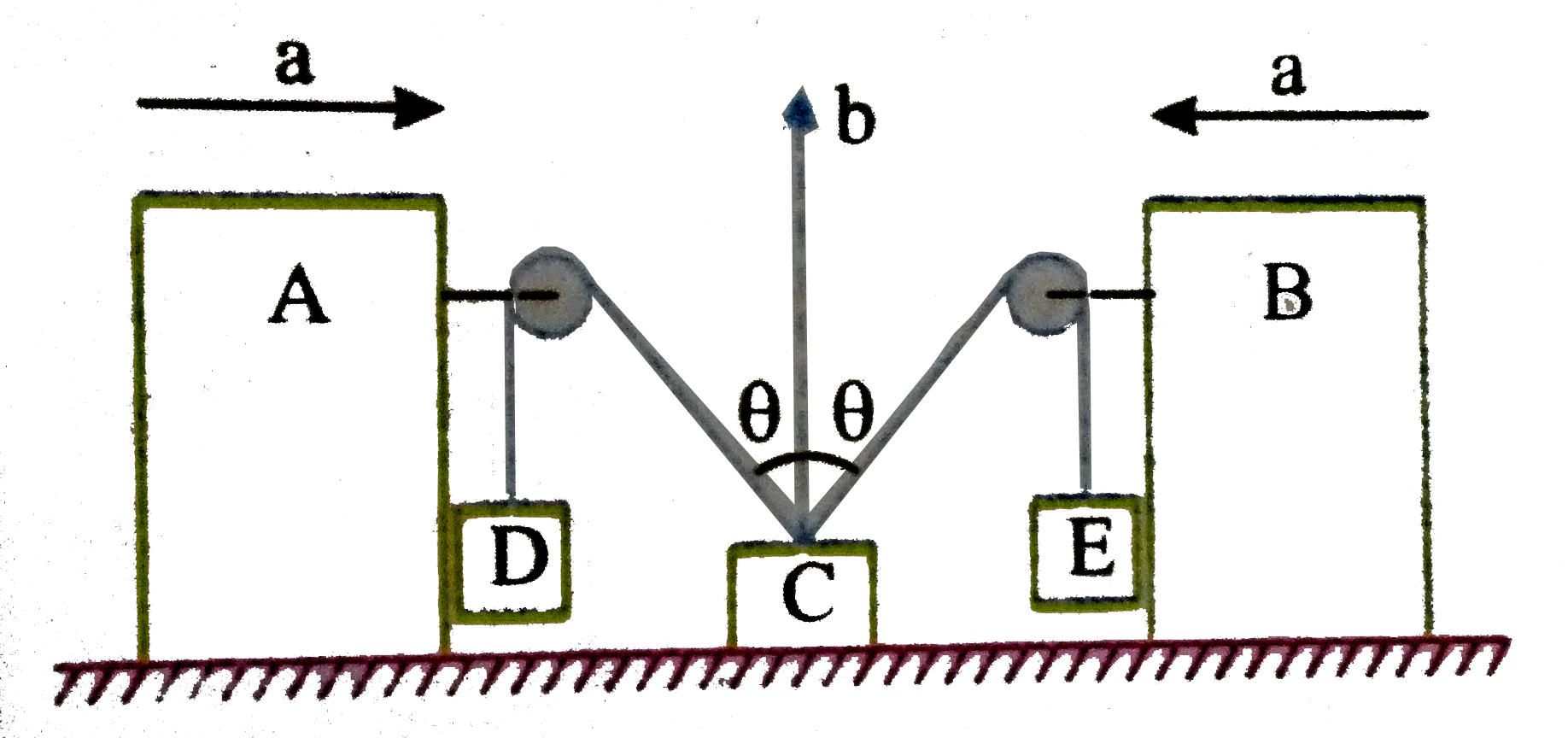
View SolutionIf A and B moves with acceleration a block c moves up with acceleration b calculate acceleration of D with respective A.
 .
.
View Solution
