Question

Identify the correct variation of potential energy $U$ as a function of displacement $x$ from mean position (or $x^2$) of a harmonic oscillator ($U$ at mean position $=0$)

Options

A.
B.
C.
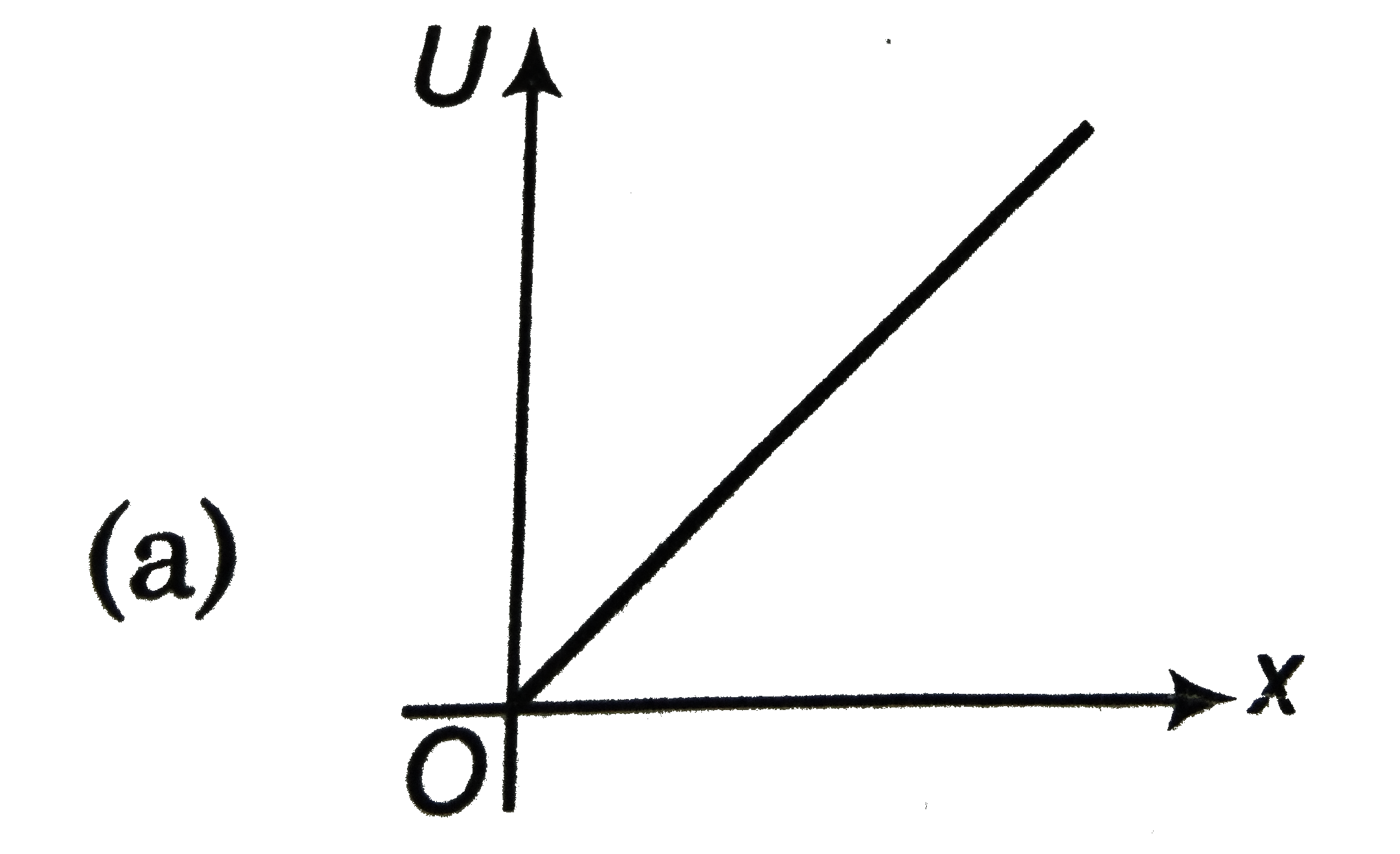
D. None of these

Answer

C

Explanation

To solve the problem of identifying the correct variation of potential energy $$U$$ as a function of displacement $$x$$ from the mean position of a harmonic oscillator, we can follow these steps:

Step 1: Understand the Potential Energy Formula
The potential energy \( U \) of a harmonic oscillator is given by the formula:
\( U = \frac{1}{2} k x^2 \)
where \( k \) is the spring constant and \( x \) is the displacement from the mean position.

Step 2: Analyze the Potential Energy at the Mean Position
At the mean position, the displacement \( x = 0 \). Substituting this into the potential energy formula:
\( U = \frac{1}{2} k (0)^2 = 0 \)
This confirms that the potential energy \( U \) is zero at the mean position.

Step 3: Identify the Relationship Between \( U \) and \( x^2 \)
From the formula \( U = \frac{1}{2} k x^2 \), we can see that potential energy \( U \) is directly proportional to the square of the displacement \( x^2 \). This means:
\( U \propto x^2 \)
This indicates that if we plot \( U \) against \( x^2 \), we will get a linear relationship.

Step 4: Graphical Representation
When plotting \( U \) against \( x^2 \):
- The graph will be a straight line starting from the origin (0,0) because when \( x^2 = 0 \), \( U = 0 \).
- The slope of the line will be \( \frac{1}{2} k \), which is a constant.

Step 5: Conclusion
Thus, the correct variation of potential energy \( U \) as a function of \( x^2 \) is a straight line passing through the origin. Therefore, the answer is that \( U \) varies linearly with \( x^2 \).