Binary stars of comparable masses $m_{1}$ and $m_{2}$ rotate under the influence of each other's gravity with a time period $T$ . If they are stopped suddenly in their motions, find their relative velocity when they collide with each constant of gravitation.

Video Solution

Text Solution

Verified by Experts

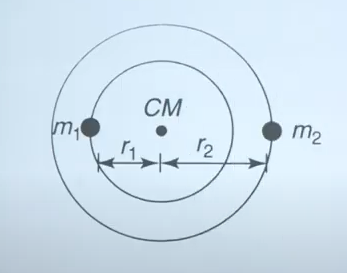
Both the stars rotate about their center of mass (COM).

For the position of $C O M$ , $\frac{r_{1}}{m_{2}} = \frac{r_{2}}{m_{1}} = \frac{r_{1} + r_{2}}{m_{1} + m_{2}} = \frac{r}{m_{1} + m_{2}}$ $(r = r_{1} + r_{2})$
Also $m_{1} r_{1} ω^{2} = \frac{G m_{1} m_{2}}{r_{2}}$
or $ω^{2} = \frac{G m_{2}}{r_{1} r^{2}}$ $(ω = \frac{2 π}{T})$
But, $r_{1} = \frac{m_{2} r}{m_{1} + m_{2}}$
$∴ ω^{2} = \frac{G (m_{1} + m_{2})}{r^{3}}$
or $r = {\frac{G (m_{1} + m_{2})}{ω^{2}}}^{1 / 3}$ ..(i)
Applying conservation of mechanical energy, we have
$- \frac{G m_{1} m_{2}}{r} = - \frac{G m_{1} m_{2}}{(R_{1} + R_{2})} + \frac{1}{2} μ υ_{r}^{2}$ ..(ii)
Hence, $μ =$ reduced mass
$= \frac{m_{1} m_{2}}{m_{1} + m_{2}}$
and $υ_{r} =$ relative between the two stars.
From Eq. (ii), we find that
$υ_{r}^{2} = \frac{2 G m_{1} m_{2}}{μ} (\frac{1}{R_{1} + R_{2}} - \frac{1}{r})$
$= \frac{2 G m_{1} m_{2}}{\frac{m_{1} m_{2}}{m_{1} + m_{2}}} (\frac{1}{R_{1} + R_{2}} - \frac{1}{r})$
$= 2 G (m_{1} + m_{2}) (\frac{1}{R_{1} + R_{2}} - \frac{1}{r})$
Substituting the value of $r$ from Eq. (i), we get
$υ_{r} = \sqrt{2 G (m_{1} + m_{2}) [\frac{1}{R_{1} + R_{2}} - {\frac{4 π^{2}}{G (m_{1} + m_{2}) T^{2}}}^{1 / 3}]}$

Updated on:21/07/2023

Binary stars of comparable masses rotates under the influence of each other's gravity at a distance ${[\frac{2 G}{ω^{2}}]}^{1 / 3}$ where $ω$ is the angular velocity of each of the system. If difference between the masses of two stars is $6$ units. Find the ratio of the masses of smaller to bigger star.

A $4 : 10$
B $1 : 7$
C $2 : 8$
D $3 : 9$
Two particles of mass $m_{1}$ and $m_{2}$ , approach each other due to their mutual gravitational attraction only. Then
Aacceleration of both the particles are equal.
Bacceleration of the particle of mass $m_{1}$ is proportional to $m_{1}$
Cacceleration the particle of mass $m_{1}$ is proportional to $m_{2}$ .
Daccelaration of the particle of mass $m_{1}$ is inversely proportional to $m_{1}$ .
Two particles of mass $m_{1}$ and $m_{2}$ , approach each other due to their mutual gravitational attraction only. Then
Aaccelerations of both the particles are equal.
Bacceleration of the particle of mass $m_{1}$ is proportional to $m_{1}$ .
Cacceleration of the particle of mass $m_{1}$ is proportional to $m_{2}$ .
Dacceleration of the particle of mass $m_{1}$ is inversely proportional to $m_{1}$ .