Two large rigid vertical walls A and B are parallel to each other and separated by 10m. A particle of mass 10g is projected with an initial velocity of 20m/s at 45∘ to the horizontal from point P on the ground, such that AP=5m. The plane of motion of the particle is vertical and perpendicular to the walls. Assuming that all the collisions are perfectly elastic, find the maximum height attained by the particle and the total number of collisions suffered by the particle with the walls before it hits ground. Take g=10m/s2.

The correct Answer is:A
As the collisions are perfectly elastic, collsion of the ball will not affect the vertical component of its velocity while the horizontal component will be simply reserved.
Hence, Hmax=v2y2g=[20×sin45∘]22×10=10m
Total time of flight
T=2vyg=2×20×(1√2)10=2√2s
Total horizontal distance travelled before striking the ground x=vxT=40m
PB+BA+AB+BA+AB=45m
Hence, total number of collision suffered by the particle with the walls before it hits ground =4.
Topper's Solved these Questions
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBook:DC PANDEYChapter:CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONExercise:Level 2 Comprehension Based Questions
Explore 3 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBook:DC PANDEYChapter:CENTRE OF MASS, IMPULSE AND MOMENTUMExercise:Comprehension type questions
Explore 15 Videos
- CIRCULAR MOTIONBook:DC PANDEYChapter:CIRCULAR MOTIONExercise:Medical entrances s gallery
Explore 19 Videos
Similar Questions
A particle is projected with a velocity 10 m//s at an angle 37∘ to the horizontal. Find the location at which the particle is at a height 1 m from point of projection.
View SolutionThe particle of mass 1 kg is projected with velocity 20√2m/s at 45∘ with ground . When , the particle is at highest point (g=10m/s2),
View Solution
Knowledge Check
A particle is projected with velocity of 10ms at an angle of 15° with horizontal.The horizontal range will be(g=10ms2)
A10mB5mC2.5mD1mA particle is projected with velocity 10m/s at an angle of 45∘ with the horizontal from some height. Consider point of projection as origin and motion in xy plane. Then (→g=10ˆjm/s2)
AIts velocity after √2 sec will be perpendicular to its initial velocityBIts velocity after √3 sec will be perpendicular to its initial velocityCIts radius of curvature is 40m at x=5(√3+1) mDIts radius of curvature is 5m at x=5mA particle is thrown vertically upward from the ground with some velocity and it strikes the ground again in time 2 s. The maximum height achieved by the particle is : (g=10m/s2)
A2.50 mB1.25 mC6.25 mD5 m
A particle is projected from the ground with an initial velocity of 20m/s at angle fo 30∘ with the horizontal. What is the magnitude of chang in velocity in 0.5scond ? ( g= 10 m//s^2).
View SolutionA ball is projected from the point O with velocity 20 m/s at an angle of 60∘ with horizontal as shown in figure. At highest point of its trajectory it strikes a smooth plane of inclination 30∘ at point A. The collision is perfectly inelastic. The maximum height from the ground attained by the ball is (g=10m/s2)

View SolutionA particle is projected from ground with velocity 20(√2)m/s at 45∘. At what time particle is at height 15 m from ground? (g=10m/s2)
View SolutionA ball projected with a velocity of 10m/s at angle of 30∘ with horizontal just clears two vertical poles each of height 1m. Find separation between the poles.
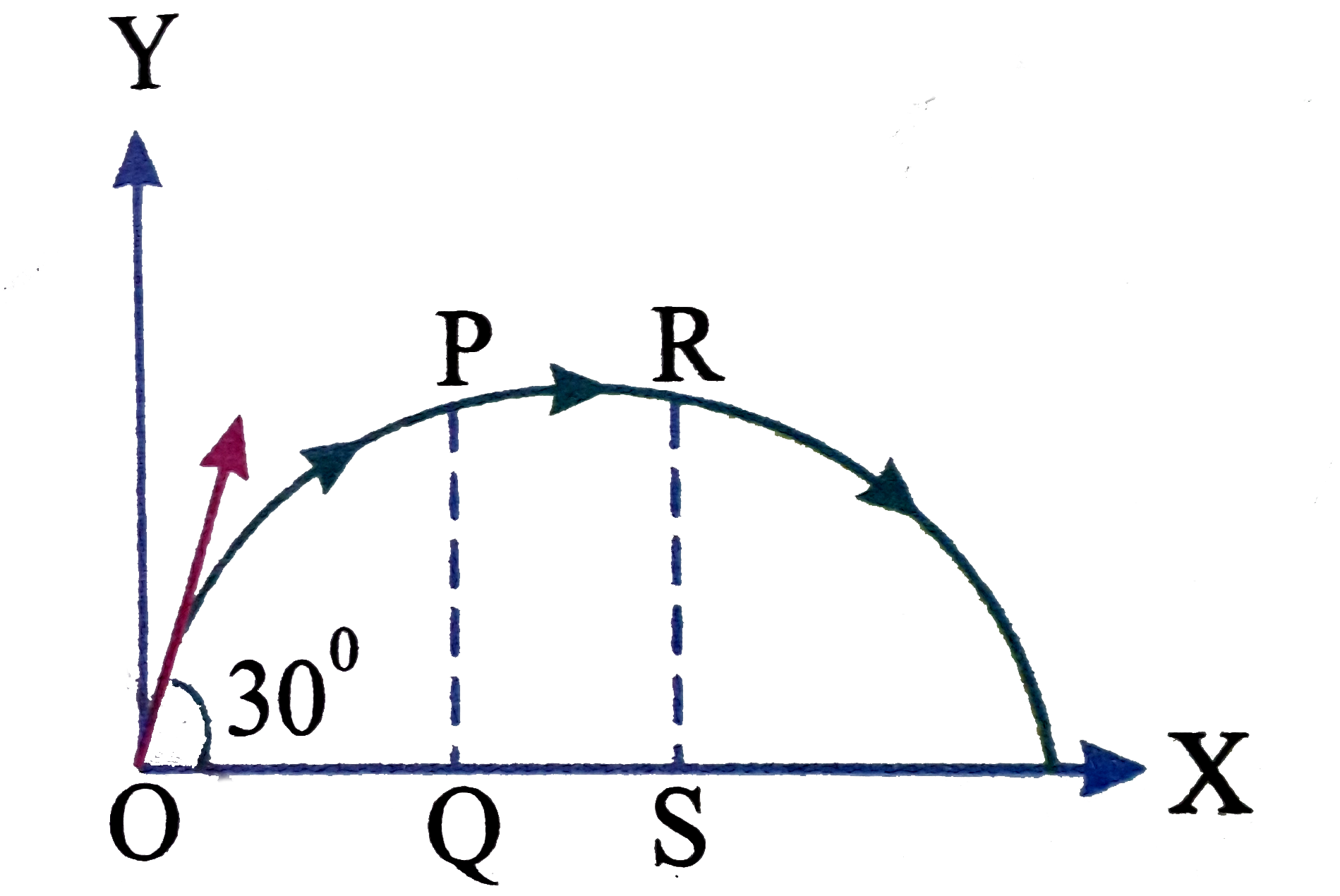
View SolutionA particle is projected from ground with some initial velocity making an angle of 45∘ with the horizontal. It reaches a height of 7.5m above the ground while it travels a horizontal distance of 10m from the point of projection. The initial speed of the projection is
View Solution