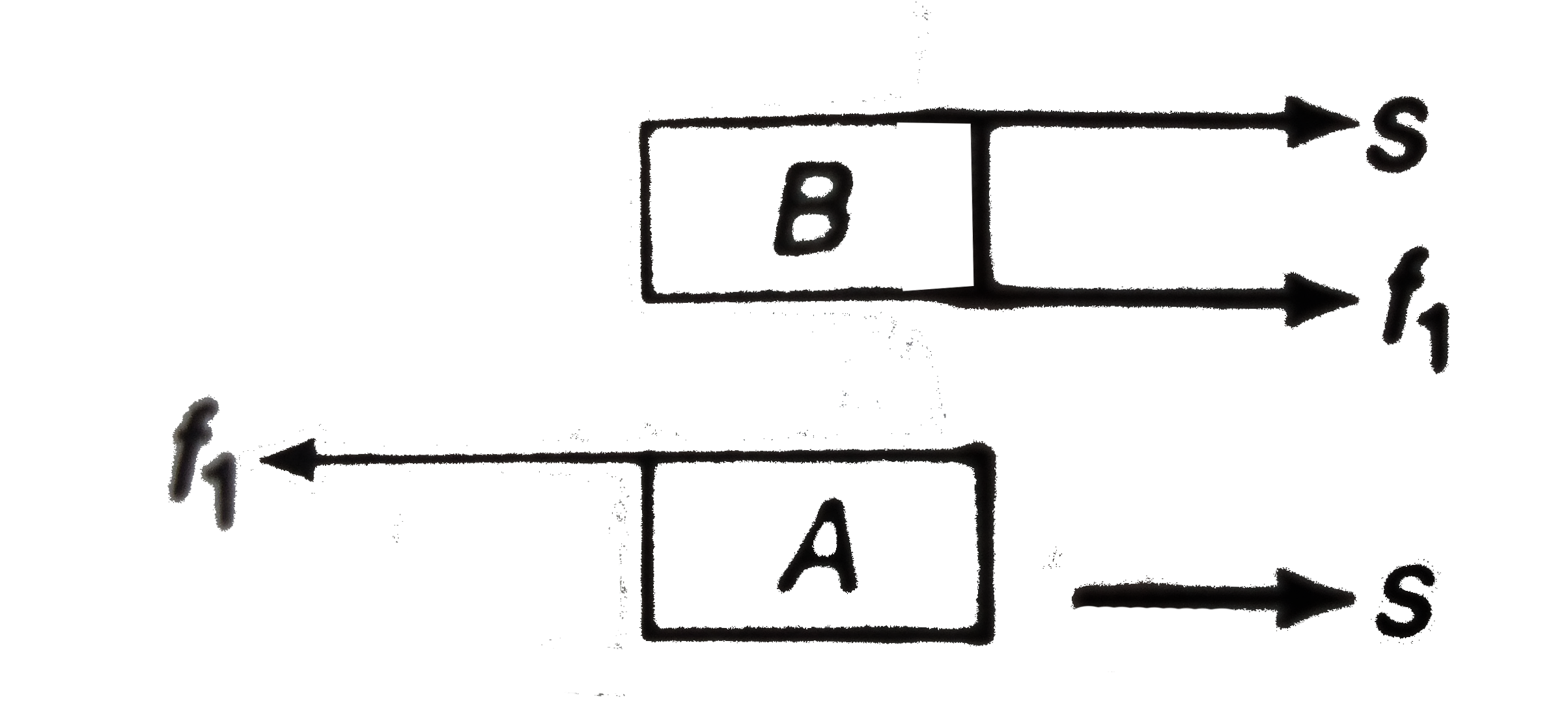
Asseration : A plank A is placed on a rough surface over which a block B is placed. In the shown situation, elastic cord is unstretched. Now a gradually increasing force F is appled slowly on A until the relative motion between the block and plank starts.
 .
.
At this moment cord is making an angleθ with the vertical. Work done by force F is equal to energy lost against fricton f2, plus potential energy stored in the cord.
Reason : work done by static friction f1 on the system as a whole is zero.
If both Assertion and Reason are true and the Reason is correct explanation of the Assertion.
If both Assertion and Reason are true and the Reason is not the correct explanation of the Assertion.
If Assertion is true, but the Reason is false
If Assertion is false but the Reason is true.
The correct Answer is:A
(Wf1)onA=−f1s
(Wf1)onB=−f1s
∴ Net work done by f1=0
 .
.
Similar Questions
A block of mass m is placed on a wedge of mass M and a force F is acting on its as shown . If all surfaces are smooth , find the force F such that, there is no relative motion between m and M.
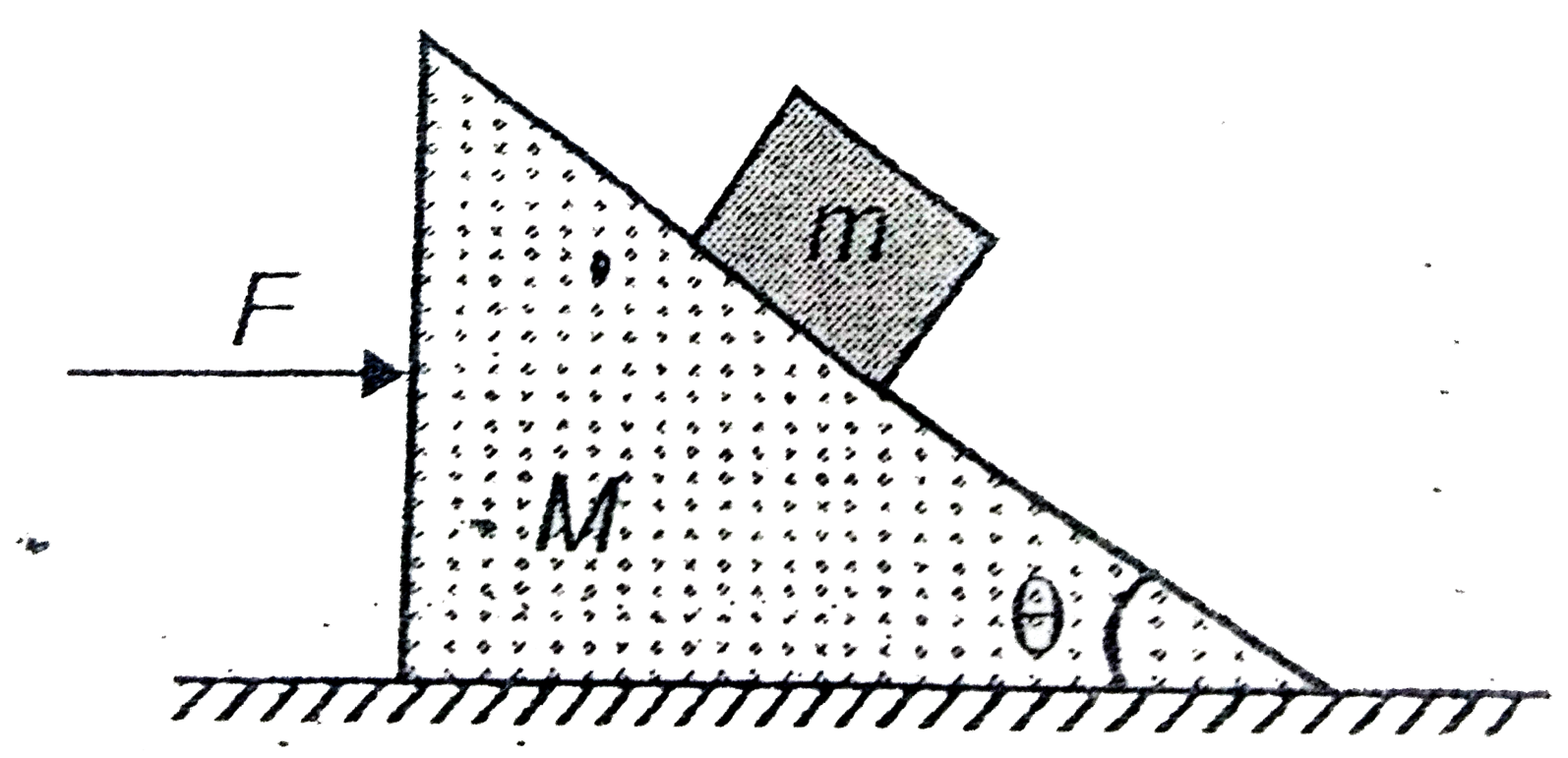
View SolutionA block of mass m is placed on a wedge of mass M and a force F is acting on it as shown . If all surface are smooth , find the force F such that , there is no relative motion between m and M .

View Solution
Knowledge Check
For the arrangement as shown in the figure the maximum force F, for no relative motion between blocks is
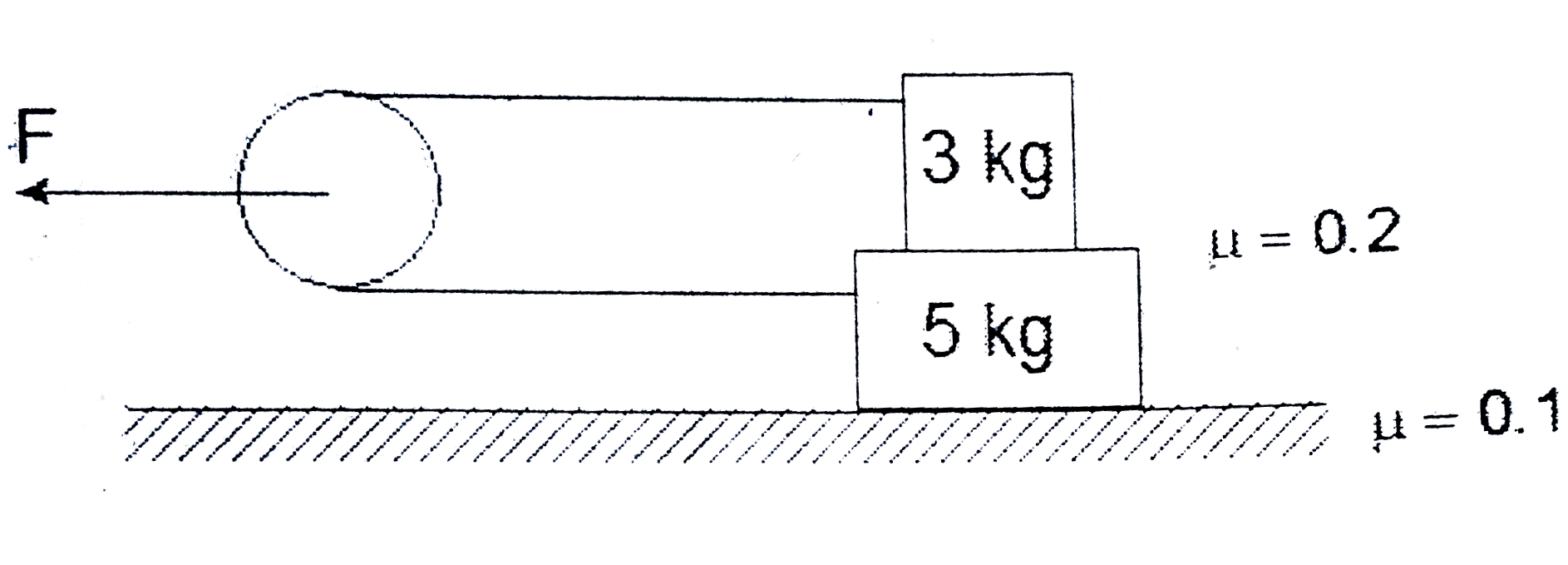 A12NB8NC20ND24N
A12NB8NC20ND24NA horizontal plane supports a plank with a block placed on it. A light elastic string is attached to the block, which is attached to a fixed point O. Initially, the cord is unstretched and vertical. The plank is slowly shifted to right until the block starts sliding over it. It occurs at the moment when the cord deviates from vertical by an angle θ=0∘. Work done by the force F equals
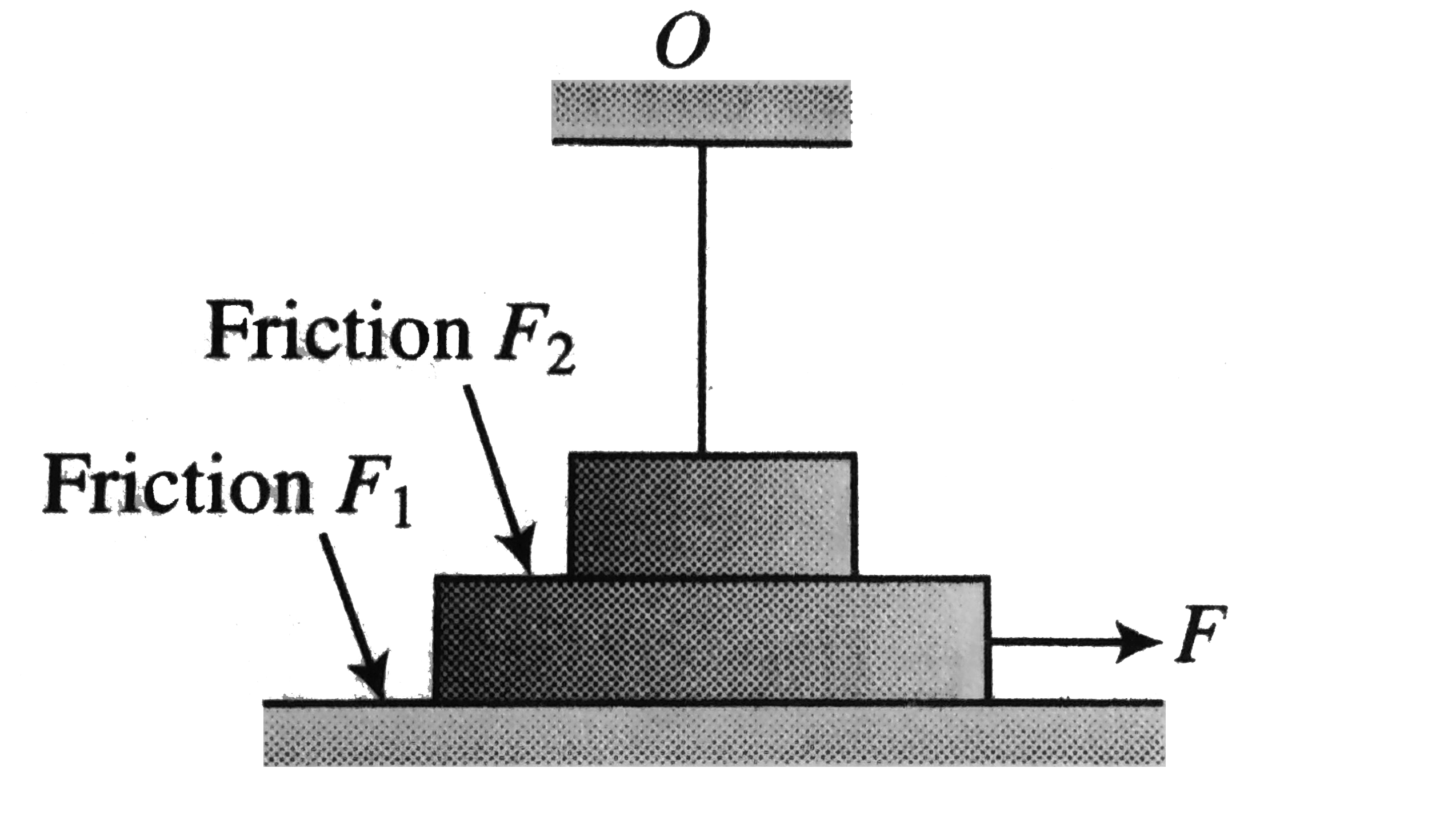 A(a) Energy lost against friction F1 plus strain energy in cordB(b) Work done against total friction acting on the plank aloneC(c) Work done against total friction acting on the plank plus strain energy in the cordD(d) Work done against total friction acting on the plank plus strain energy in the cord minus work done by friction acting on the block
A(a) Energy lost against friction F1 plus strain energy in cordB(b) Work done against total friction acting on the plank aloneC(c) Work done against total friction acting on the plank plus strain energy in the cordD(d) Work done against total friction acting on the plank plus strain energy in the cord minus work done by friction acting on the blockA disc of mass m and radius R is placed over a plank of same mass m. There is sufficient friction between disc and plank to prevent slipping. A force F is applied at the centre of the disc.

Force of friction between the disc and the plank isAF2BF4CF3D2F3
A block of mass 10 kg is kept over a rough surface and a force F=4t is applied on it. At what value of t the block will start moving :-

View SolutionBlock A of mass 1kg is placed on the rough surface of block B of mass 3kg. Block B is placed on smooth horizontal surface. Blocks are given the velocities as shown. Find net work done by the frictional force. [in (−)veJ].
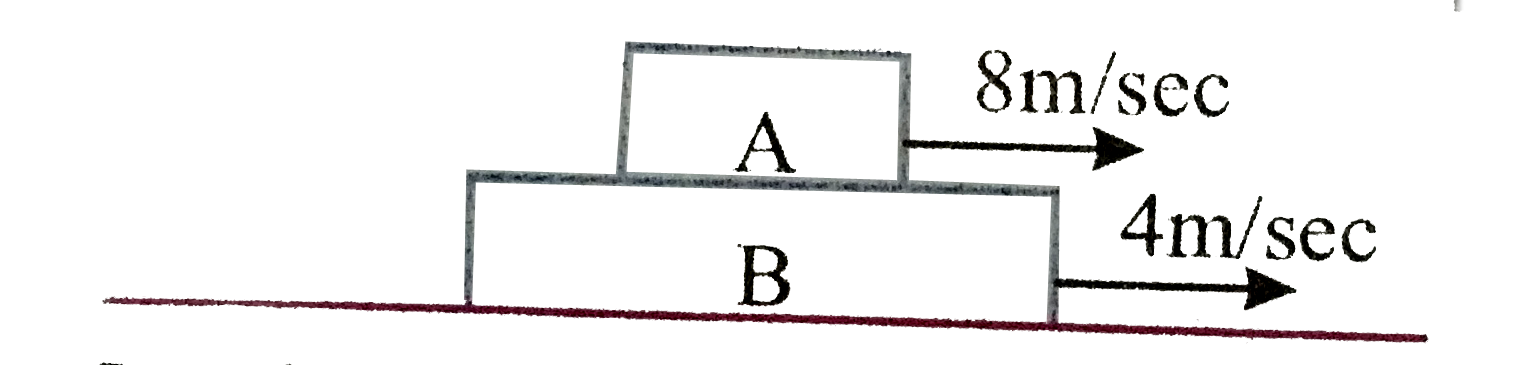 .
.
View SolutionA block of mass M rests on a rough horizontal surface as shown. Coefficient of friction between the block and the surface is μ. A force F=mg acting at angle θ with the vertical side of the block pulls it. In which of the following cases the block can be pulled along the surface?

View SolutionA body is placed on a rough inclined plane of inclination θ. As the angle θ is the increased from 0∘ to 90∘, the contact force between the block and the plane

View SolutionA body is placed on a rough inclined plane of inclination θ. As the angle θ is the increased from 0∘ to 90∘, the normal force between the block and the plane is,
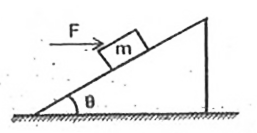
View Solution
