Question

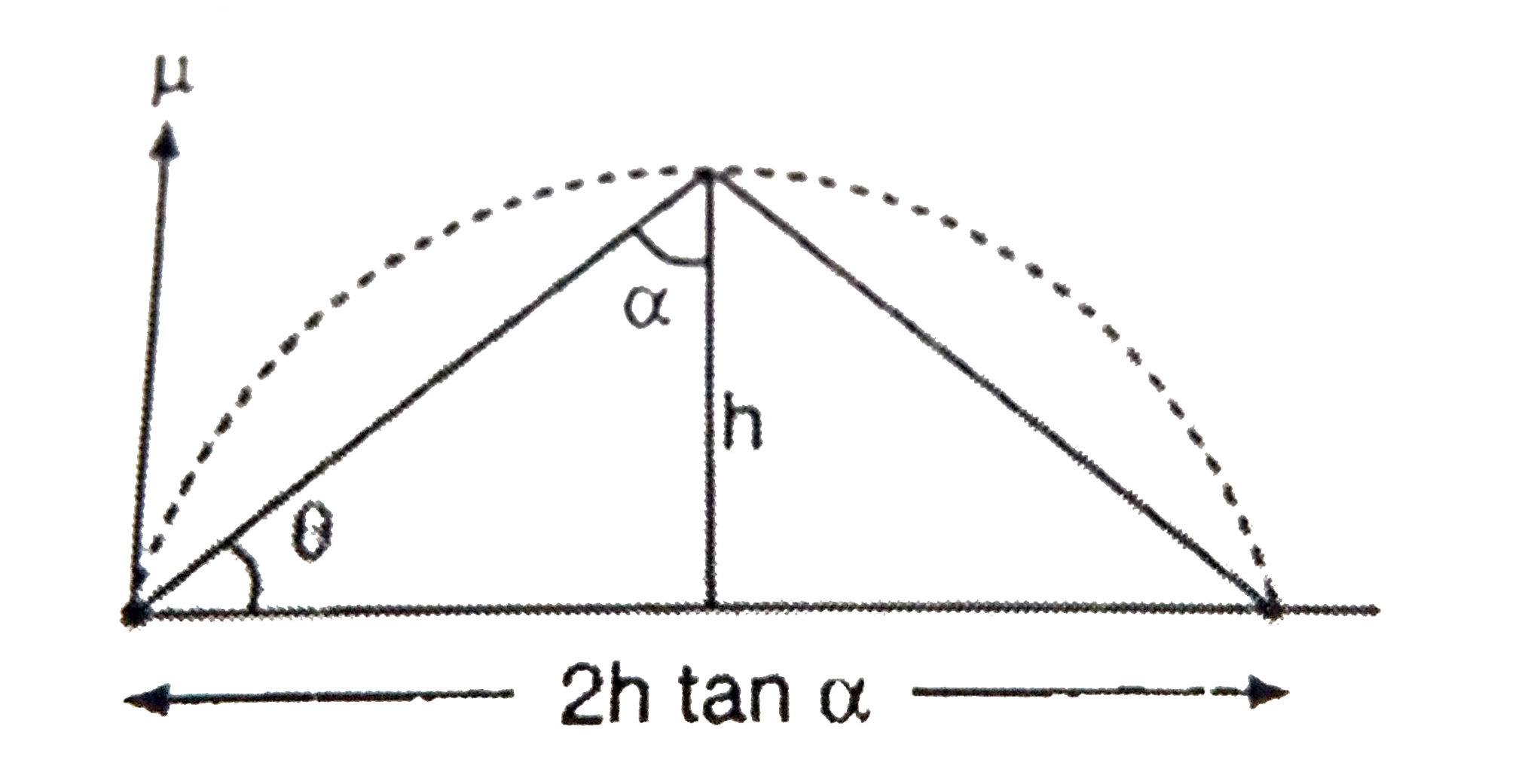
A particle is thrown over a triangle from one end of a horizontal base and after grazing the vertex falls on the other end of the base. If $\alpha \text{and}\beta$ be the base angles and $\theta$ the angle of projection, prove that $\tan \theta =\tan \alpha +\tan \beta$ .

Answer

A

Explanation

To prove that $$\tan \theta =\tan \alpha +\tan \beta$$, we will follow these steps:

Step 1: Define the Triangle and Variables
Let triangle \( ABC \) have base \( AB \) with angles \( \alpha \) at point \( A \) and \( \beta \) at point \( B \). The height from point \( C \) to base \( AB \) is \( y \). The horizontal distance from point \( A \) to the vertex \( C \) is \( x \), and the distance from vertex \( C \) to point \( B \) is \( r - x \), where \( r \) is the total length of the base \( AB \).

Step 2: Express Tangents in Terms of Height and Distances
Using the definition of tangent in right triangles:
- For angle \( \alpha \):
\( \tan \alpha = \frac{y}{x} \)
- For angle \( \beta \):
\( \tan \beta = \frac{y}{r - x} \)

Step 3: Add the Tangents
Now, we can add the two expressions for \( \tan \alpha \) and \( \tan \beta \):
\( \tan \alpha + \tan \beta = \frac{y}{x} + \frac{y}{r - x} \)

Step 4: Find a Common Denominator
To combine the fractions, we find a common denominator:
\( \tan \alpha + \tan \beta = \frac{y(r - x) + yx}{x(r - x)} = \frac{yr}{x(r - x)} \)

Step 5: Use the Equation of Trajectory
The equation of the projectile's trajectory can be expressed as:
\( y = x \tan \theta \left(1 - \frac{x}{r}\right) \)
From this, we can rearrange to find:
\( y = x \tan \theta - \frac{x^2 \tan \theta}{r} \)

Step 6: Substitute for \( y \)
Now, we can express \( y \) in terms of \( \tan \theta \):
\( y = \frac{x \tan \theta r}{r - x} \)

Step 7: Equate the Two Expressions for \( y \)
Now we have two expressions for \( y \):
1. From the tangent addition:
\( y = \frac{yr}{x(r - x)} \)
2. From the trajectory equation:
\( y = \frac{x \tan \theta r}{r - x} \)

Equating these gives:
\( \frac{yr}{x(r - x)} = \frac{x \tan \theta r}{r - x} \)

Step 8: Simplify and Conclude
By simplifying, we find:
\( \tan \theta = \tan \alpha + \tan \beta \)

Thus, we have proved that:
\( \tan \theta = \tan \alpha + \tan \beta \)