The figure shows a modified Young’s double slit experimental set-up. Here SS2−SS1=λ/4.
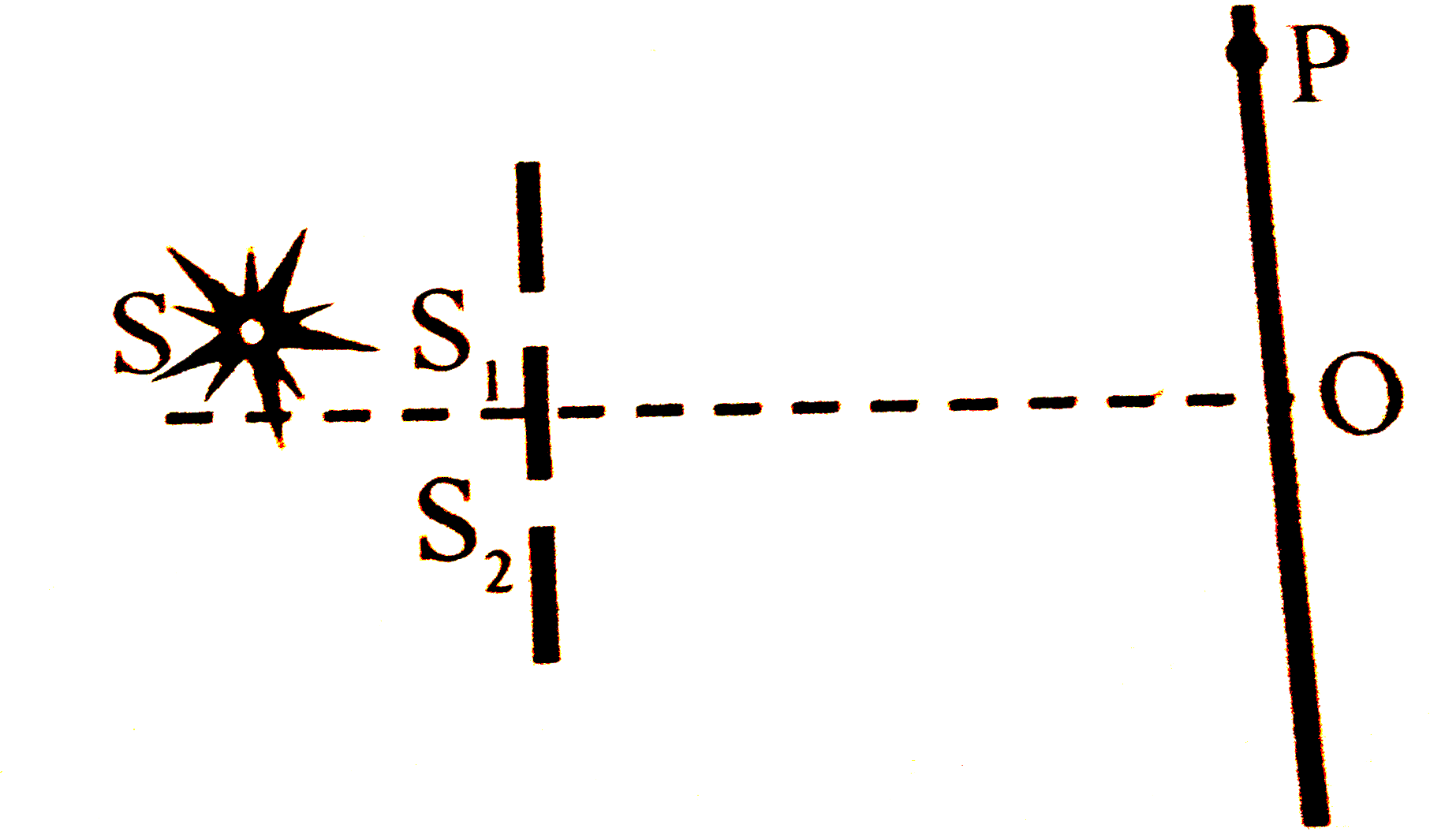
(a) Write the condition for constructive interference.
(b) Obtain an expression for the fringe width.
The path difference S2P−S1P=[yndD+λ4]
For constructive interference , path difference =nλ,
Where n=0,1,2,3,…..
[yndD+λ4]=nλ,
So, position of nth bright fringe is =yn=(n−14)λDd
Fringe widthβ=yn−yn−1
Or β=λDd
Topper's Solved these Questions
Similar Questions
The figure shows a modified Young's double slit experimental set-up.
Here SS2−SS1=λ/4.
(a) Write the condition for constructive interference.
(b) Obtain an expression for the fringe width.
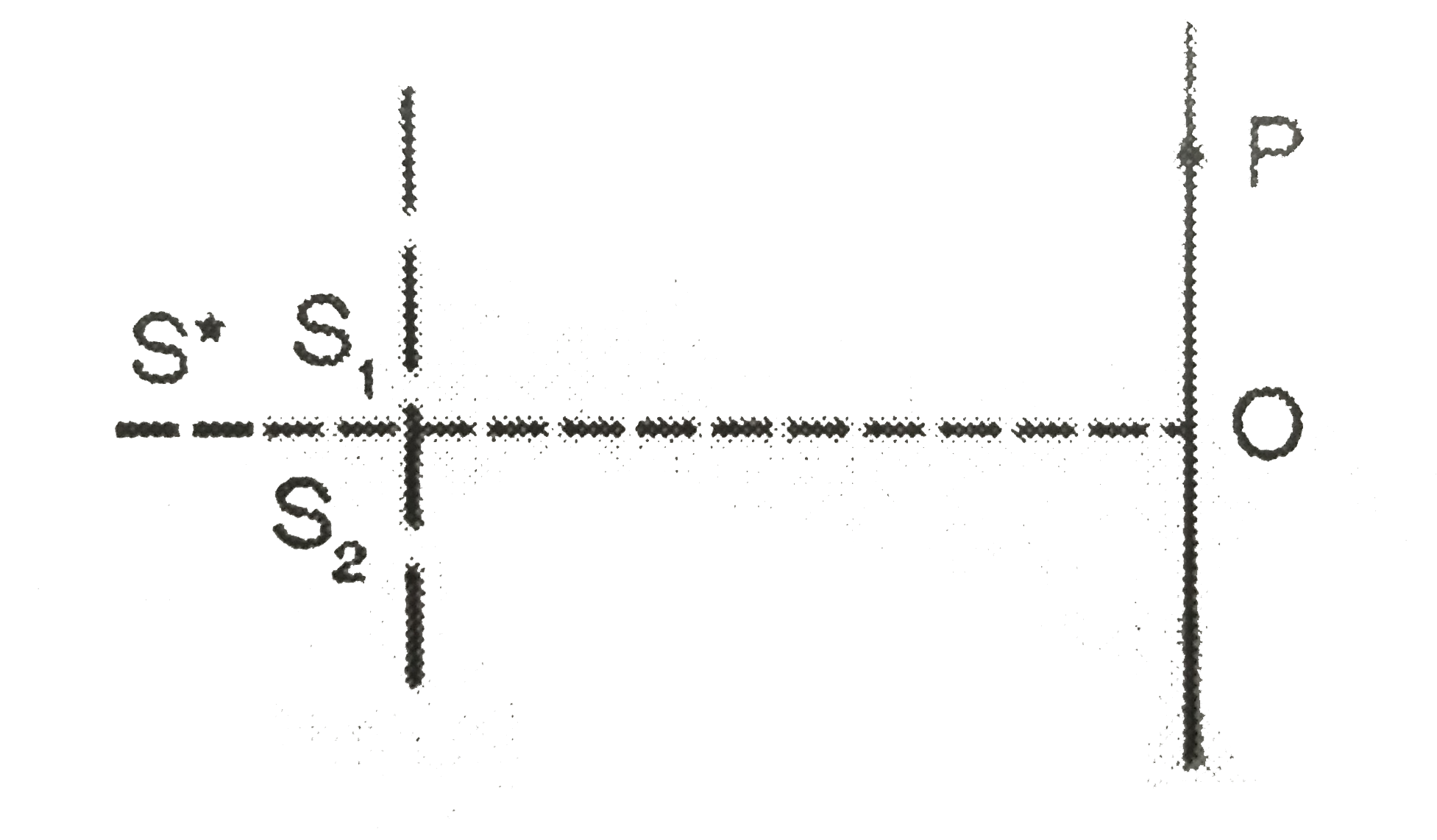
View SolutionFig. shows an experimental set up simillar to Young's double slit experiment to observe interference of light. Here SS2−SS1=λ/4. Write down the conditions of (i) Contstructive interference
(ii) Destructive interference at any point P in terms of path diff. (S2P−S1P). Does the central fringe observed in the above set up lie above or below O ? Give reason.
View Solution
Knowledge Check
The fringe width in Young’s double slit experiment increases when
AWavelength increasesBDistance between the slits increasesCDistance between the source and screen decreasesDThe width of the slits increasesIn a Young's double slit experiment, let S1 and S2 be the two slits, and C be the centre of the screen. If ∠S1CS2=θ and λ is the wavelength, the fringe width will be
AλθBλθC2λθDλ2θIn a Young's double-slit experiment, let S1 and S2 be the two slits, and C be the centre of the screen. If ∠S1CS2=θ and λ is wavelength, the fringe width will be
AλθBλθC2λθDλ2θ
State the importance of coherent sources in the phenomenon of interference.
In Young's double-slit experiment to produce interference pattern, obtain the conditions for constructive and destructive interference.
Hence, deduce the expression for the fringe width.
How does the fringe width get affected, if the entire experimental apparatus of Young's is immersed in water ?
View SolutionState the importance of coherent sources in the phenomenon of interference. In Young's double slit expet-ment to produce interference pattern obtain the conditions for constructive and destructive interference. Hence deduce the expression for the fringe width. How does the firinge width get affected, if the entire experimental apparatus of Young's is immersed in water ?
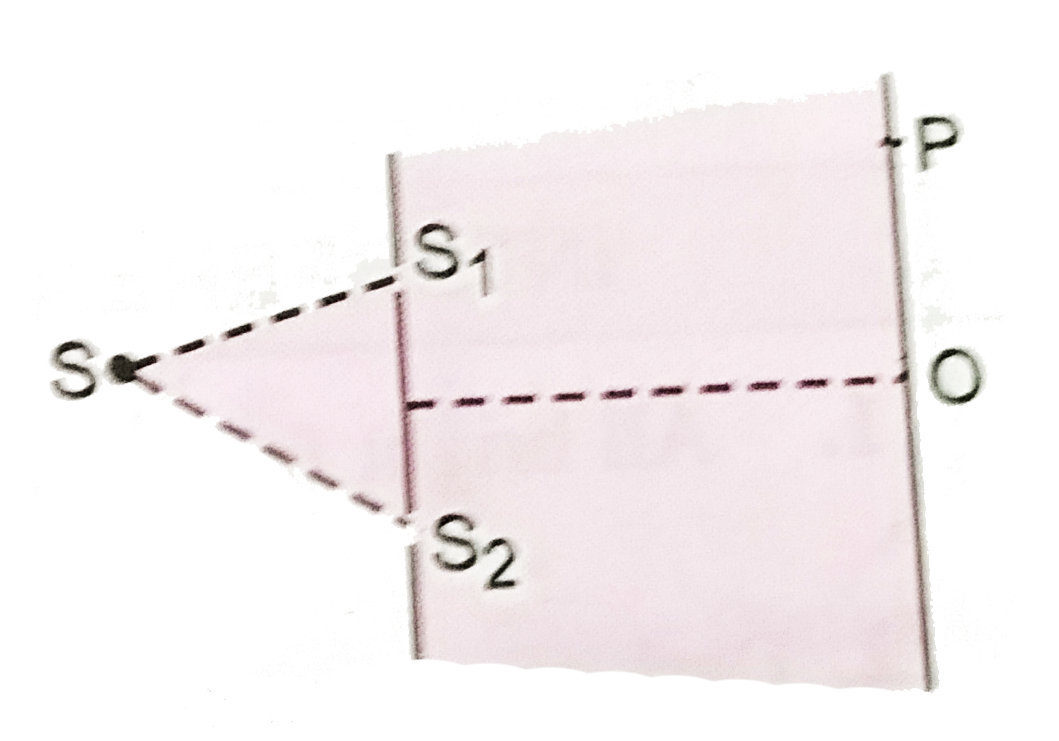
View SolutionIn a modified set up of Young's double slit experiment, it is given that SS2−SS1=λ4 i.e., the source S is not equidistant from the slits S1andS2.
(a) Obtain the condition for constructive and destructive interference at any point P on the screen in terms of the path difference Δ=S2P−S1P.
(b) Does the observed central bright fringe lie above or below O ? Give reason in support of your answer.
View Solution(a) In Young's double-slit experiments, deduce the conditions for obtaining constructive and desctructive interference fringes. Hence deduce the expression for the fringe width.
(b) Show that the fringe pattern on the screen is actually a superposition of single slit diffraction from each slit.
(c ) What should be the width of each slit to obtain 10 maxima of the double-slit pattern within the central maximum of the single slit pattern, for green light of wavelength 500 nm, if the separation between two slits is 1 mm?
View Solution(a) In Young's double slit experiment, describe briefly how bright and dark frings are obtained on the screen kept in front of a double slit. Hence obtain the expression for the fringe width.
(b) The ratio of the intensities at minima to the maxima in the Young's double slit experiment is 9 : 25. Find the ratio of the widths of the two slits.
View Solution
