A body of mass 1 kg is made to oscillate on a spring of force constant 16 N/m. Calculate (a) Angular frequncy , (b) Frequency of vibrations.
To solve the problem step by step, we will calculate the angular frequency and the frequency of vibrations for a mass oscillating on a spring.
Given Data:
- Mass (m) = 1 kg
- Spring constant (k) = 16 N/m
Step 1: Calculate Angular Frequency (ω)
The formula for angular frequency (ω) in a spring-mass system is given by:
ω=√km
Substituting the given values:
ω=√16N/m1kg
Calculating the value:
ω=√16=4radians/second
Step 2: Calculate Frequency of Vibrations (f)
The relationship between angular frequency (ω) and frequency (f) is given by:
ω=2πf
From this, we can express frequency (f) as:
f=ω2π
Substituting the value of ω we found:
f=42π
Calculating the value:
f=42×3.14≈46.28≈0.6369Hz
Final Answers:
(a) Angular frequency (ω) = 4 radians/second
(b) Frequency of vibrations (f) ≈ 0.6369 Hz
---
- Mass (m) = 1 kg
- Spring constant (k) = 16 N/m
The formula for angular frequency (ω) in a spring-mass system is given by:
The relationship between angular frequency (ω) and frequency (f) is given by:
(a) Angular frequency (ω) = 4 radians/second
(b) Frequency of vibrations (f) ≈ 0.6369 Hz
Topper's Solved these Questions
- FEBRUARY 2019BOOK - GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERSCHAPTER - FEBRUARY 2019EXERCISE - SECTION-C
12 Videos
- FEBRUARY 2019BOOK - GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERSCHAPTER - FEBRUARY 2019EXERCISE - SECTION-D
6 Videos
- FEBRUARY 2019BOOK - GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERSCHAPTER - FEBRUARY 2019EXERCISE - SECTION-D
6 Videos
- FEBRUARY 2018BOOK - GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERSCHAPTER - FEBRUARY 2018EXERCISE - SECTION-II
21 Videos
- JULY 2016BOOK - GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERSCHAPTER - JULY 2016EXERCISE - SECTION -II
21 Videos
Similar Questions
Knowledge Check
If a body of mass 0.98 kg is made to oscillate on a spring of force constant 4.84 N/m the angular frequency of the body is
A1.22 rad/sB2.22 rad/sC3.22 rad/sD4.22 rad/sA body of mass 1 kg suspended by a light vertical spring of spring constant 100 N/m executes SHM. Its angular frequency and frequency are
A5π rad/s, 100 HzB10 rad/s, π5 HzC10 rad/s, 10π HzD10 rad/s, 5π HzTwo spring of force constants K and 2K are connected a mass m below The frequency of oscillation the mass is
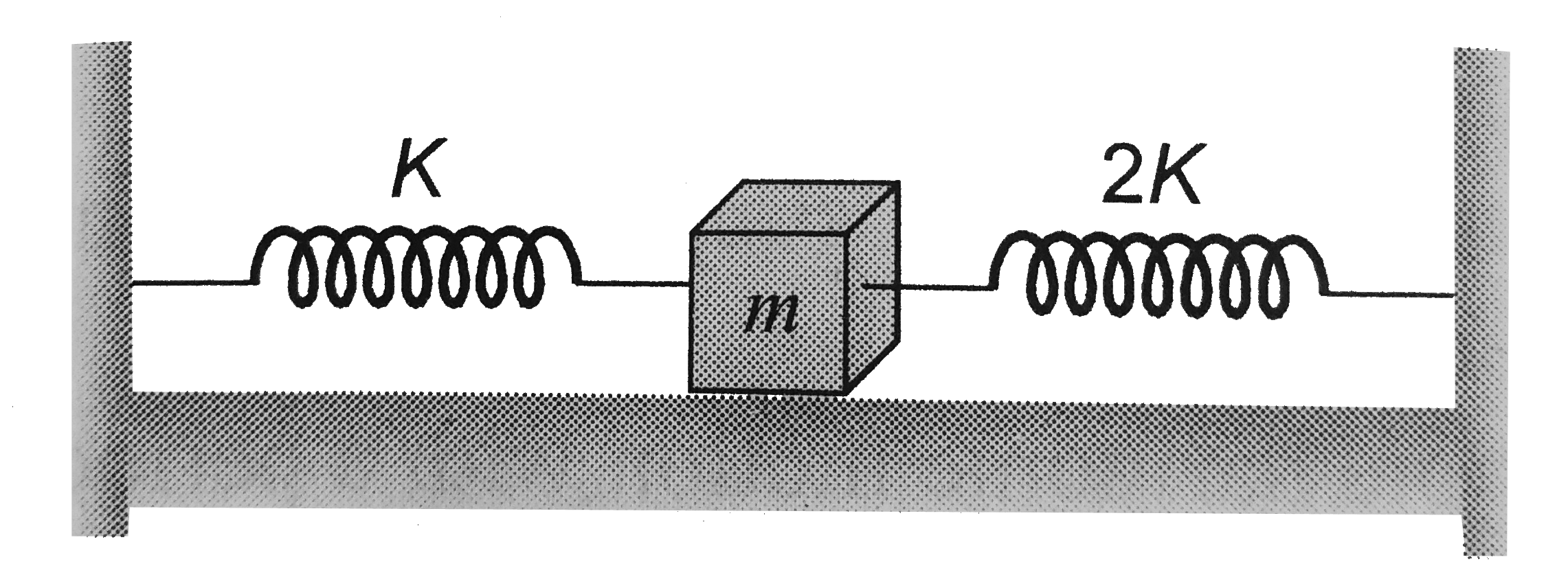 A(12π)√(Km)B(12π)√(2Km)C(12π)√(3Km)D(12π)√(mK)
A(12π)√(Km)B(12π)√(2Km)C(12π)√(3Km)D(12π)√(mK)
A body of mass 16 kg is made to oscillate on a spring of force constant 100Nkg−1. Deduce the angular frequency.
View SolutionA mass of 2kg oscillates on a spring with force constant 50N/m. By what factor does the frequency of oscillation decrease when a damping force with constant b=12 is introduced ?
View SolutionA block of mass 0.2 kg is attached to a mass less spring of force constant 80 N/m as shown in figure. Find the period of oscillation. Take g=10m/s2. Neglect friction

View SolutionA body of mass 0.5 kg is suspended by a weightless spring of force constant 500 Nm−1. Another body of mass 0.1 kg moving vertically upwards with velocity of 5 ms−1 hits the suspended body and gets embeded into the suspended body. What will be the frequency of oscillation ?
View SolutionA body of mass 1.0 kg is suspended from a weightless spring having force constant 600Nm−1. Another body of mass 0.5 kg moving vertically upwards hits the suspended body with a velocity of 3.0ms−1 and gets embedded in it. Find the frequency of oscillations and amplitude of motion.
View Solution
