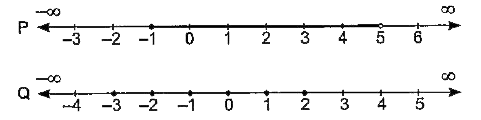
The diagram, given below, represents two inequations P and Q on real number lines :

Write down P and Q in set builder notation.
Step by step video & image solution for The diagram, given below, represents two inequations P and Q on real number lines : Write down P and Q in set builder notation. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - LINEAR INEQUATIONSEXERCISE - Multiple Choice Questions47videos
LINEAR INEQUATIONS
- BOOK - ICSECHAPTER - LINEAR INEQUATIONSEXERCISE - Assertion and Reason Based Questions5videos
LINEAR INEQUATIONS
- BOOK - ICSECHAPTER - LINEAR INEQUATIONSEXERCISE - EXERCISE 4 (B)65videos
LINEAR INEQUATIONS
- BOOK - ICSECHAPTER - HEIGHTS AND DISTANCES EXERCISE - Exercise 22 C28videos
HEIGHTS AND DISTANCES
- BOOK - ICSECHAPTER - LOCI (LOCUS AND ITS CONSTRUCTIONS)EXERCISE - EXERCISE 16(B)49videos
LOCI (LOCUS AND ITS CONSTRUCTIONS)
Similar Questions
The diagrams, given below, represent two inequations A and B on real number line.
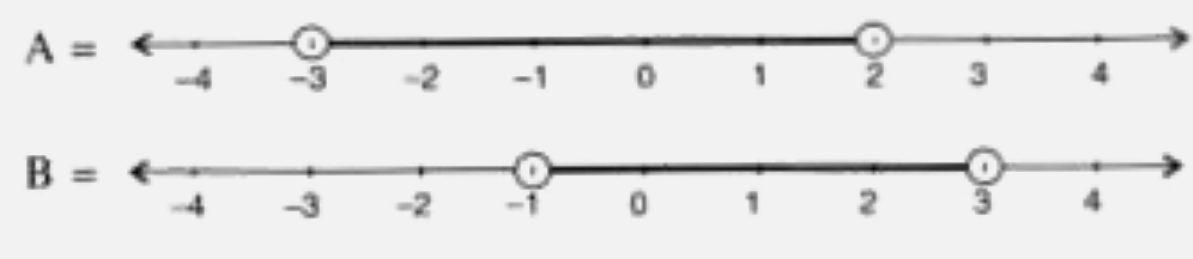
(i) Write down A and B in set builder notations.
(ii) Represent A∩BandA'∩B on two different numbers lines.View SolutionThe diagram represents two inequation A and B on real number lines:
Write down A and B in set builder notation.View Solution
Knowledge Check
- Question 1 - Select One
The following diagram represents the solution of two sets P and Q on the number line:
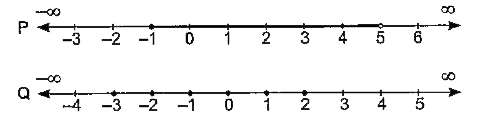
The solution set of Q in set builder form is:A{x:−3<x<2,x∈R}B{x:−3≤x≤2,x∈I}C{x:−3<x≤2,x∈N}D{x:−3≤x<2,x∈W} - Question 2 - Select One
The following diagram represents the solution of two sets P and Q on the number line:
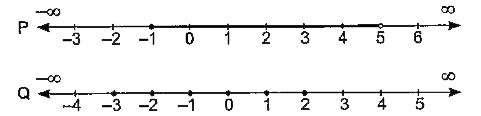
The solution set of P in set-builder form isA{x:−1<x≤5,x∈R}B{x:−1≤x<5,x∈R}C{x:−1≤x≤5,x∈R}D{x:−1<x<5,x∈R} - Question 3 - Select One
If
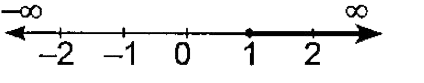 represents solution of a linear inequation in x on a number line, then the solution set in set-builder form is: A{x:x>1,x∈R}B{x:x≥1,x∈R}C{x:x>1,x∈W}D{x:x≥1,x∈W}
represents solution of a linear inequation in x on a number line, then the solution set in set-builder form is: A{x:x>1,x∈R}B{x:x≥1,x∈R}C{x:x>1,x∈W}D{x:x≥1,x∈W}
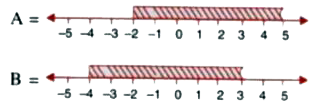
The given diagram represents two sets A and B on real number lines.
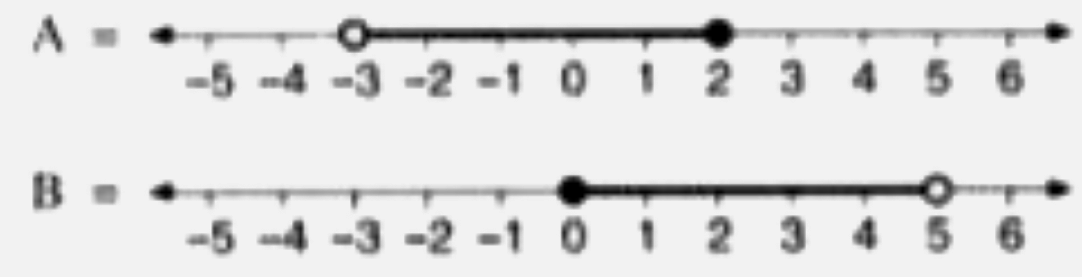
Write down A and B in set-builder notation.View SolutionThe diagram, given below, represents two inequations P and Q on real number lines :

Represent each of the following sets on different number lines :
(a) P∪Q
(b) P∩Q
(c ) P−Q
(d) Q - P
(e ) P∩Q
(f) P∩QView SolutionThe diagram represents two inequation A and B on real number lines:
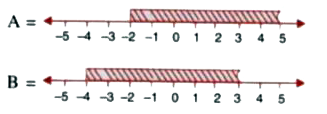
Represent A∩BandA∩B' on two different number lines.View SolutionThe given diagram represents two sets A and B on real number lines.
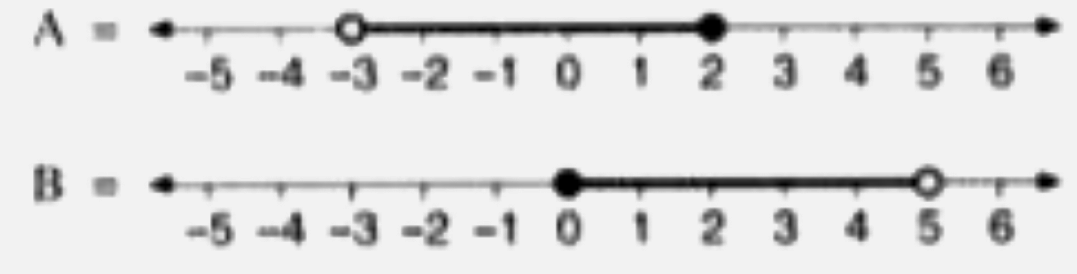
Represent A∪B,A∩B,A'∩B,A−BandB−A on separate number lines.View SolutionThe following diagram represents the solution of two sets P and Q on the number line:
The solution set of Q in roaster form is:View Solution
