Simplify : - 13≤x2−113<16:x∈R.
Graph the values of x on the real number line.
The correct Answer is:2≤x<3
To simplify the given inequality −13≤x2−113<16, we will break it down into two parts and solve each part step by step.
Step 1: Rewrite the Mixed Fraction
First, we convert the mixed fraction 113 into an improper fraction:
113=1+13=33+13=43
So, the inequality can be rewritten as:
−13≤x2−43<16
Step 2: Solve the Left Inequality
Now, we will solve the left part of the inequality:
−13≤x2−43
To isolate x2, we add 43 to both sides:
−13+43≤x2
Calculating the left side:
33≤x2
This simplifies to:
1≤x2
Now, multiply both sides by 2:
2≤x
or
x≥2
Step 3: Solve the Right Inequality
Next, we solve the right part of the inequality:
x2−43<16
Again, we add 43 to both sides:
x2<16+43
To add these fractions, we need a common denominator. The least common multiple of 6 and 3 is 6:
43=86
So, we have:
x2<16+86=96
This simplifies to:
x2<32
Now, multiply both sides by 2:
x<3
Step 4: Combine the Results
From the two parts, we have:
x≥2andx<3
This can be combined into a single inequality:
2≤x<3
Step 5: Graph the Solution on the Real Number Line
To graph the solution 2≤x<3 on the real number line:
- Draw a closed circle at 2 (indicating that 2 is included).
- Draw an open circle at 3 (indicating that 3 is not included).
- Shade the region between 2 and 3.
First, we convert the mixed fraction 113 into an improper fraction:
113=1+13=33+13=43
So, the inequality can be rewritten as:
−13≤x2−43<16
Now, we will solve the left part of the inequality:
−13≤x2−43
To isolate x2, we add 43 to both sides:
−13+43≤x2
Calculating the left side:
33≤x2
This simplifies to:
1≤x2
Now, multiply both sides by 2:
2≤x
or
x≥2
Next, we solve the right part of the inequality:
x2−43<16
Again, we add 43 to both sides:
x2<16+43
To add these fractions, we need a common denominator. The least common multiple of 6 and 3 is 6:
43=86
So, we have:
x2<16+86=96
This simplifies to:
x2<32
Now, multiply both sides by 2:
x<3
From the two parts, we have:
x≥2andx<3
This can be combined into a single inequality:
2≤x<3
To graph the solution 2≤x<3 on the real number line:
- Draw a closed circle at 2 (indicating that 2 is included).
- Draw an open circle at 3 (indicating that 3 is not included).
- Shade the region between 2 and 3.
Topper's Solved these Questions
- LINEAR INEQUATIONSBOOK - ICSECHAPTER - LINEAR INEQUATIONSEXERCISE - Multiple Choice Questions
47 Videos
- LINEAR INEQUATIONSBOOK - ICSECHAPTER - LINEAR INEQUATIONSEXERCISE - Assertion and Reason Based Questions
5 Videos
- LINEAR INEQUATIONSBOOK - ICSECHAPTER - LINEAR INEQUATIONSEXERCISE - EXERCISE 4 (B)
65 Videos
- HEIGHTS AND DISTANCES BOOK - ICSECHAPTER - HEIGHTS AND DISTANCES EXERCISE - Exercise 22 C
28 Videos
- LOCI (LOCUS AND ITS CONSTRUCTIONS)BOOK - ICSECHAPTER - LOCI (LOCUS AND ITS CONSTRUCTIONS)EXERCISE - EXERCISE 16(B)
49 Videos
Similar Questions
Knowledge Check
If 2x−3<x+1<4x+7,x∈R then the solution set of x on the number line is:
A B
B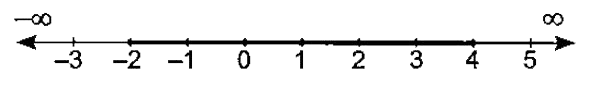 C
C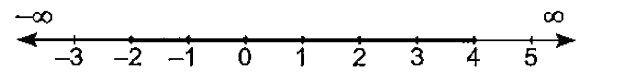 D
D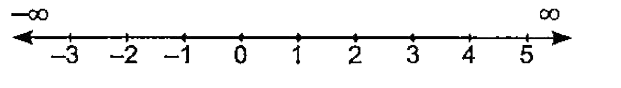
Find the values of x, which satisfy the inequation: −2≤12−2x3<156,x∈N Graph the solution set on the real number line.
View SolutionFind the range of vaues of x, which satisfy the inequality :
−15≤3x10+1<25,x∈R.
Graph the solution set on the number line.
View SolutionFind the range of values of x, which satisfy :
−13≤x2+123<516
Graph, in each of the following cases, the values of x on the different real number lines :
x∈R.
View SolutionFind the range of values of x, which satisfy :
−13≤x2+123<516
Graph, in each of the following cases, the values of x on the different real number lines :
x∈W
View SolutionFind the range of values of x, which satisfy :
−13≤x2+123<516
Graph, in each of the following cases, the values of x on the different real number lines :
x∈Z
View SolutionFind the values of x, which satisfy the inequation:
−2≤12−2x3≤156,x∈N.
Graph the solution on the number line.
View SolutionFind the values of x, which satisfy the inequation −256<12−2x3≤2,x∈W. Graph the solution set on the number line.
View Solution
