In a triangle XYZ, let x, y, z be the lengths of sides opposite to the angles X, Y, Z, respectively, and 2s = x + y + z. If s−x4=s−y3=s−z2 of incircle of the triangle XYZ is 8π3
(a) area of the triangle XYZ is 6√6
(b) the radius of circumcircle of the triangle XYZ is 356√6
(c) sin.X2sin.Y2sin.Z2=435
(d) sin2(X+Y2)=35
The correct Answer is:A, C, D
s−x4=s−y3=s−z2=3s−(x+y+z)9=s9
∴x=5s9,y=2s3,z=7s9
Area of incircle πr2=8π3⇒r2=83
⇒Δ2=r2s2=8s23
⇒s.4s9.s3.2s9=83s2
⇒s=9
∴Δ=√83×9=6√6 sq. units
R=xyz4Δ=5s9.2s3.7s94×6√6=3524√6
sinX2sinY2sinZ2=r4R=√83435√624=435
sin2(X+YZ)=cos2(Z2)=1+cosZ2=35 (Using cosine rule)
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Archives (Matrix Match Type)1videos
PROPERTIES AND SOLUTIONS OF TRIANGLE
- BOOK - CENGAGE ENGLISHCHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Archives (Numerical Value Type)3videos
PROPERTIES AND SOLUTIONS OF TRIANGLE
- BOOK - CENGAGE ENGLISHCHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Archives (JEE Advanced)3videos
PROPERTIES AND SOLUTIONS OF TRIANGLE
- BOOK - CENGAGE ENGLISHCHAPTER - PROGRESSION AND SERIESEXERCISE - ARCHIVES (MATRIX MATCH TYPE )1videos
PROGRESSION AND SERIES
- BOOK - CENGAGE ENGLISHCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Archives(Matrix Match Type)1videos
RELATIONS AND FUNCTIONS
Similar Questions
In a triangle △XYZ, let a,b,c be the lengths o the sides opposite to the angle X,Y and Z respectively. If 1+cos2X−2cos2Y=2sinXsinY, then positive value (s) of ab is (are
View SolutionIf s−x/4= s−y/3=s-z/2 and area of incircle of the triangle XYZ is 8(pi)/3 thenThe radius of the circumcircle of △XYZ
View SolutionIn triangle PQR. ∠Q is a right angle, QR=24, and PR=26. Triangle YXZ is similar to triangle PQR, where vertices X, Y, and Z correspond to vertices P, Q, and R, respectively, and each side of triangle XYZ is 12 the length of the corresponding side of triangle PQR. What is the value of sin Z?
View SolutionIn a ΔPQR (as shown in figure) if x:y:z=2:3:6, then the value of ∠QPR is :
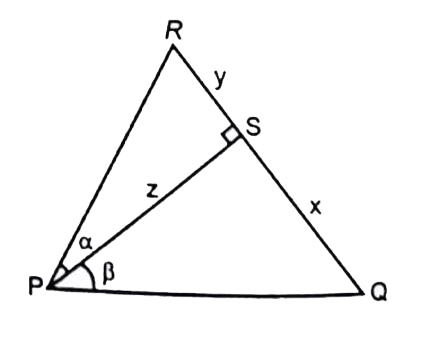 View Solution
View SolutionIf in ΔABC, the distance of the vertices from the orthocenter are x,y, and z then prove that ax+by+cz=abcxyz
View SolutionIn a ΔPQR as show in figure given that x:y:z::2:3:6, then find value of ∠QPR.
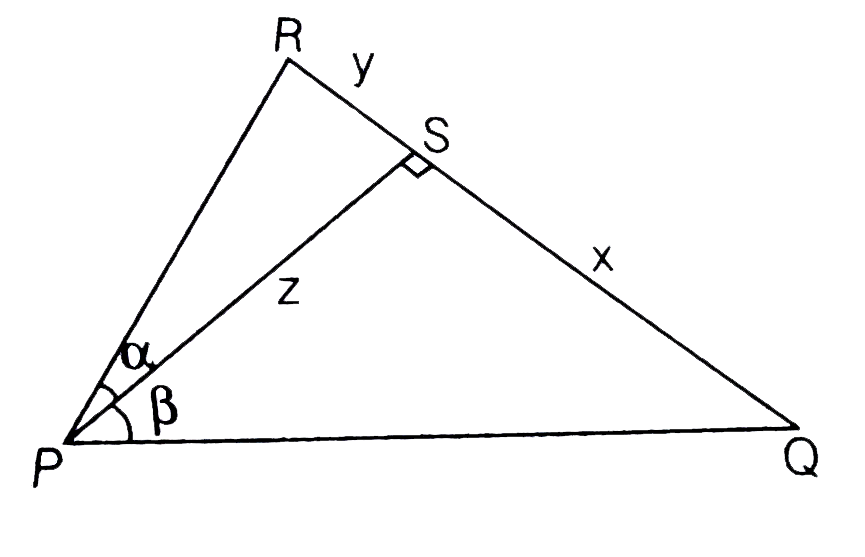 View Solution
View SolutionFind the perimeter of a triangle whose sides are 2y+3z,z−y,4y−2z.
View SolutionLet x,y,z are positive reals and x+y+z=60andx>3.
Maximum value of xyz is :View SolutionFind the coordinates of the centroid of the triangle whose vertices are (x1,y1,z1), (x2,y2,z2)and (x3,y3,z3).
View SolutionIf xyz=1 and x,y,z>0 then the minimum value of the expression (x+2y)(y+2z)(z+2x) is
View Solution


