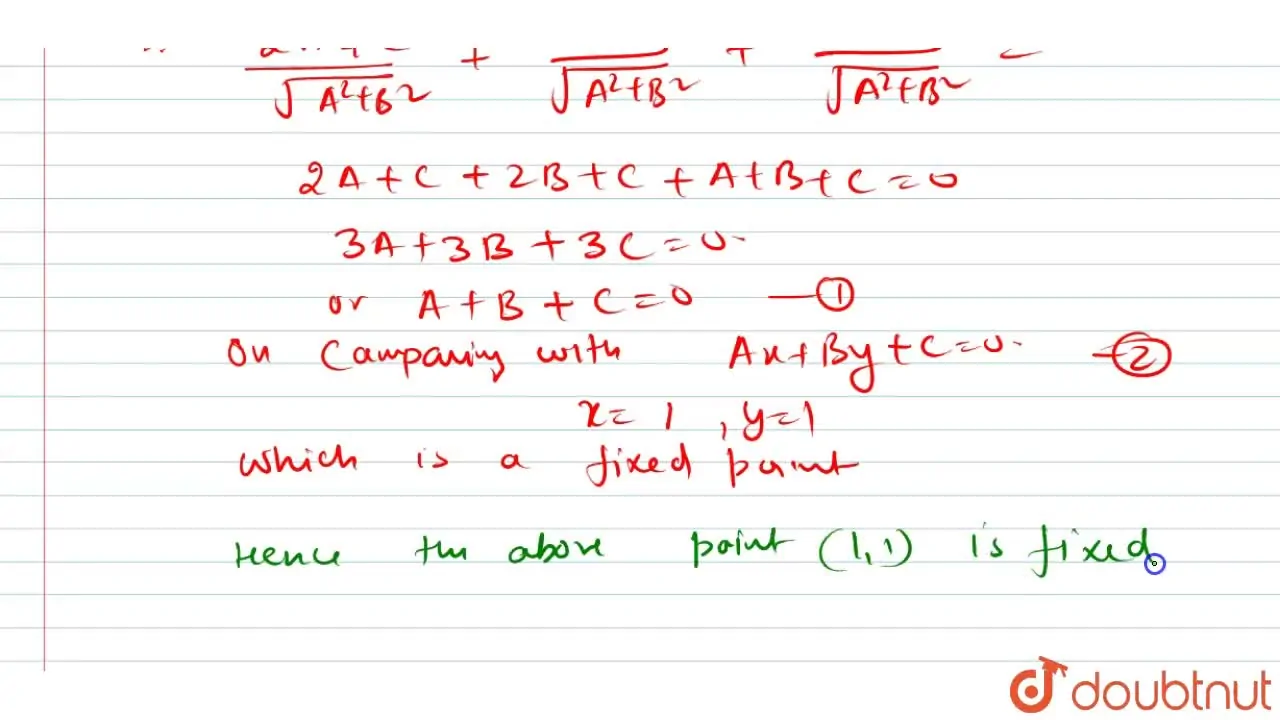
If the algebraic sum of the perpendiculars from the points to a variable line be zero, then prove that line passes through a fixed-point whose coordinates are
Step by step video & image solution for If the algebraic sum of the perpendiculars from the points (2,0),(0,2),(1,1) to a variable line be zero, then prove that line passes through a fixed-point whose coordinates are (1,1)dot by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
Similar Questions
Let the algebraic sum of the perpendicular distance from the points (2, 0), (0,2), and (1, 1) to a variable straight line be zero. Then the line passes through a fixed point whose coordinates are___
View SolutionIf the algebraic sum of perpendiculars from n given points on a variable straight line is zero then prove that the variable straight line passes through a fixed point
View SolutionIf the algebraic sum of the distances of a variable line from the points (2,0),(0,2), and (−2,−2) is zero, then the line passes through the fixed point. (a)(−1,−1) (b) (0,0) (c)(1,1) (d) (2,2)
View SolutionThe algebraic sum of the perpendicular distances from A(x1,y1), B(x2,y2) and C(x3,y3) to a variable line is zero. Then the line passes through (A) the orthocentre of △ABC (B) centroid of △ABC (C) incentre of △ABC (D) circumcentre of △ABC
View SolutionIf the product of the perpendiculars drawn from the point (1,1) on the lines ax2+2hxy+by2=0 is 1, then
View SolutionTangents are drawn from the points on the line x−y−5=0 to x2+4y2=4, then all the chords of contact pass through a fixed point, whose coordinate are
View SolutionA variable line passes through a fixed point P. The algebraic sum of the perpendiculars drawn from the points (2,0), (0,2) and (1,1) on the line is zero. Find the coordinate of the point P.
View SolutionA variable line passes through a fixed point P. The algebraic sum of the perpendiculars drawn from the points (2,0), (0,2) and (1,1) on the line is zero. Find the coordinate of the point P.
View SolutionIf a,b and c are in AP, then the straight line ax+by+c=0 will always pass through a fixed point whose coordinates are (a) (1,2) (b) (1,-2) (c) (2,3) (d) (0,0)
View SolutionFind the coordinates of the foot of the perpendicular drawn from the point (1,−2) on the line y=2x+1.
View Solution