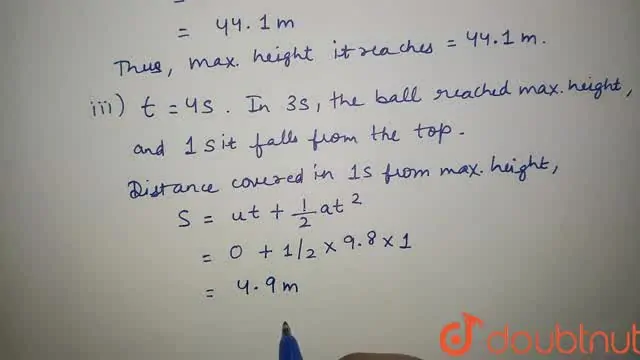A ball thrown up verically returns to the thrower after 6 s. Find
(a) the velocity with which it was thrown up. (b) the maximum height it reaches, and (c ) its position after 4 s.
The correct Answer is:intial velocity =29.4ms−1 height =44.1 m After a s the ball will be at a distance of 4.9 m from the top or 39.2 m from the bottom .
time of ascent is equal to the time of descent , the ball takes a total of 6 s for its upward and downward journey .
Hence , it has taken 3 s to attain the maximum height .
Final velocity of the ball at the maximum height , v=0
Acceleration due to gravity , g=−9.8ms−2
Equation of motion , v=u+ gt will give
0=u+(−9.8×3)
u=9.8×3=29.4vms−1
Hence , the ball was thrown upwards with a velovity of 29.4 ms−1
(b) Let the maximum height attained by the ball be h .
initial velocity during the upward journey , u=29.4 ms−1
final velocity ,v=0
Acceleration due to gravity ,g≡−9.8ms−2
From the equation of motion ,s=ut+1/2at2
h=29.4×3+1/2×9.8×(3)2=44.1m
( c) Ball attains the maximum height after 3 s After attaining this height it will start falling downwards . in this case
initial velocity , u=0
Position of the after 4 s of the thrown is given by the distance travelled by it during its downward journey in 4 s -3s =1s .
Equation of motion , s=ut+1/2>2will give ,
s=0×t+1/2×9.8×12=4.9m
Total height =44.1 m
this means that th ball is 39.2 m (44.1 m-4.9 m) above ground after 4 seconds.
Related Playlists
Similar Questions
A ball thrown vertically upward returns to its starting point in 4 s. Find its initial speed.
View SolutionA ball is thrown vertically upwards from the top of tower of height h with velocity v . The ball strikes the ground after time.
View SolutionA ball is thrown upward with such a velocity v that it returns to the thrower after 3 s. Take g = 10 ms−2. Find the value of v :-
View SolutionA body is thrown vertically up reaches a maximum height of 78.4 m. After what time it will reach the ground from the maximum height reached ?
View SolutionAn object is thrown vertically upward with a speed of 30 m/s. The velocity of the object half-a-second before it reaches the maximum height is
View SolutionIn quick succession, a large number of balls are thrown up vertically in such a way that the next ball is thrown up when the previous ball is at the maximum height. If the maximum height is 5m, then find the number of the balls thrown up per second (g=10 m s^(-2)).
View SolutionA ball is thrown vertically upwards. It returns 6 s later. Calculate the greatest height reached by the ball
View SolutionA ball is thrown upwards, it takes 4 s to reach back to the ground. Find its initial velocity
View SolutionA particle is thrown vertically upwards. If its velocity is half of the maximum height is 20m/s, then maximum height attained by it is
View SolutionA body is throw up with a velocity 'u'. It reaches maximum height 'h'. If its velocity of projection is doubled the maximum height it reaches is _____
View Solution