Let the coordinates of the points A, B, C be (1,8,4), (0,-11,4) and (2,-3,1) respectively. What are the coordinates of the point D which is the foot of the perpendicular from A on BC ?
(3,4,-2)
(4,-2,5)
(4,5,-2)
(2,4,5)
The correct Answer is:C
A(1,8,4),B(0,−11,4),C(2,−3,1)
Let D =(α,β,γ)
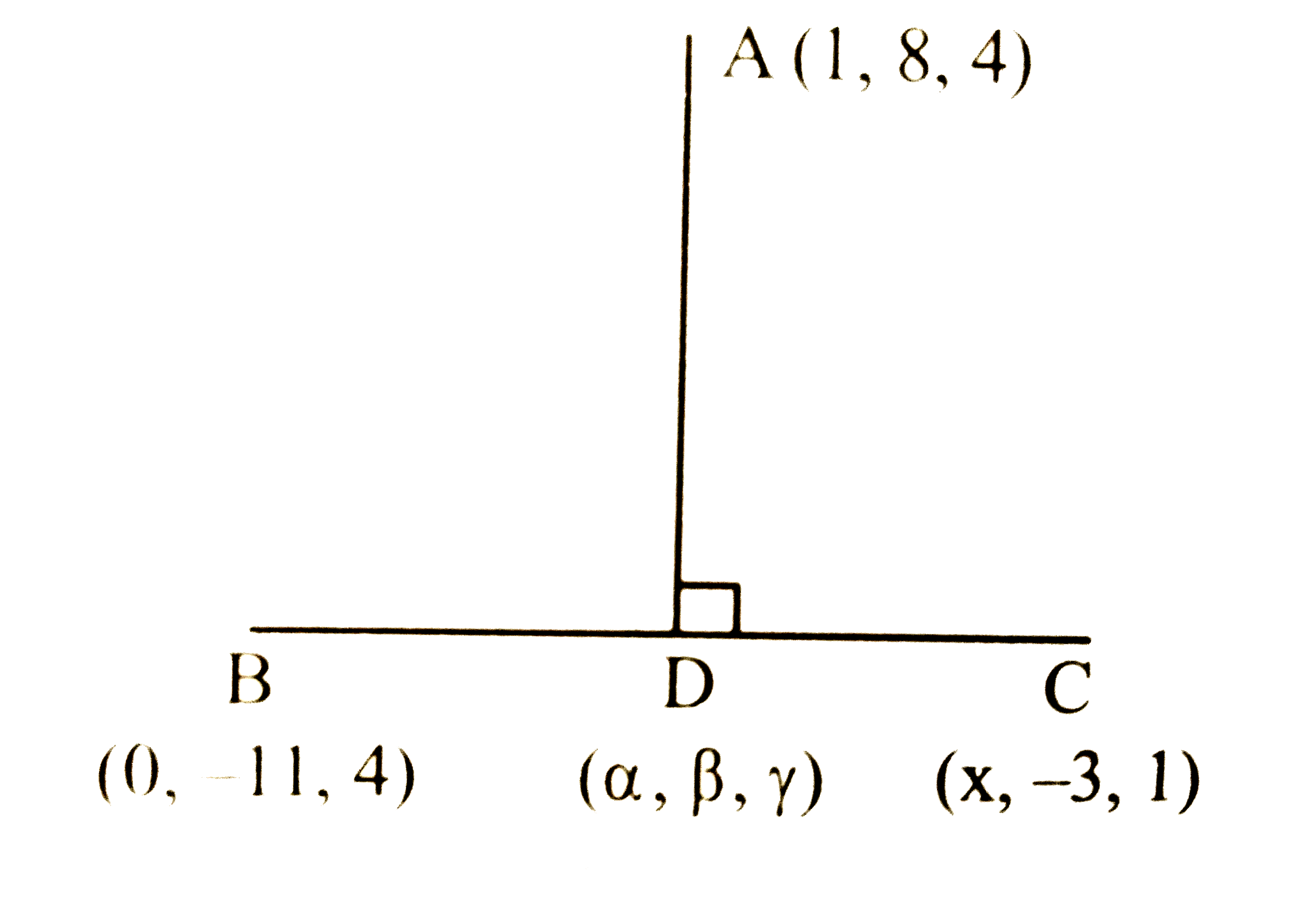
Direction ratios of BC =(x2−x1,y2−y1,z2−z1)
Let, (a, b, c)=(2, 8, -3)
Direction ratios of AD =(x2−x1,y2−y1,z2−z1)
Let (a',b',c')=(α−1,β−8,γ−4)
Since, AD is perpendicular to BC,
aa'+′+'=0
⇒2(α−1)+8(β−8)−3(γ−4)=0
⇒2α−2+8β−64−3γ+12=0...(1)
On substituting the options, we find option (c) is correct.
when (α,β,γ)=(4,5,−2)
(1)⇒2(4)+8(5)−3(−2)−54=0
⇒8+40+6−54=0
⇒0=0
Related Playlists
Similar Questions
If A,B,C are point (1,0,4) , (0,-1,5) and (2,-3,1) respectively , then the coordinates of foot of the perpendicular drawn from A to the line BC are
View SolutionIf the coordinates of the points A,B,C be (−1,5),(0,0) and (2,2) respectively, and D be the middle point of BC, then the equation of the perpendicular drawn from B to the line AD is
View SolutionWrite the coordinates of the foot of the perpendicular from the point (1,2,3) on y-axis.
View SolutionThe coordinate of the foot of the perpendicular from the point (a,b,c) on z-axis is
View SolutionThe coordinates of the mid points of sides AB, BC and CA of ABC are D(1,2,−3), E(3,0,1)and F(−1,1,−4) respectively. Write the coordinates of its centroid.
View SolutionABCD is a parallelogram. If the coordinates of A, B, C are (2,3), (1,4)and (0,−2) respectively, find the coordinates of D.
View SolutionThe coordinates of the points A, B, C, D are (2, a), (3,5), (3,4) and (0, 6) respectively. If the lines AC and BD be perpendicular, then a = ?
View SolutionABCD is parallelogram. If the coordinates of A,B,C are (−2,−1),(3,0) and (1,−2) respectively, find the coordinates of D.
View SolutionWhat are the coordinates of the foot of the perpendicular from the point (2 , 3) on the line x + y - 11 = 0 ?
View SolutionWhat are the coordinates of the foot of the perpendicular drawn from the point (3,5, 4) on the plane z = 0
View Solution


