Question

The length of the diameter of the circle which touches the x-axis at the point (1, 0) and passes through the point (2, 3)

Options

A. `(10)/(3)`
B. `(3)/(5)`
C. `(6)/(5)`
D. `(5)/(3)`

Answer

A

Explanation

Given, a cilcle whichtouches X-axis at the point (1,0), also the circle passes through the point (2.3). Let us assume that the ccordintes of centre of the circle are C(h,k) and its radius is r. since, circle toucjhed x -axis at (1,0), hence its radius should be equal to ordinated of centre.

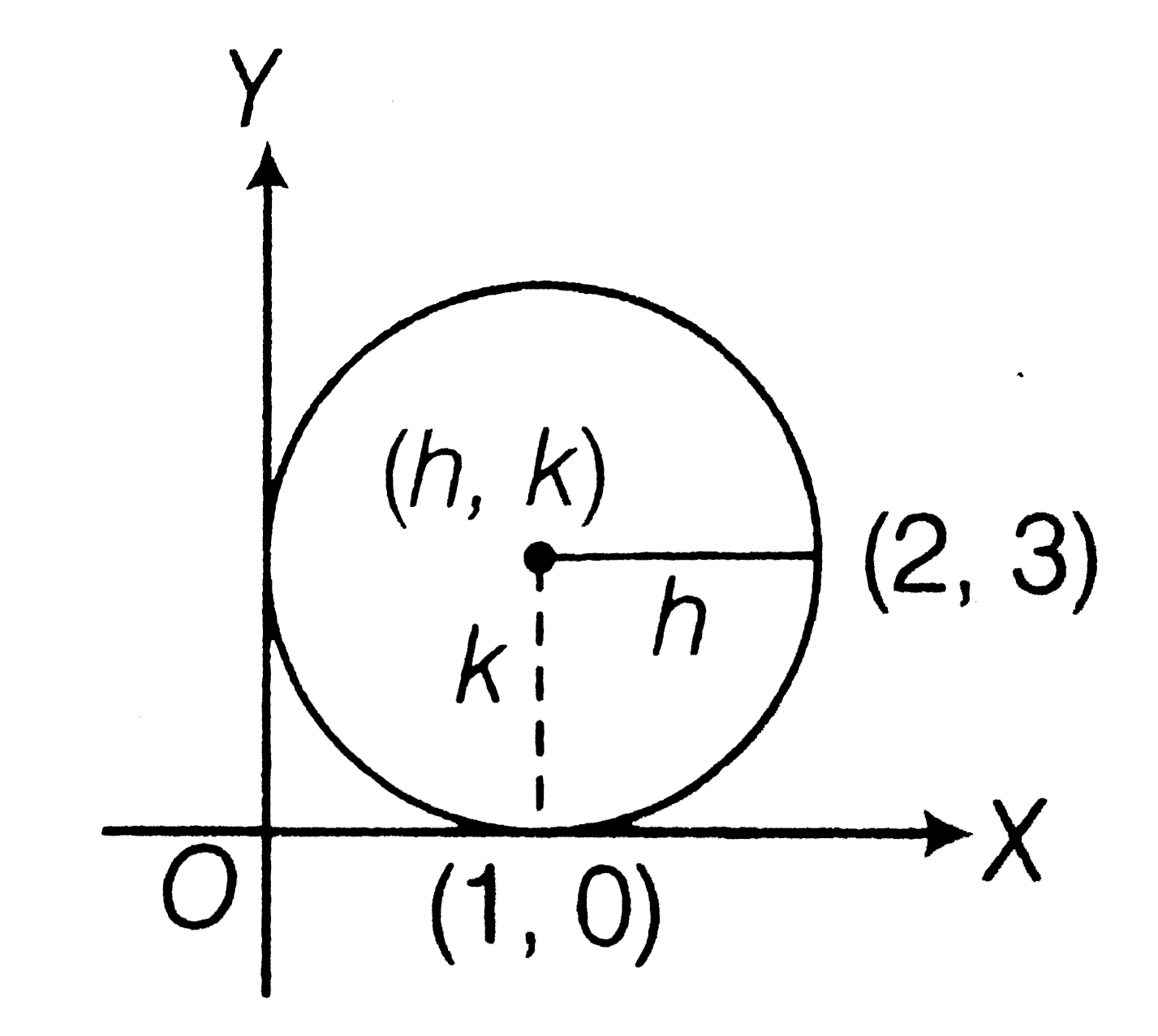
Hence, the equation of the circle is `(x-h)^(2)+(y-k)^(2)=k^(2)` Also, given that circle passes through points (1,0) and (2,0) substituing them in the equation of the circle, we get `(1-h)^(2)+(0-k)^(2)=k^(2)` and `(1-h)^(2)+(3-k)^(2)=k^(2)`
from eq. (i),h=1 on substituing in eq. (ii), we get `(2-1)^(2)+(3-k)^(2)=k^(2)`
`(2-1)^(2)+(3-k)^(2)=k^(2)` so, the diameter of the circle is 2k `=10/3`