A solid metallic right circular cone 20 cm high with vertical angle $60 o$ is cut into two parts at the middle point of its height by a plane parallel to the base. If the frustum, so obtained, be drawn into a wire of diameter $\frac{1}{16} c m$ , find the length of the wire.

Video Solution

Text Solution

Verified by Experts

Let OAB be the cone in which $∠ A O B = 60^{\circ}$ .
Clearly $∠ D O E = 30^{\circ}, O E = 10 c m, O F = 20 c m$ .
Let ED = r cm and FB = R cm.

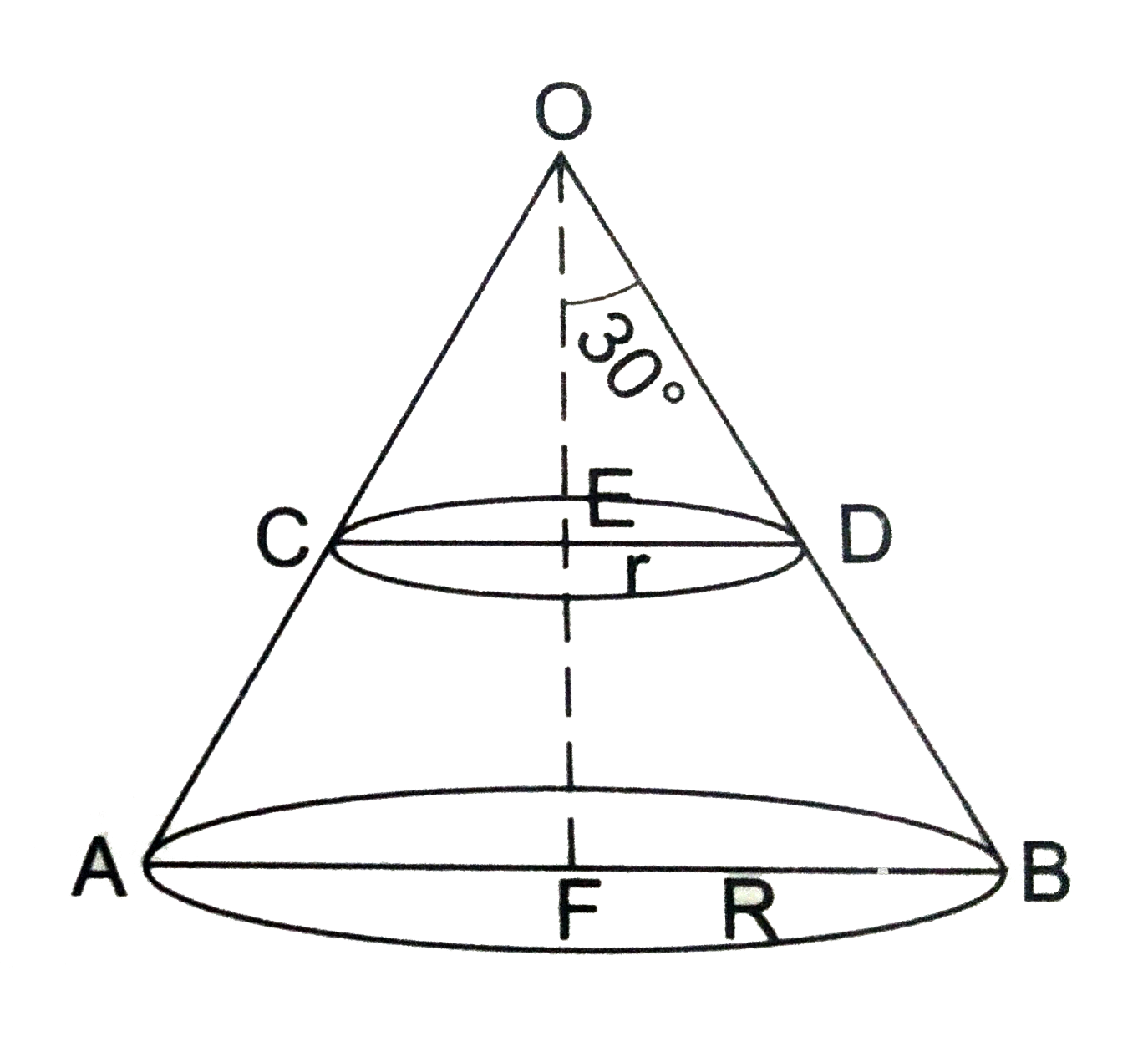
$∴ \frac{E D}{O E} = {tan 30}^{\circ} \Rightarrow \frac{E D}{10} = \frac{1}{\sqrt{3}}$
$\Rightarrow E D = (10 \times \frac{1}{\sqrt{3}}) c m \Rightarrow r = \frac{10}{\sqrt{3}} c m$
$and, \frac{F B}{O F} {tan 30}^{\circ} \Rightarrow \frac{F B}{20} = \frac{1}{\sqrt{3}}$
$\Rightarrow F B = (20 \times \frac{1}{\sqrt{3}}) c m \Rightarrow R = \frac{20}{\sqrt{3}} c m .$
Also, EF = 10 cm.
Thus, ABCD is the frustum of the cone in which
$R = \frac{20}{\sqrt{3}} c m, r = \frac{10}{\sqrt{3}} c m and h = 10 c m .$
$Volume of this frustum = \frac{1}{3} π h (R^{2} + r^{2} + R r)$
$= \frac{1}{3} \times π \times 10 (\frac{400}{3} + \frac{100}{3} + \frac{200}{3}) c m^{3}$
$= (\frac{π \times 10}{3} \times \frac{700}{3}) c m^{3} = (\frac{700 π}{9}) c m^{3}$ .
Let the lenfth of the wire be l.
Radius of the wire, $r_{1} = \frac{1}{32} c m$ .
$Volume of the wire = π r_{1}^{2} = π \times {(\frac{1}{32})}^{2} \times l$ .
$∴ \frac{700 π}{9} = \frac{π l}{32 \times 32} \Rightarrow l = (\frac{700 \times 32 \times 32}{9}) c m$
$\Rightarrow l = (\frac{7000 \times 32 \times 32}{9 \times 100}) m = (\frac{71680}{9}) m$
= 7964.44m.

Updated on:21/07/2023