The height of a cone is 30 cm. A small cone is cut off at the top by a plane parallel to the base. If its volume be $\frac{1}{27}$ of the volume of the given cone, at what height above the base is the section mode?

Video Solution

Text Solution

Verified by Experts

Height of the givne cone = 30 cm
Let the radius of its base be R cm.
$Volume of the given cone = (\frac{1}{3} π R^{2} \times 30) c m^{3} (10 π R^{2}) c m^{3}$ .
Let the radius and height of the smaller cone be r cm and h cm respectively.
Then, $volume of the smaller cone = (\frac{1}{3} π r^{2} h) c m^{3}$ .
$∴ \frac{1}{3} π r^{2} h = \frac{1}{27} (10 π R^{2} r) [given]$

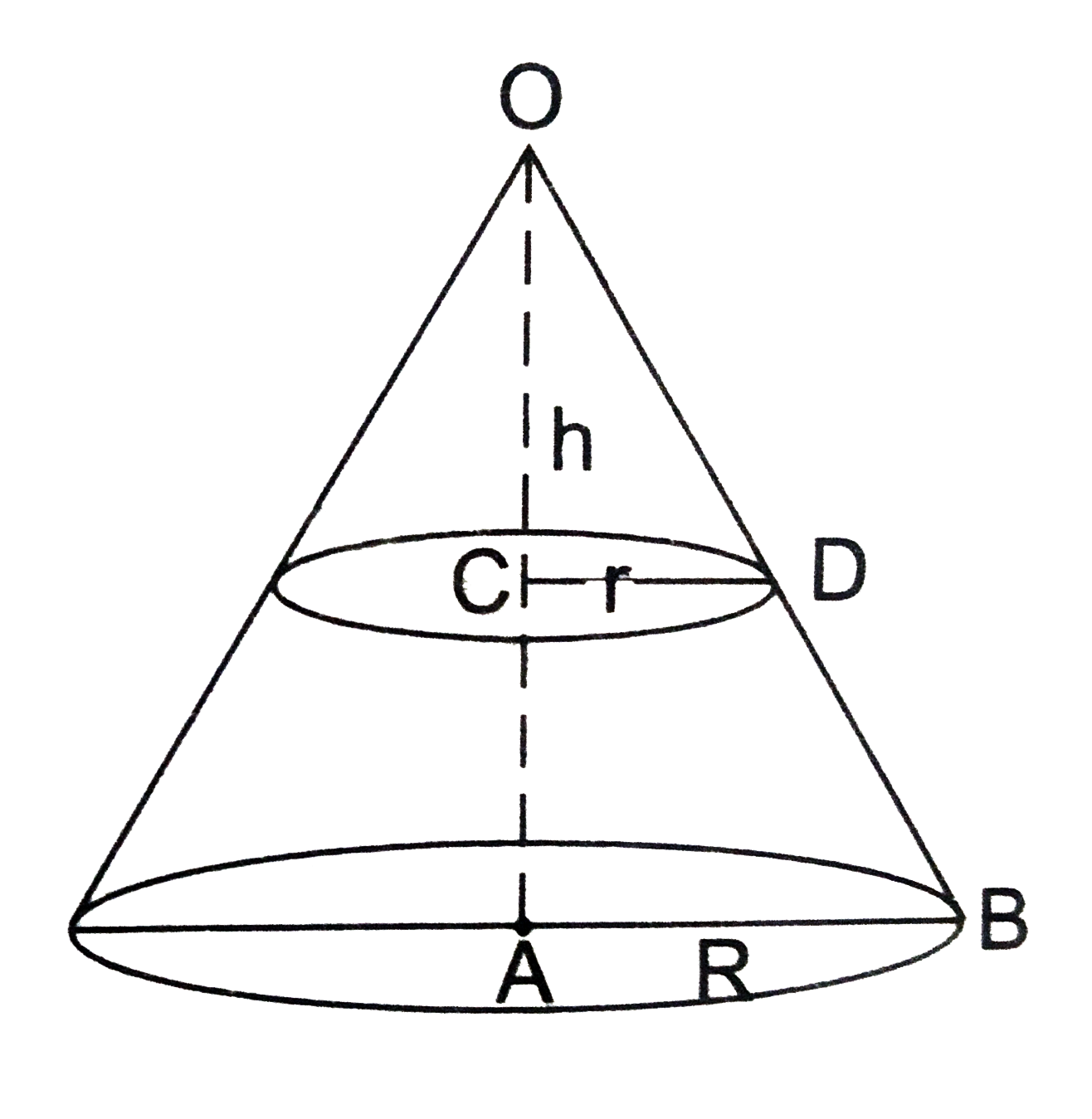
$\Rightarrow {(\frac{R}{r})}^{2} = \frac{9 h}{10} ...(i)$
Now, $Δ O A B ~ Δ O C D$ .
$∴ \frac{A B}{C D} = \frac{O A}{O C} \Rightarrow \frac{R}{r} = \frac{30}{r} ...(ii)$
From (i) and (ii), we get
${(\frac{30}{h})}^{2} = \frac{9 h}{10} \Rightarrow \frac{30 \times 30}{h \times h} = \frac{9 h}{10}$
$\Rightarrow h^{3} = \frac{30 \times 30 \times 10}{9} = 1000 \Rightarrow h^{3} = {(10)}^{3} \Rightarrow h = 10$ .
$∴ height of the smaller cone = 10 cm .$
Height of the section from the base = (30 - 10) cm = 20 cm.

Updated on:21/07/2023

|

Class 10 MATHS SURFACE AREAS AND VOLUMES

Topper's Solved these Questions

Similar Questions

Knowledge Check

The height of a cone is 30 cm .A small cone is cut off at the top by a plane parallel to the base . If its volume be $\frac{1}{27}$ of the volume of the given cone, at what height above the base the section has been made?
A20 cm
B18 cm
C27 cm
D15 cm

RS AGGARWAL-VOLUME AND SURFACE AREAS OF SOLIDS-MULTIPLE-CHOICE QUESTIONS(MCQ)

Home
Ask Doubt
Profile