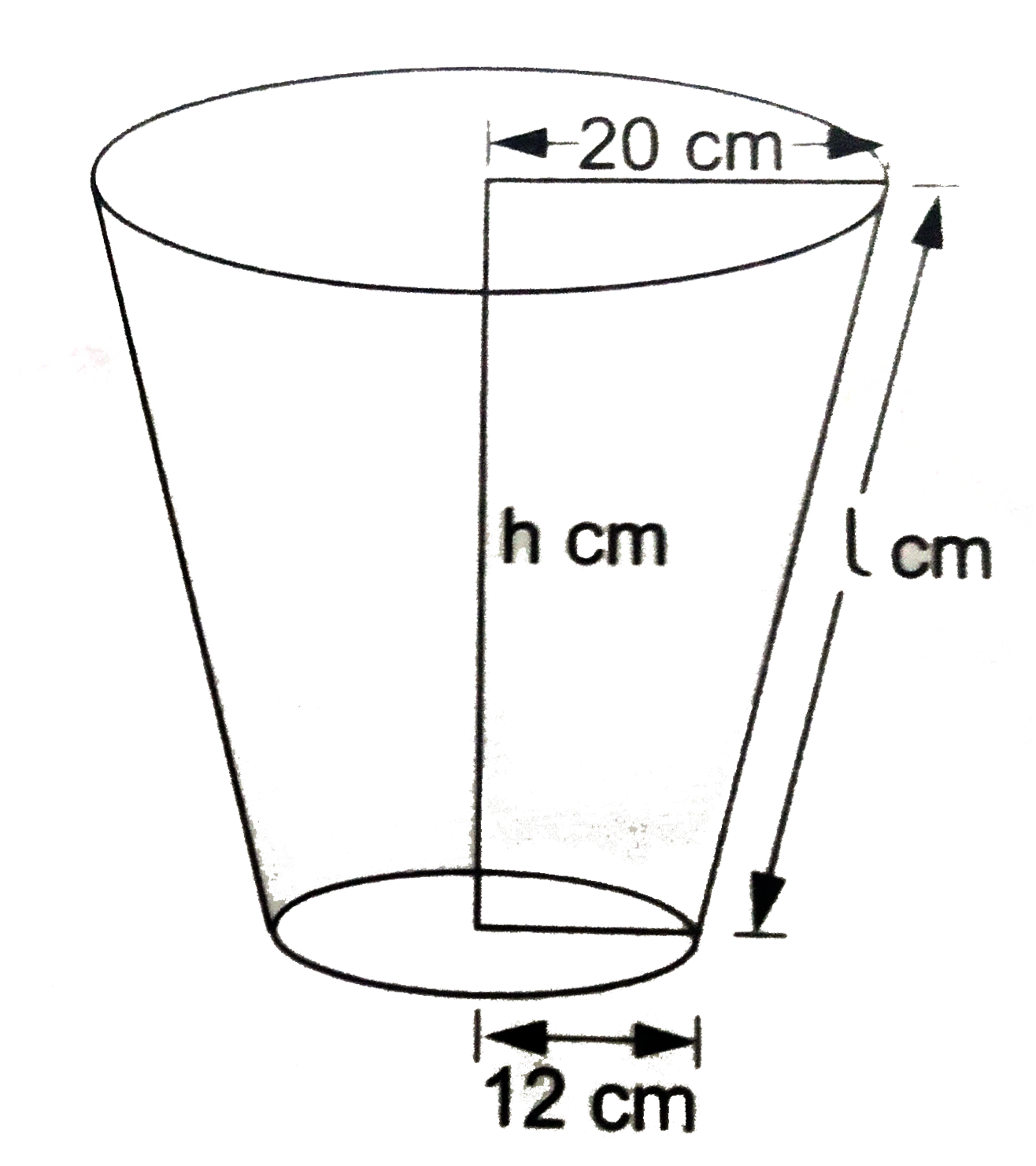
A bucket is in form of a frustum of a cone with a copacity of $12308.8 c m^{3}$ of water. The radii of the top and bottom circular ends are 20 cm and 12 cm respectively. Find the height of the bucket and the area of the metal sheet used in its making. [ Use $π = 3.14 .$ ]

Video Solution

Text Solution

Verified by Experts

Here, R = 20 cm, r = 12 cm
and volume = 12308.8 $c m^{2}$
Let the height of the bucket be h cm.
Volume of the bucket
= volume of frustum of the cone.
$∴ \frac{1}{3} π h (R^{2} + r^{2} + R r) = 12308.8$
$\Rightarrow \frac{1}{3} \times 3.14 h [{(20)}^{2} + 20 \times 12] = 12308.8$
$\Rightarrow 784 h = \frac{12308.8 \times 3}{3.14}$
$\Rightarrow h = (\frac{12308.8 \times 3}{3.14 \times 784}) = 15$ .
Slant height of the bucket
$= \sqrt{h^{2} + {(R - r)}^{2}} units$
$= \sqrt{{(15)}^{2} + {(20 - 12)}^{12}} c m = \sqrt{{(15)}^{2} + 8^{2}} c m$
$= \sqrt{225 + 64} c m = \sqrt{289} c m = 17 c m$ .
Area of the metal sheet used
=(curved surface area) + (area of the bottom)
$= [π l (R + r) \times π r^{2}] sq units$
$= [3.14 \times 17 \times (20 \times 12) + 3.14 \times 12 \times 12] c m^{2}$
$= [3.14 \times (17 \times 32) + 3.14 \times 144] c m^{2}$
$= [3.14 \times (544 + 144)] c m^{2}$
$= (3.14 \times 688) c m^{2} = 2160.32 c m^{2}$ .

Updated on:21/07/2023

|

Class 10 MATHS SURFACE AREAS AND VOLUMES

Topper's Solved these Questions

Similar Questions

Knowledge Check

A bucket is in the form of a frustum of a cone and holds 28.490 litres of water. The radii of the top and bottom are 14 cm and 21 cm respectively. Find the height of the bucket.
A29.2 cm
B10 cm
C20.9 cm
D25 cm

RS AGGARWAL-VOLUME AND SURFACE AREAS OF SOLIDS-MULTIPLE-CHOICE QUESTIONS(MCQ)

Home
Ask Doubt
Profile