Theorem 10.11 : The sum of either pair of opposite angles of a cyclic quadrilateral is 180º.

Video Solution

Text Solution

Verified by Experts

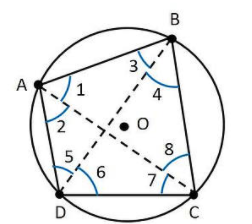
For chord $A B$ , angles in same segment are equal.
$∠ 5 = ∠ 8$
Similarly, in chords $B C$ , $C D$ , and $A D$
$∠ 1 = ∠ 6$
$∠ 2 = ∠ 4$
$∠ 7 = ∠ 3$
Angle sum property of quadrilateral gives
$∠ A + ∠ B + ∠ C + ∠ D = 360$
$∠ 1 + ∠ 2 + ∠ 3 + ∠ 4 + ∠ 5 + ∠ 6 + ∠ 7 + ∠ 8 = 360$
$2 (∠ 1 + ∠ 2 + ∠ 7 + ∠ 8) = 360$
$∠ 1 + ∠ 2 + ∠ 7 + ∠ 8 = 180$
$∠ B A D + ∠ B C D = 180$
Similarly we can prove that,
$∠ A B C + ∠ A D C = 180$

Updated on:21/07/2023