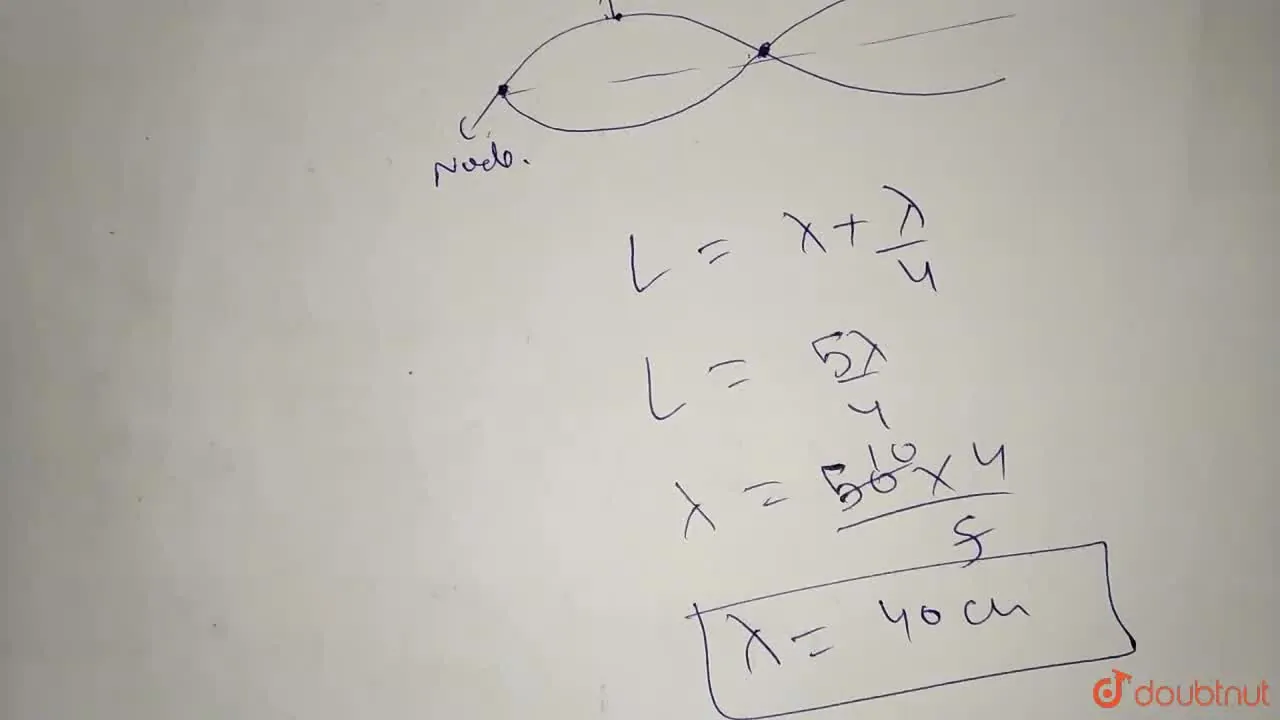Stationary waves in the air column inside a pipe of length 50 cm and closed at one end have three nodes and three antinodes. What is the wavelegth ?
Here L=5λ4
Wavelength = λ=4L5=4×505=40cm
Related Playlists
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SOLVED PROBLEMS - I EXERCISE - KINETIC THEORY OF GASES AND RADIATION26videos
SOLVED PROBLEMS - I
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SOLVED PROBLEMS - I EXERCISE - ASSIGNMENTS24videos
SOLVED PROBLEMS - I
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SOLVED PROBLEMS - I EXERCISE - WAVE MOTION11videos
SOLVED PROBLEMS - I
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SHORT ANSWER QUESTIONSEXERCISE - Assignments3videos
SHORT ANSWER QUESTIONS
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SOLVED PROBLEMS-II EXERCISE - Assignments5videos
SOLVED PROBLEMS-II
Similar Questions
The harmonics formed in air column in an organ pipe closed at one end are
View SolutionAn air column in a pipe of length 1 m and closed at one end, will be in resonace with a vibrating tuning fork of frequency 250 Hz, if the length of the column is
[v = 330 m/s]View SolutionNeglecting and correction , the frequency of Ist overtone of the air column in a pipe of length 25 cm closed at one end (v = 350 m/s in air ) is
View SolutionWhat are forced vibrations and resonance ? Show that only odd harmonics are present in an air column vibrating in a pipe closed at one end. A stretched wire emits a fundamental note of frequency 256 Hz. Keeping the stretching force constant and reducing length of the wire by 10cm, the frequency becomes 320 Hz. Calculate the original length of wire.
View SolutionTwo consecutive harmonics of an air column in a pipe closed at one end are of frequencies 150 Hz and 250 Hz.
What is the fundamental frequency ?View SolutionA pipe of length 10 cm, closed at one end, has a frequency equal to half the 2nd overtone of another pipe open at both the ends. What is the length of the open pipe ?
View SolutionThe frequency of third overtone of a pipe closed at one end , is in unison with the fifth overtone of a pipe open at both the ends. Then the ratio of length of the pipe closed at one end to the open at both the ends is
View SolutionFind the frequency of the third overtone of an air column vibrating in a pipe closed at one end. The length of the pipe is 30cm and the innter diameter of the pipe is 2 cm the speed of sound in air at room temperature is 350 m/s .
View SolutionIn a stationary wave of frequency 200 Hz, the distance between a node and a neighbouring antinode is 0.4m . What the wavelength and velocity of the wave ?
View SolutionIf the air column in a pipe which is closed at one end, is in resonance with a vibrating tuning fork at a frequency 260 Hz, then the length of the air column is
View Solution