A particle in linear SHM has speeds 4 cm/s and 3 cm/s when its displacements from the mean position are 3 cm and 4 cm , respectively. Find its period of oscillation.
To solve the problem, we will follow these steps:
Step 1: Understand the given data
We have two scenarios:
- For the first scenario:
- Speed (v1) = 4 cm/s
- Displacement (x1) = 3 cm
- For the second scenario:
- Speed (v2) = 3 cm/s
- Displacement (x2) = 4 cm
Step 2: Use the formula for velocity in SHM
The velocity in Simple Harmonic Motion (SHM) can be expressed as:
v=ω√A2−x2
Where:
- v is the speed,
- ω is the angular frequency,
- A is the amplitude,
- x is the displacement from the mean position.
Step 3: Set up equations for both scenarios
From the first scenario:
v21=ω2(A2−x21)
42=ω2(A2−32)
16=ω2(A2−9) (Equation 1)
From the second scenario:
v22=ω2(A2−x22)
32=ω2(A2−42)
9=ω2(A2−16) (Equation 2)
Step 4: Divide the two equations
Now, we will divide Equation 1 by Equation 2:
169=A2−9A2−16
Step 5: Cross-multiply and simplify
Cross-multiplying gives:
16(A2−16)=9(A2−9)
Expanding both sides:
16A2−256=9A2−81
Step 6: Rearrange the equation
Rearranging gives:
16A2−9A2=256−81
7A2=175
A2=25
Taking the square root:
A=5 cm
Step 7: Substitute back to find ω
Using Equation 1:
16=ω2(A2−9)
Substituting A2=25:
16=ω2(25−9)
16=ω2(16)
ω2=1
Taking the square root:
ω=1 rad/s
Step 8: Calculate the time period
The time period T is given by:
T=2πω
Substituting ω=1:
T=2π1=2π
Calculating:
T≈6.28 seconds
Final Answer
The period of oscillation is approximately 6.28 seconds.
---
We have two scenarios:
- For the first scenario:
- Speed (v1) = 4 cm/s
- Displacement (x1) = 3 cm
- For the second scenario:
- Speed (v2) = 3 cm/s
- Displacement (x2) = 4 cm
The velocity in Simple Harmonic Motion (SHM) can be expressed as:
v=ω√A2−x2
Where:
- v is the speed,
- ω is the angular frequency,
- A is the amplitude,
- x is the displacement from the mean position.
From the first scenario:
v21=ω2(A2−x21)
42=ω2(A2−32)
16=ω2(A2−9) (Equation 1)
v22=ω2(A2−x22)
32=ω2(A2−42)
9=ω2(A2−16) (Equation 2)
Now, we will divide Equation 1 by Equation 2:
169=A2−9A2−16
Cross-multiplying gives:
16(A2−16)=9(A2−9)
Expanding both sides:
16A2−256=9A2−81
Rearranging gives:
16A2−9A2=256−81
7A2=175
A2=25
Taking the square root:
A=5 cm
Using Equation 1:
16=ω2(A2−9)
Substituting A2=25:
16=ω2(25−9)
16=ω2(16)
ω2=1
Taking the square root:
ω=1 rad/s
The time period T is given by:
T=2πω
Substituting ω=1:
T=2π1=2π
Calculating:
T≈6.28 seconds
The period of oscillation is approximately 6.28 seconds.
Topper's Solved these Questions
- SOLVED PROBLEMS - I Book:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:SOLVED PROBLEMS - I Exercise:ELASTICITY
Explore 26 Videos
- SOLVED PROBLEMS - I Book:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:SOLVED PROBLEMS - I Exercise:WAVE MOTION
Explore 11 Videos
- SOLVED PROBLEMS - I Book:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:SOLVED PROBLEMS - I Exercise:GRAVITATION
Explore 9 Videos
- SHORT ANSWER QUESTIONSBook:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:SHORT ANSWER QUESTIONSExercise:Assignments
Explore 3 Videos
- SOLVED PROBLEMS-II Book:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:SOLVED PROBLEMS-II Exercise:Assignments
Explore 5 Videos
Similar Questions
A body executing S.H.M. has its velocity 10cm/s and 7cm/s when its displacement from the mean positions are 3cm and 4cm respectively. Calculate the length of the path.
View SolutionA boby excuting SHM has its velocity its 10cm/seeand7cm/see when its displacement from themean positionare 3cm and 4cm , respectively. Calculate the length of the path.
View Solution
Knowledge Check
The velocities of a body executing S.H.M. are 3 cm/s and 4 cm/s when the displacements from the mean position are 4 cm and 3 cm, then the period of oscillation is
A12πsBx2sC2xsD2πsA body executes SHM has its velocity 10cm/s and 7cm/s when its displacements from mean positions are 3cm and 4cm respectively. The length of path is nearly
A10cmB9.5cmC4cmD11.36cmTwo blocks of masses 3kg block is attached to a spring with a force constant, kk=900N/ma which is compressed 2m initially from its equilibrium position. When 3kg mass is released, it strikes the 6kg mass and the two stick togther in an inelastic collision.
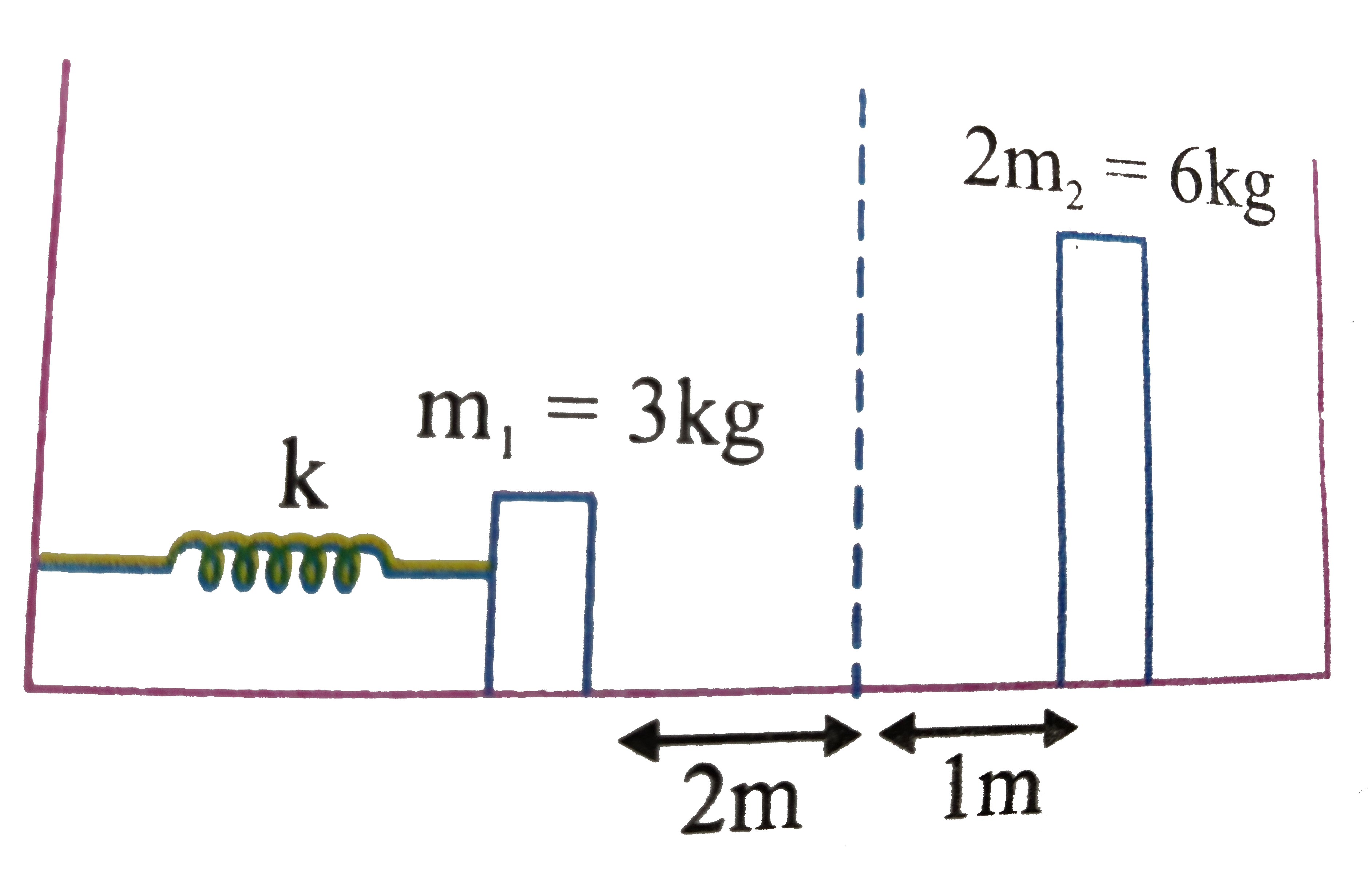
The velocities of a particle executing S.H.M. are 30cm/s and 16cm/s when its displacements are 8cm and 15cm from the equilibrium position. then its amplitude of oscillation in cm is:A25B21C17D13
A particle performing S.H.M. has velocities of 8 cm/s and 6 cm/s at displacements of 3 cm and 4 cm respectively. Calculate the amplitude and period of S.H.M.
View SolutionA particle of mass 10 g is performing SHM. Its kinetic energies are 4.7 J and 4.6 J when the displacements are 4 cm and 6 cm , respectively. Compute the period of oscillation.
View SolutionAcceleration of a particle in SHM at displacement x=10cm (from the mean position is a=−2.5cm/s2). Find time period of oscillations.
View SolutionA body of mass 0.1 kg performing linear SHM experiences a restoring force of 1 N when its displacement from the mean position is 5 cm. Find (i) the force constant (ii) the period of SHM (iii) the acceleration of the body, when its displacement from the mean position is 1 cm./
View SolutionA particle moving with S.H.M. has velocitiesof 4cm/s and 3cm/s at distances of 3 cm and 4 cm respectively from the equilibrium position. Find
(a)The amplitude of the osciallation
(b) Time period
(c ) The velocity of the particle as is pases through the equilibrium position.
View Solution
