For a given integer k in the interval [2πk−π2,2πk+π2] the graph of sinx is
A
increasing from -1 to 1
B
decreasing from -1 to 0
C
decreasing from 0 to 1
D
None of these
Video Solution
Text Solution
The correct Answer is:A
Since 2πk−π2<sinx≤2πk+π2
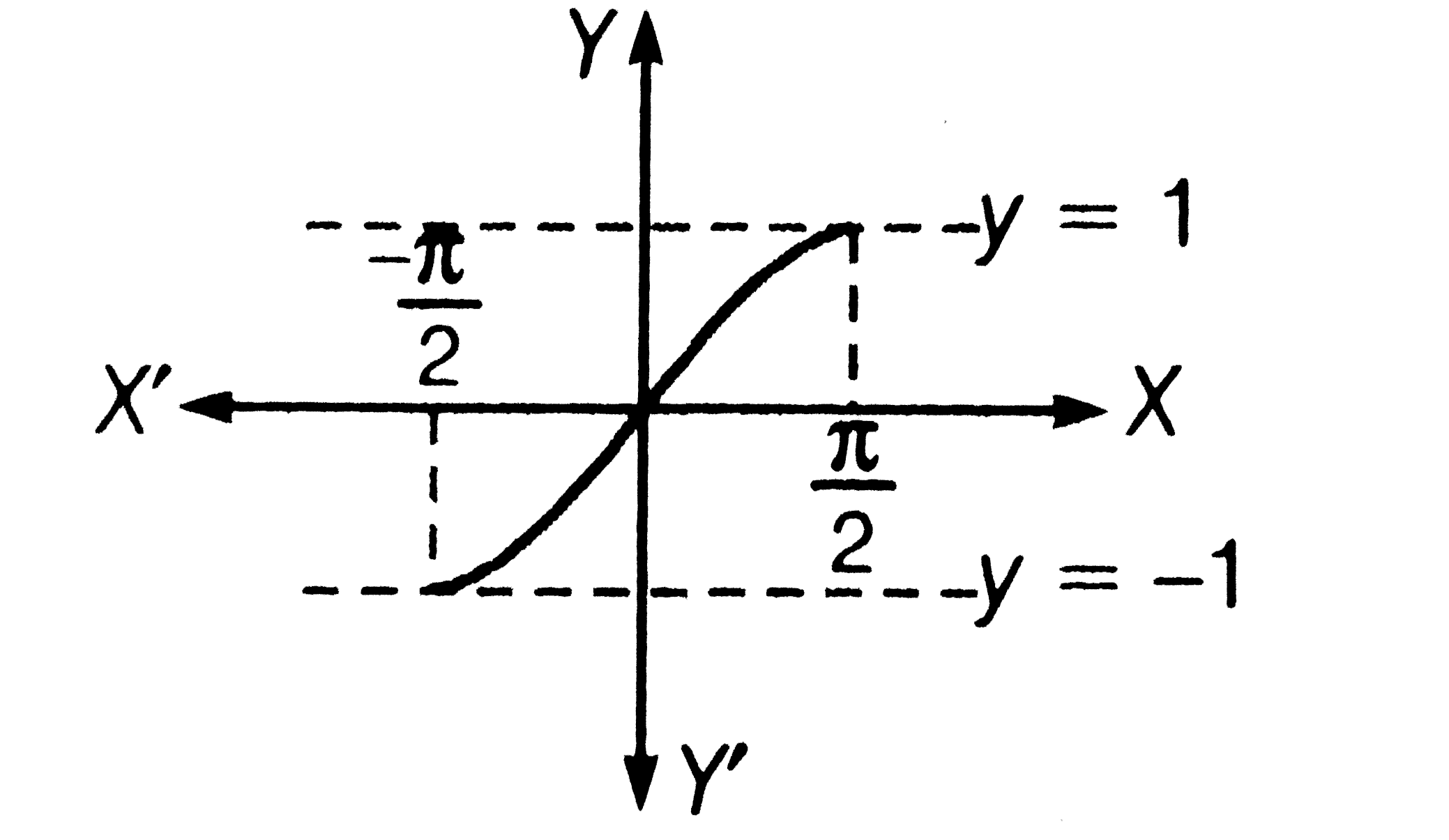
For k=0,−π2<sinx<π2
which increases from -1 to 1.
Similarly, for other values of k it is increases from -1 to 1.
|
Topper's Solved these Questions
- APPLICATIONS OF DERIVATIVESBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - MISCELLANEOUS PROBLEMS
80 Videos
- APPLICATIONS OF DERIVATIVESBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - MHT CET CORNER
21 Videos
- APPLICATIONS OF DEFINITE INTEGRALS BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - APPLICATIONS OF DEFINITE INTEGRALS EXERCISE - MHT CET Corner
6 Videos
- BINOMIAL DISTRIBUTIONBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - BINOMIAL DISTRIBUTIONEXERCISE - MHT CET Corner
6 Videos
Similar Questions
Knowledge Check
On the interval (0,π2) the function logsinx is
AincreasingBdecreasingCneither increasing nor decreasingDNone of the abovewhich of the following statement is/are true ?
(i) f(x) =sin x is increasing in interval [−π2,π2]
(ii) f(x) = sin x is increasing at all point of the interval [−π2,π2]
(3) f(x) = sin x is increasing in interval (−π2,π2)UU(3π2,5π2)
(4) f(x)=sin x is increasing at all point of the interval (−π2,π2)UU(3π2,5π2)
(5) f(x) = sin x is increasing in intervals [−π2,π2]&[3π2,5π2]Aall are correctBall are falseC(3) and (4) are correctD(1),(4) & (5) are correctThe number of solution of sinx+sin3x+sin5x=0 in the interval [π2,3π2] is
A2B3C4D5
If −π2<θ<π2 then acosθ+bsecθ≥K then K=
View SolutionIf k is an integer, evaluate ∫π0sin2kxsinxdx
View SolutionIf n is a positive integer and (1+i)2n=kcos(nπ2), then the value of k is
View SolutionVerify Rolle's theorem for the following functions in the given intervals.
f(x) = sinx + cos x in the interval [0,π/2].
View SolutionIf sinx+x≥|k|x2,∀x∈[0,π2], then the greatest value of k is
View Solution
