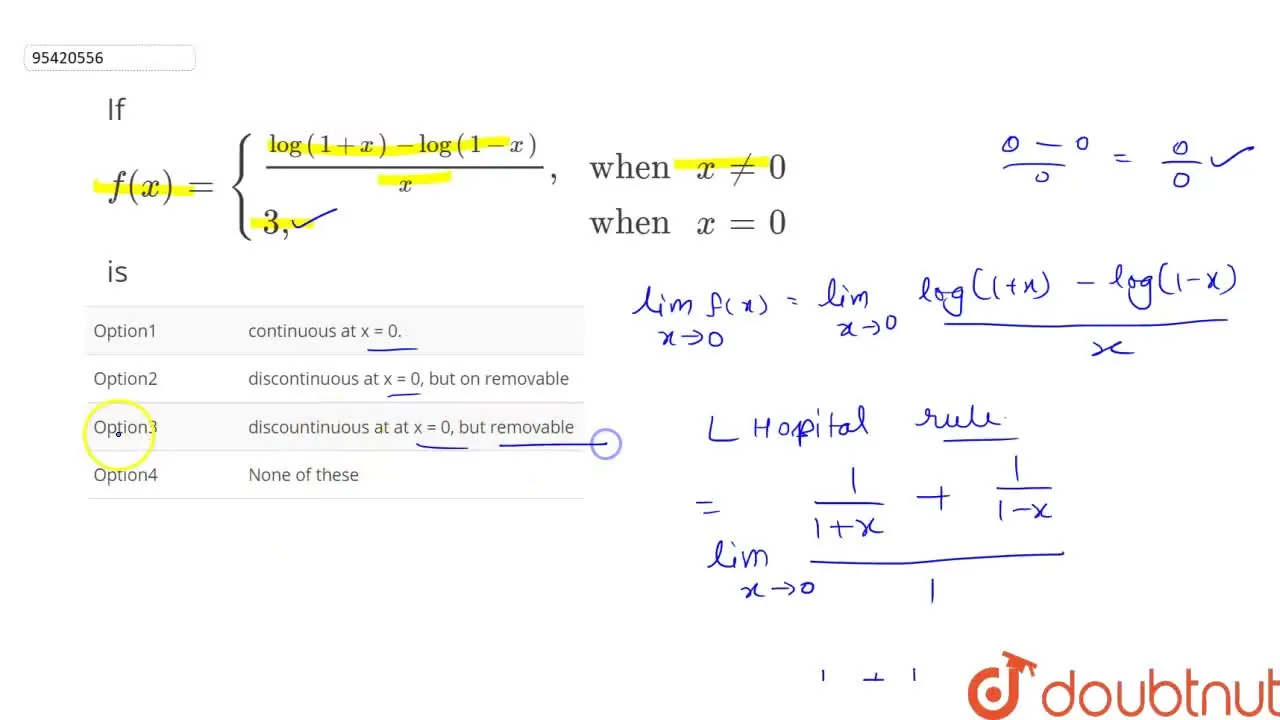
If f(x)={log(1+x)−log(1−x)x,when x≠03,when x=0 is
continuous at x = 0.
discontinuous at x = 0, but on removable
discountinuous at at x = 0, but removable
None of these
The correct Answer is:C
Given , f(x)={log(1+x)−log(1−x)x,x≠03,x=0
Now, limx→0f(x)=limx→0log(1+x)−log(1−x)x
=limx→0=11+x+11−x1=limx→011+111=2
andf(0)=3
∴limx→0f(x)≠f(0)
Hence, f(x) is discontinuous at x = 0 and removable .
Related Playlists
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - CONTINUITYEXERCISE - EXERCISE 2 (MISCELLANEOUS PROBLEMS)60videos
CONTINUITY
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - CONTINUITYEXERCISE - MHT CET Corner16videos
CONTINUITY
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - CIRCLE AND CONICSEXERCISE - All Questions74videos
CIRCLE AND CONICS
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - DEFINITE INTEGRALSEXERCISE - MHT CET Corner22videos
DEFINITE INTEGRALS
Similar Questions
If f(x)={log(1+2ax)−log(1−bx)x,x≠0k,x=0 is continuous at x = 0, then value of k is
View Solutionf(x)={log(1+2ax)−log(1−bx)x,x≠0x=0
View SolutionIf f(x)={log(1+2ax)−log(1−bx)x,x≠0 k,x=0 is continuous at x = 0, then k is equal to
View SolutionIf f(x) =log(1−2x)−log(1−3x)x, x≠0=a, x=0 is continuous at x = 0 , then : a =
View SolutionIf f(x) =log(1+3x)−log(1−2x)x, if x≠0=a, if x=0 is continuous at x = 0 , then a =
View SolutionFor what value of k, the function defined by
f(x)={log(1+2x)sinx∘x2,for x≠0k,for x=0
is continuous at x = 0 ?View SolutionShow that f(x)={x sin1x,whenx≠00,whenx=0 is continuous but not differentiable at x = 0
View Solutionf(x)={sin3xx,whenx≠01,whenx=0 is discontinuous atx =0.
View SolutionIf f(x)=⎧⎨⎩xlogcosxlog(1+x2),x≠00,x=0 then f(x) is
View SolutionIf f(x)={log(1−3x)(1+3x),forx≠0k,forx=0 is continuous at x = 0, then k is equal to
View Solution