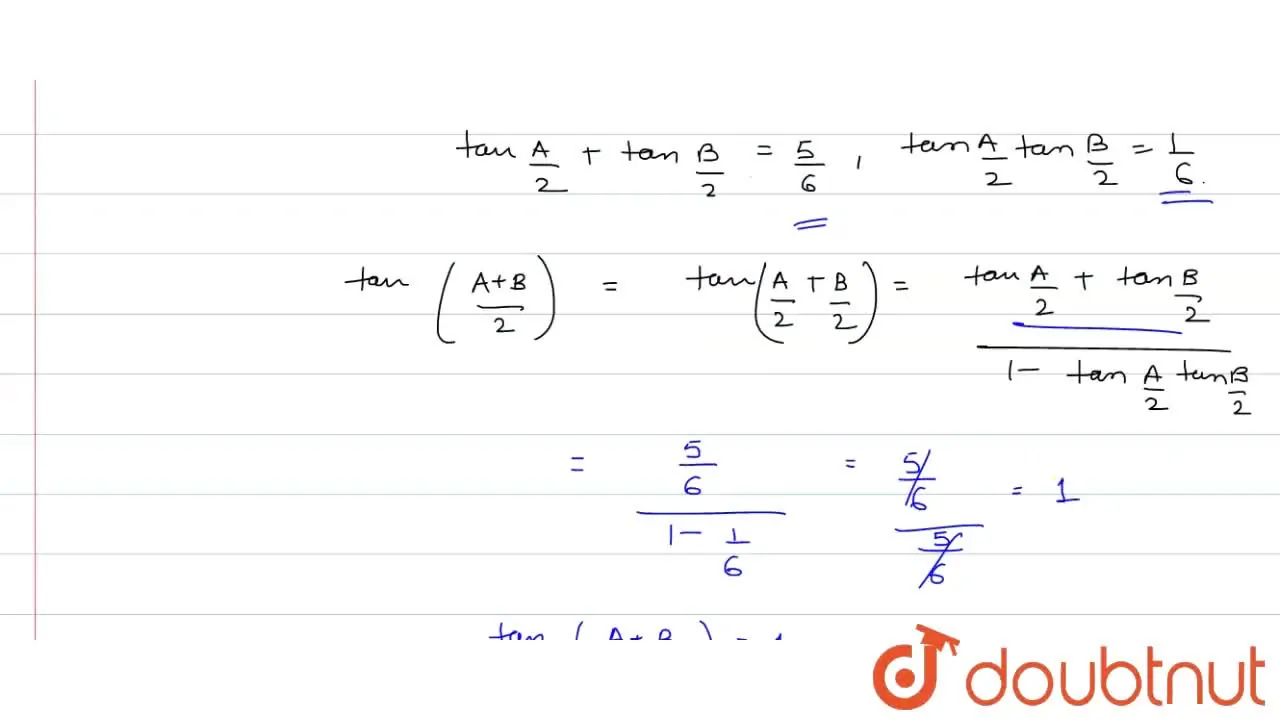
In a $Δ A B C, tan \frac{A}{2} and tan \frac{B}{2}$ , satisfy $6 x^{2} - 5 x + 1 = 0$ . Then ,

A

$a^{2} + b^{2} > c^{2}$

B

$a^{2} - b^{2} = c^{2}$

C

$a^{2} + b^{2} = c^{2}$

D

None of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

$tan \frac{A}{2} + tan \frac{B}{2} = \frac{5}{6}, tan \frac{A}{2} \cdot tan \frac{B}{2} = \frac{1}{6}$
$\Rightarrow tan (\frac{A + B}{2}) = \frac{tan \frac{A}{2} + tan \frac{B}{2}}{1 - tan \frac{A}{2} tan \frac{B}{2}} = 1$
$\Rightarrow A + B = 2 \times \frac{π}{4}$
$\Rightarrow Δ$ is right angled at $∠ C$ .
$∴ c^{2} = a^{2} + b^{2}$

|

Updated on:21/07/2023

Class 12 MATHS TRIGONOMETRIC FUNCTIONS

Similar Questions

Knowledge Check

Question 1 - Select One
In $Δ A B C, tan . \frac{A}{2} + tan . \frac{B}{2}$ =
A $\frac{c cot . \frac{C}{2}}{4 s}$
B $\frac{2 c cot . \frac{C}{2}}{a + b + c}$
C $\frac{2 c tan . \frac{C}{2}}{s}$
D $\frac{c tan . \frac{C}{2}}{a + b + c}$
Question 2 - Select One
In $Δ A B C, 1 - tan . \frac{A}{2} tan . \frac{B}{2}$ =
A $\frac{2 c}{a + b + c}$
B $\frac{a}{a + b + c}$
C $\frac{2}{a + b + c}$
D $\frac{4 a}{a + b + c}$
Question 3 - Select One
In $Δ A B C', (a + b + c)$ ("tan" A/2+"tan" B/2) is equal to
A $2 c cot \frac{C}{2}$
B $2 a cot \frac{A}{2}$
C $2 b cot \frac{B}{2}$
D $tan \frac{C}{2}$

Recommended Questions

Home
Ask Doubt
Profile