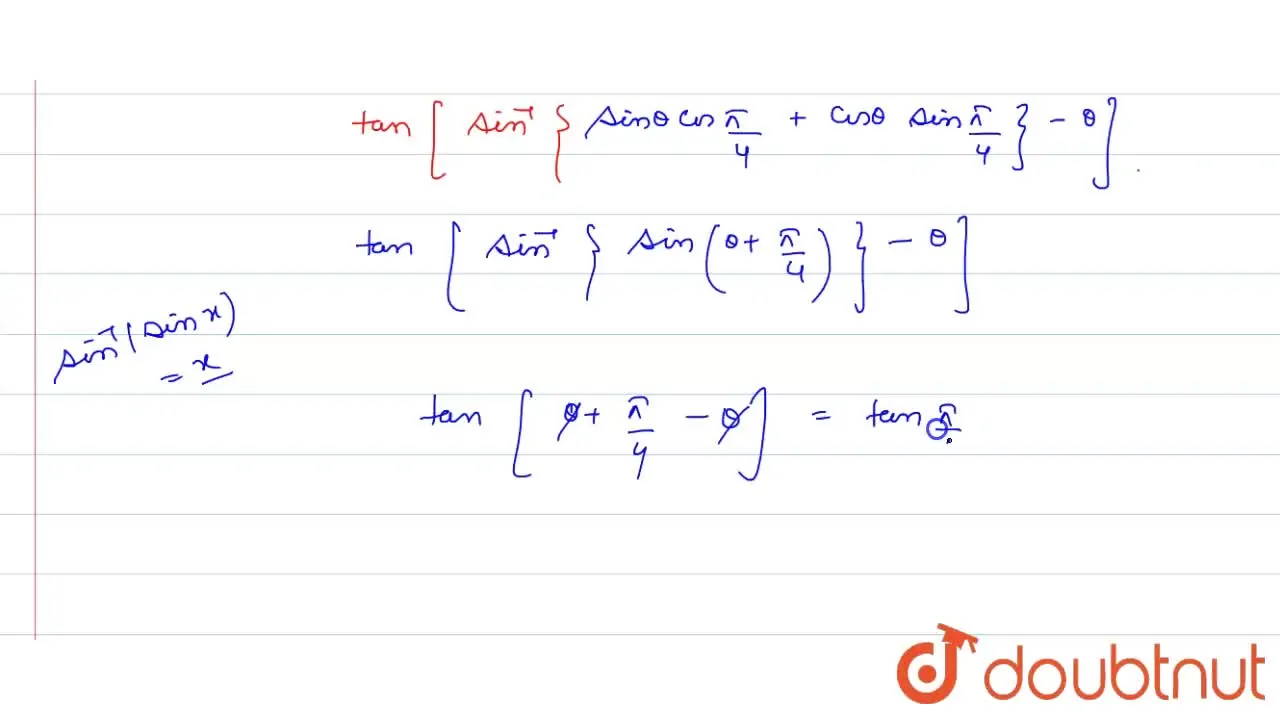
Given , , then the value of is
A
1
B
C
D
Video Solution
Text Solution
The correct Answer is:A
Given , for ,
|
Updated on:21/07/2023
Similar Questions
Given , 0≤x≤12, then the value of tan[sin−1{x√2+√1−x2√2}−sin−1x] is
View SolutionGiven, 0≤x≤12, then the value of tan[sin−1{x√2+√1−x2√2}−sin−1x] is
View SolutionIf x∈[√32,1] then [sin−1{x√2+√1−x2√2}−sin−1x]=
View Solutionif x greater than equal to 0 and less than equal to 1/2 then find the value of tan[sin−1{x√2+√1−x22}−sin−1x]
View SolutionThe value of sin−1[x√1−x−√x√1−x2] is equal to
View SolutionGiven that 0≤x≤12, and A=tan(sin−1(x√2+√1−x22)−sin−1x) .If B=tan(4tan−1(15)−tan−1(1239)) ,then find the value of A and B
View SolutionIf x∈(π,3π2) then the value of tan−1(√1−sinx+√1+sinx√1−sinx−√1+sinx)
View SolutionProve that tan−1{xa+√a2−x2}=12sin−1xa,−a<x<a
View SolutionFind the value of cot−1[√1−sinx+√1+sinx√1−sinx−√1+sinx]
View SolutionFind the value of limx→0√2−√1+cosxsin2x
View Solution