The equation to the line bisecting the join of (3,-4) and (5,2) and having intercepts on the x-axis and y-axis in the ratio of 2:1 is:

A

x+y-3=0

B

2x-y=9

C

x+2y=2

D

2x+y=7

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:C

Let the points be A (3,-4) and B(5,2)
Mid - point of AB = (4,-1)
It is given that the bisecting line intersect the coordinate axes in the ratio 2 :1 .
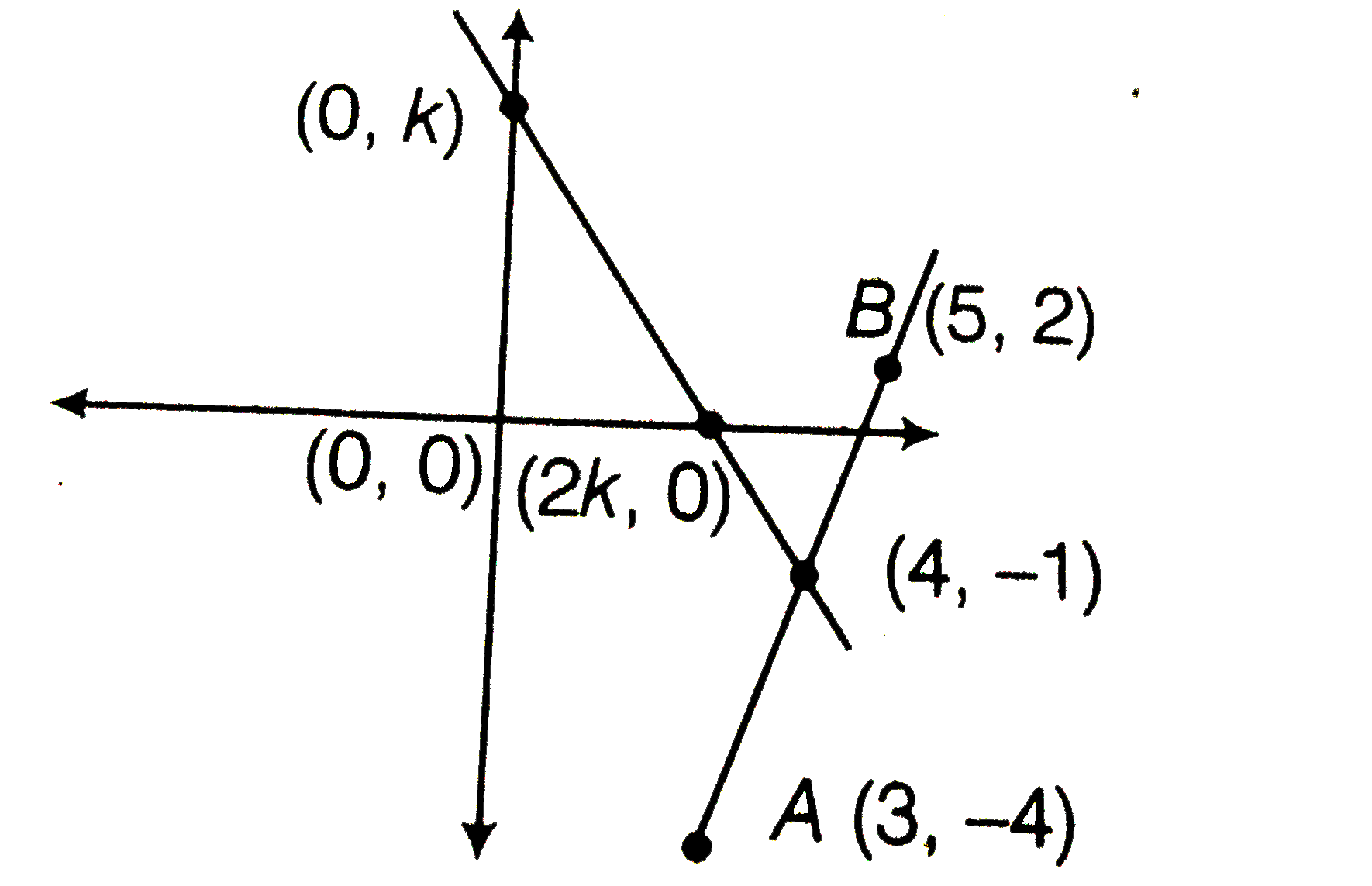
ocr_image
So, point on coordinate axes are (2k, 0) and (0,k) . The equation of line passing through the above point is
y−0=k−00−2k(x−2k)⇒y=−12(x−2k)
Since it is passing through the mid-point of AB.i.e. (4,-1)
−1=−12(4−2k)⇒k=1
On putting the value of k in Eq. (i) we get ,
y=−12(x−2)⇒x+2y=2

Updated on:21/07/2023
|
Class 12MATHSSTRAIGHT LINE

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • The equation of the line bisecting the join of (3,-4) and (5,2) and having its intercepts on the X-axis and the Y-axis in the ratio 2:1 is

    Ax+y-3=0
    B2x-y=9
    Cx+2y=2
    D2x+y=7

  • The equation to the line bisecting the joining of (3. - 4) and (5, 2) having its intercepts on X-axis and Y-axis in the ratio 2:1, is

    Ax + y - 3= 0
    B2x - y = 9
    Cx + 2y = 2
    D2x + y = 7

  • The equation of a line bisecting the join of (2010, 1600) and (−1340,1080) and having intercept on the axes in the ratio 1 : 2 is

    A2x+y=1680
    Bx+2y=1680
    C2x+y=2010
    Dnone of these