The area of the region bounded by the parabola (y−2)2=x−1, the tangent to the parabola at the point (2,3) and the x-axis is
2:1
3:1
3:2
None of these
The correct Answer is:A
Parabola (y−2)2=x−1
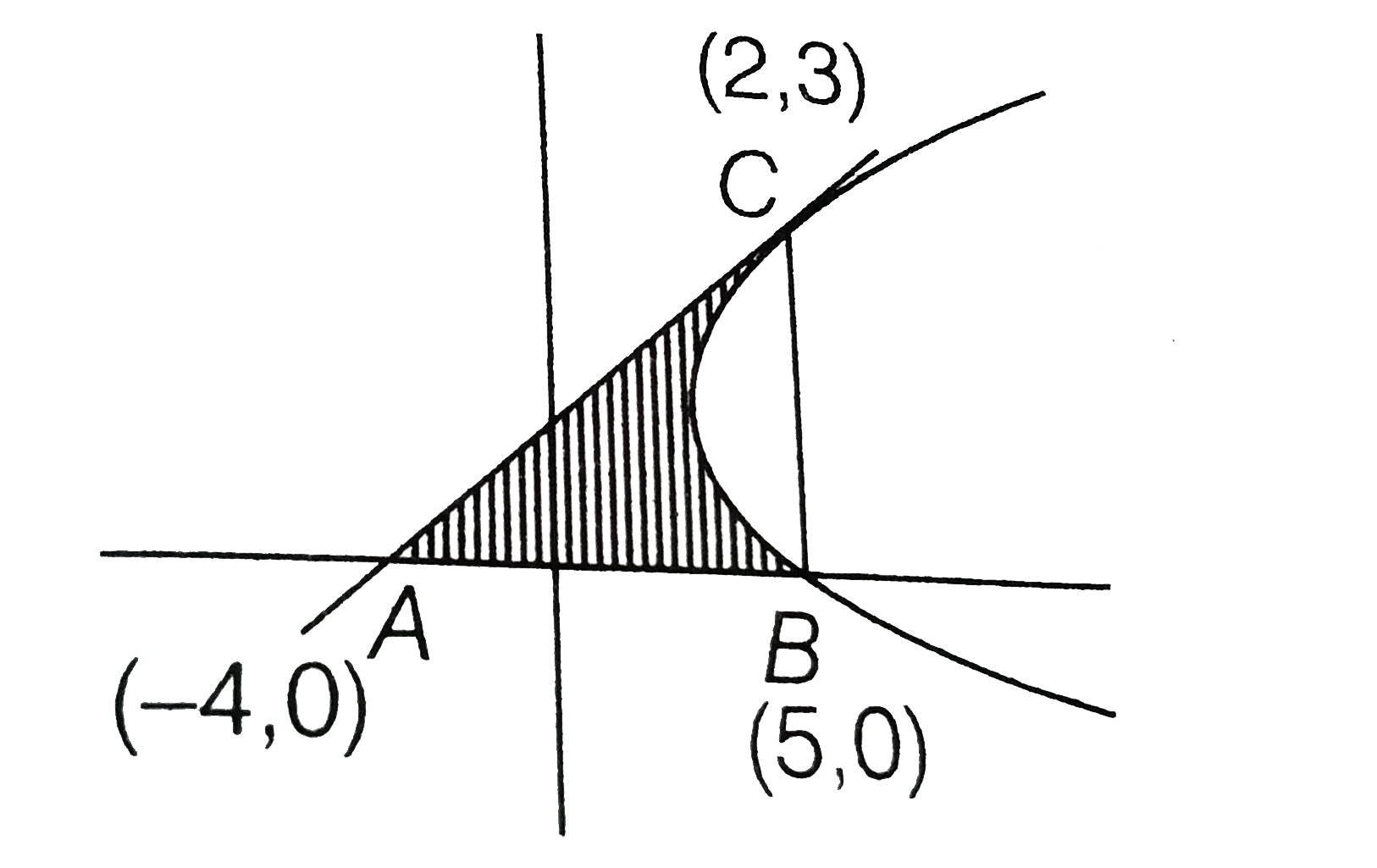
It meets the X-axis at B(5,0)
Tangent to the parabola.
at C(2,3) is x=2y−4
meets the X-axis at (−4,0).
Area of ΔABC=12∣∣
∣∣231−401501∣∣
∣∣=272
Area fo the shaded region
=∫30[(y−2)2+1−2y+4]dy
=[(y−2)33+5y−y2]30
=13+15−9−86=9
∴ Required ratio =9:(272−9)
=2:1
Topper's Solved these Questions
Similar Questions
The area of the region bounded by the parabola (y2)2=x1 , the tangent to the parabola at the point (2, 3) and the xaxis is (1) 3 (2) 6 (3) 9 (4) 12
View SolutionThe area of the region bounded by the parabola (y−2)2=(x−1), the tangent to it at the point whose ordinate is 3 and the x-axis is :
View Solution
Knowledge Check
The area of the region bounded by the parabola y2=9x and the line y=3x is :
A12 sq. unitsB13 sq. unitsC14 sq. unitsD23 sq. units
Find the area of the region bounded by the parabola y2=8x and the line x=2
View SolutionFind the area of the figure bounded by the parabola y=−x2+2x+3, the line tangent to it at the point M (2,−5) and the y-axis
View SolutionFind the area of the region bounded by the parabola y=x2 and y=|x|.
View SolutionFind the area of the region bounded by the parabola y=x2 and y=|x| .
View SolutionThe area of the region bounded by the parabola x=−y2 and the line y=x+2 is
View SolutionFind the area of the region bounded by the parabola y2=2x and the line x−y=4
View SolutionFind the area of the region bounded by the parabola y2=25x and the line x =5
View Solution