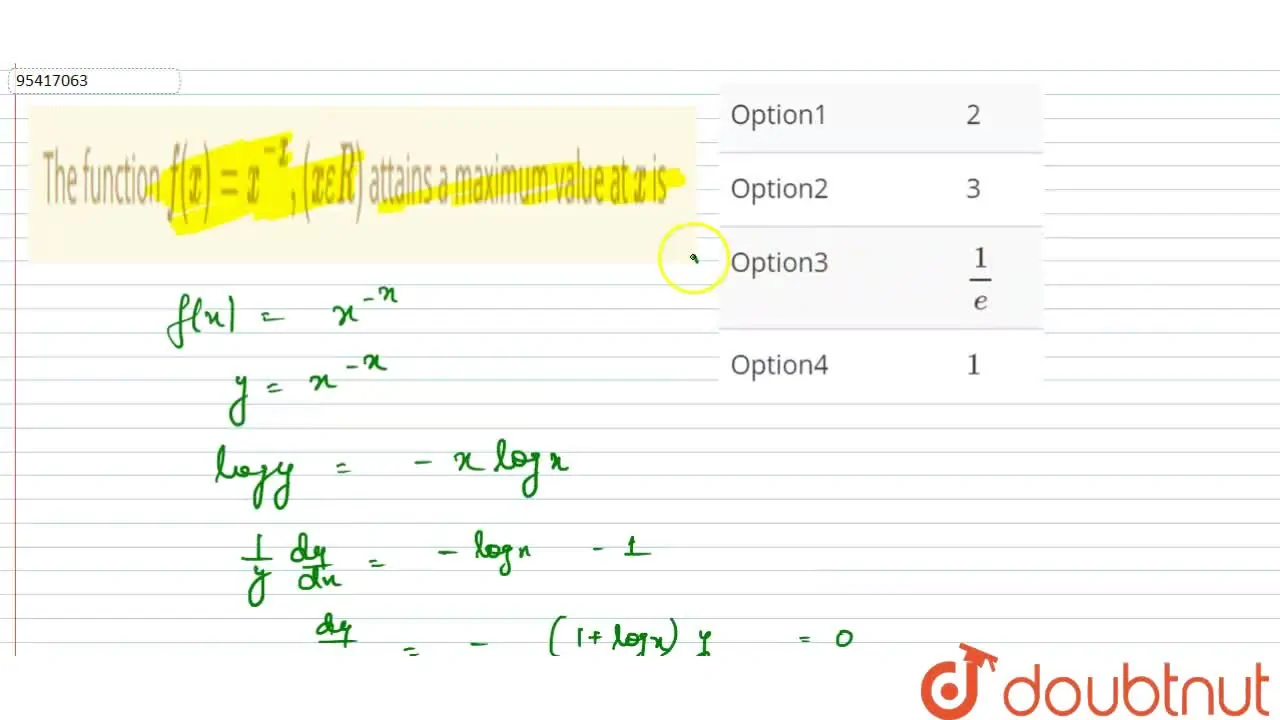
The function f(x)=x−x,(xεR) attains a maximum value at x is
2
3
1e
1
The correct Answer is:C
Given curve is f(x)=y=x−x
On takinglog both sides we get
logy=−xlogx
On differentiating w.r.t x we get
1ydydx=−[x1x+logx] ltbRgt =1ydydx=−[1+logx]
=dydx=−x−x[1+logx]
⇒dydx=x−x[log1x−1]
For maximum put dydx=0
⇒log1x=logee
⇒1x=e⇒x=1e
Related Playlists
Similar Questions
The function f(x)=x−x,(xεR) attains a maximum value at x which is
View SolutionThe function f(x)=xe−x(x∈R) attains a maxium value at x= …..
View SolutionThe function f(x)=x2−4x,x∈[0,4] attains minimum value at
View SolutionThe function f(x)=x2−4x,x∈[0,4] attains minimum value at:
View SolutionThe number of values of x where the function f(x)=cosx+cos(√2x) attains its maximum value is
View SolutionThe function f(x)=x4−62x2+ax+9 attains its maximum value on the interval [0,2] at x = 1. Then the value of a is
View SolutionThe function f(x)=x2,x∈R has no maximum value.
View SolutionStatement -1 The maximum value of f(x)=13x4+8x3−18x2+60is153
Statement -2 : The function g(x) =1f(x) attains its minimum value at x=1 and x=-3View SolutionConsider the function f(x)=x2−x+1x2+x+1
What is the maximum value of the function ?View SolutionThe function f(x)=cosx−sinx has maximum or minimum value at x=…..
View Solution