Voltmeters V1 and V2 are connected in series across a D.C. line V1 reads 80 volts and has a per volt resistance of 200ohms, V2 has a total resistance of 32 kilo ohms.
The line voltage is
120 V
160 V
220 V
240 V
The correct Answer is:D
R1=80×200=16000Ω=16kΩ
current through V1andV2 is same
l2=8016×103=5×10−3A
So, potential difference across V2 is V2=5×10−3×32×103=160V
Hence, line voltage=V1+V2
=80+160=240V
Topper's Solved these Questions
Similar Questions
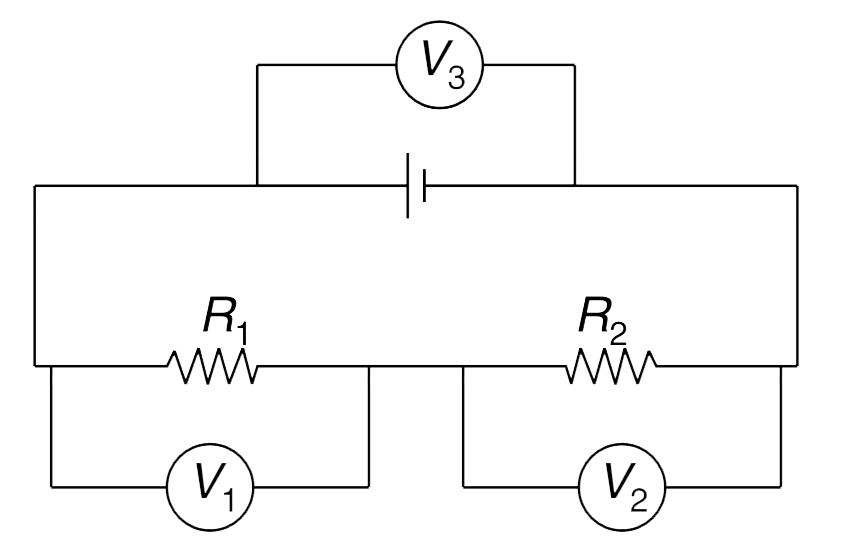
In the circuit shown in the figure, two resistors R1andR2 have been connected in series to an ideal cell. When a voltmeter is connected across R1 its reading is V1=4.0 volt and when the same voltmeter is connected across R2 its reading is V2=6.0 volt. The reading of the voltmeter when it is connected across the cell is V3=12.0 volt. Find the actual across R1 in the circuit.
View SolutionIn the circuit shown below, each battery is 5V and has an internal resistance of 0.2 ohm.
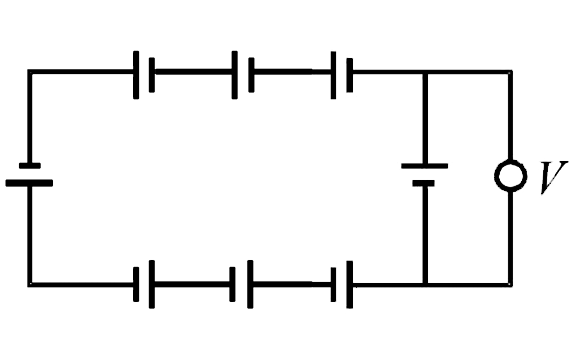
The reading in the ideal voltmeter V is .
View Solution
Knowledge Check
Voltmeters V1andV2 are placed in series and then connected to an ideal battery. V1 reads 80 V and has resistance 20KΩ and V2 has resistance 10KΩ . The EMF of the battery is:
A240VB220VC160VD120VTwo electric bulbs rated P1 watt and V volt, are connected in series, across V-volt supply. The total power consumed is
AP1+P22B√P1.P2CP1.P2P1+P2D(P1+P2)Two electric bulbs rated P1 watt and V volt, are connected in series, across V-volt supply. The total power consumed is
AP1+P22B√P1.P2CP1.P2P1+P2D(P1+P2)
A 600Ω resistor and a 400Ω resistor are connected in series across a 90-volt line. A voltmeter acros the 600Ω resistor reads 45V. What is the value of the voltmeter resistance? If the same voltmeter is connected across the 400Ω resistor, what will be its reading?
View SolutionAn alternating voltage is connected in series with a resistance R and inductance L if the potential drop across the resistance is 200V and across the inductance is 150V, then the applied voltage is
View SolutionA voltmeter of resistance G ohm has range V volt. To increase its range upto (nV), one must connect
View SolutionA voltmeter and an ammeter are connected in series to an ideal cell of emfE. The voltmeter reading is V, and the ammeter readings is I. Then
(i) V<E (ii) the voltmeter resistance is V/I
(iii) the potential difference across the ammeter is E−V
(iv) Voltmeter resistance + ammeter resistance = E//I
Correct statements are
View SolutionAn ideal voltmeter of 1 V has the resistance equal to
View Solution
