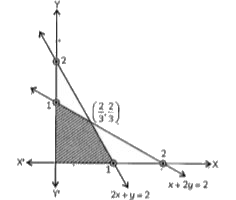
The maximum value of objective function z=2x+3y in the given feasible region is
A
29
B
18
C
14
D
15
Video Solution
Text Solution
The correct Answer is:B

∵z=2x+3y
At O(0,0),z=0
At A(7,0),z=2×7+0=14
At B(6,2),z=2×6+3×2
=12+6=18
and at C(0,5),z=0+3×5=15
From above it is clear that the maximum value of objective function is 18 at B(6,2).
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
The minimum value of linear objective function z=2x+2y under linear constraints 3x+2y≥12,x+3y≥11andx,y≥0 is
A10B12C6D5The maximum value of the objective function Z=3x+2y for linear constraints x+y≤7,2x+3y≤16,x≥0,y≥0 is
A16B21C25D28The maximum value of the objective function
Z=3x+4y
subject to the constraints x+y≤4,x≥0,y≤o isA16B18C20D25
In a LPP, the maximum value of the objective function Z = ax +by is always finite.
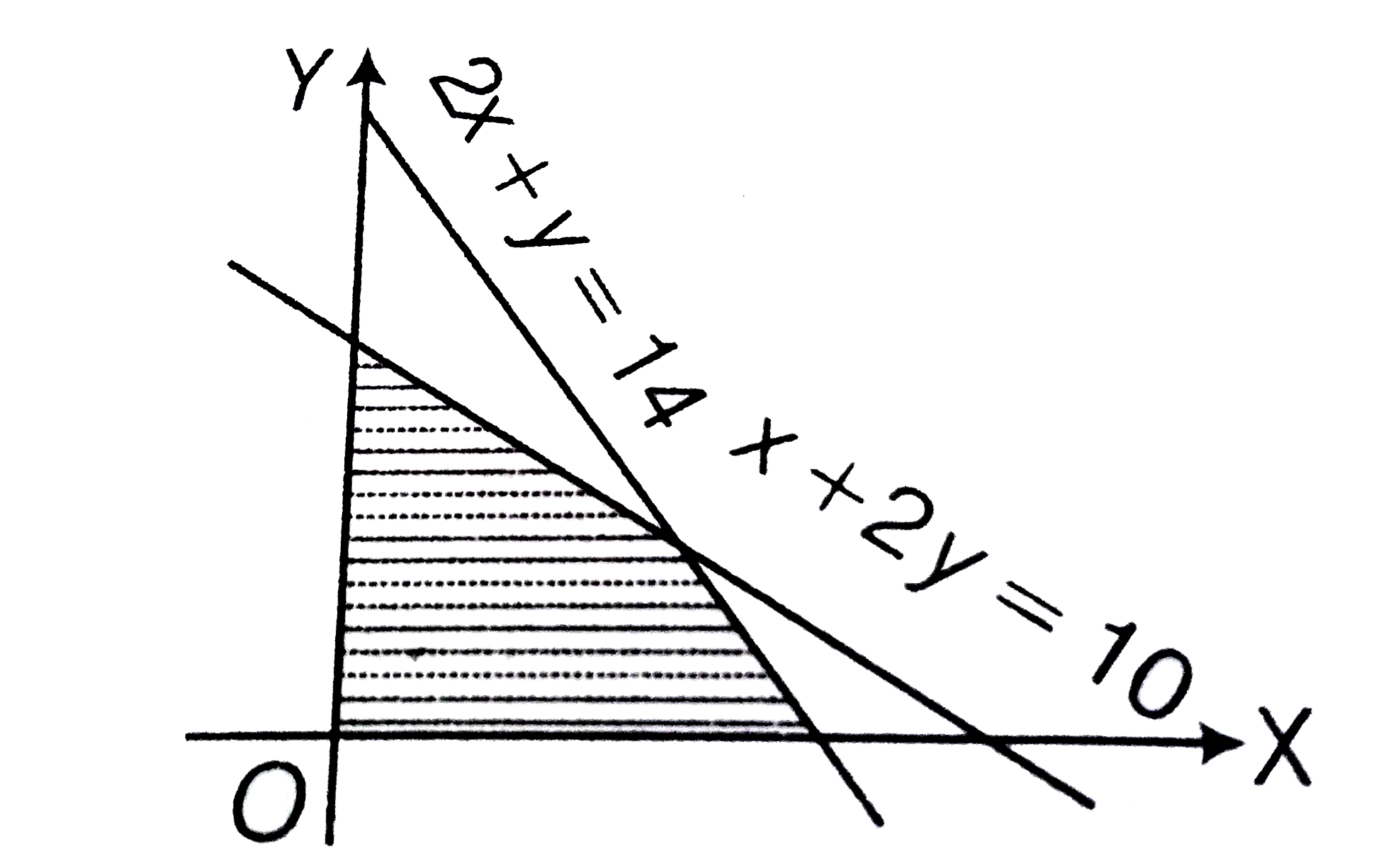
View SolutionThe maximum value of Z = 4x + 3y, if the feasible region for an LPP is shown in following figure, is
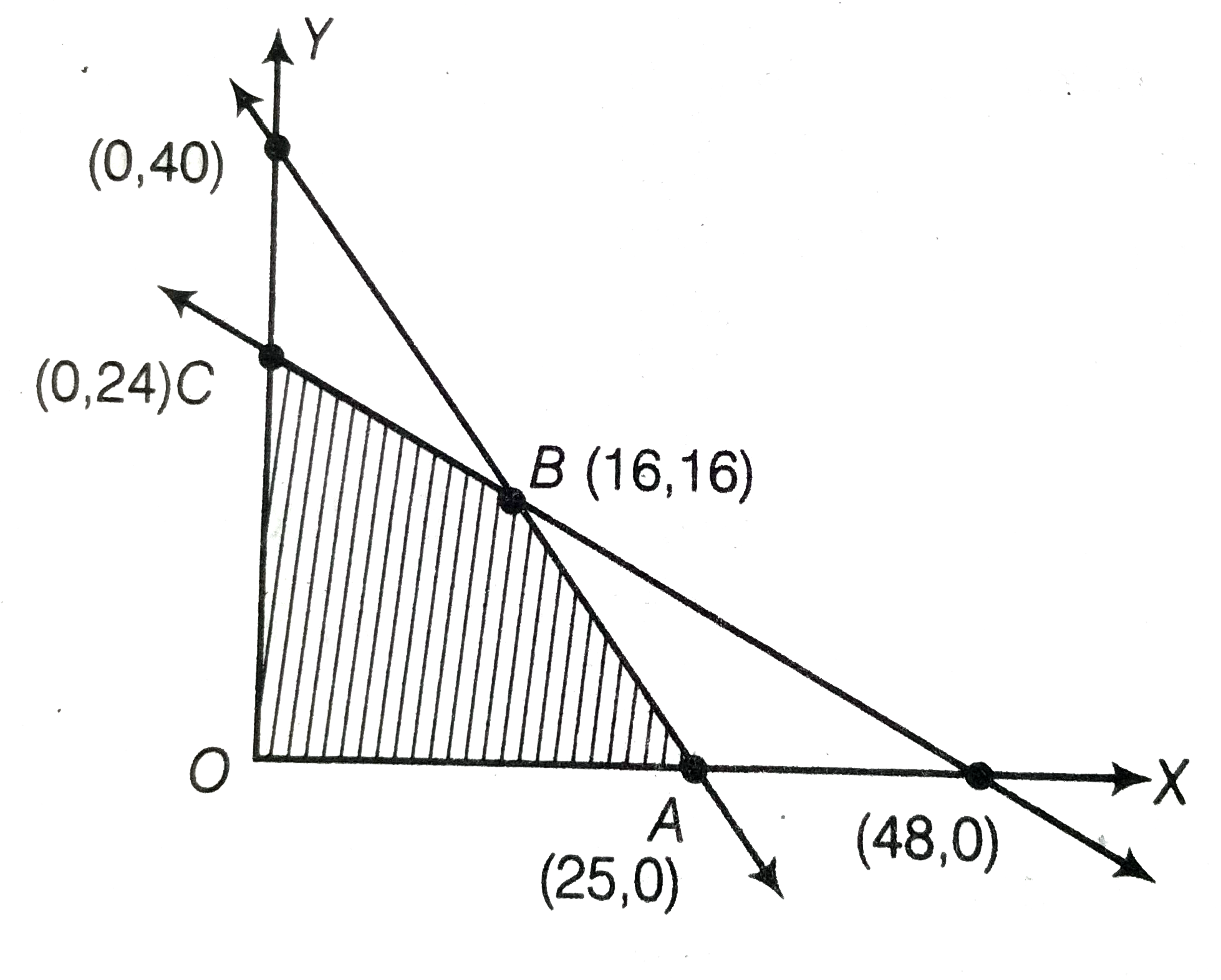
View SolutionThe corner points of the feasible region of a system of linear inequalities are (0, 0), (4,0), (3,9), (1, 5) and (0, 3). If the maximum value of objective function, Z = ax + by occurs at points (3, 9) and (1, 5), then the relation between a and b is:
View SolutionThe minimum value of linear objective function z =2x + 2y under linear constraints 3x+2y≥12,x+3y≥11andx,y≥0 is
View SolutionThe minimum value of the objective function Z=x+2y
Subject to the constraints,
2x+y≥3,x+2y≥6,x,y≥0 occurs
View Solution