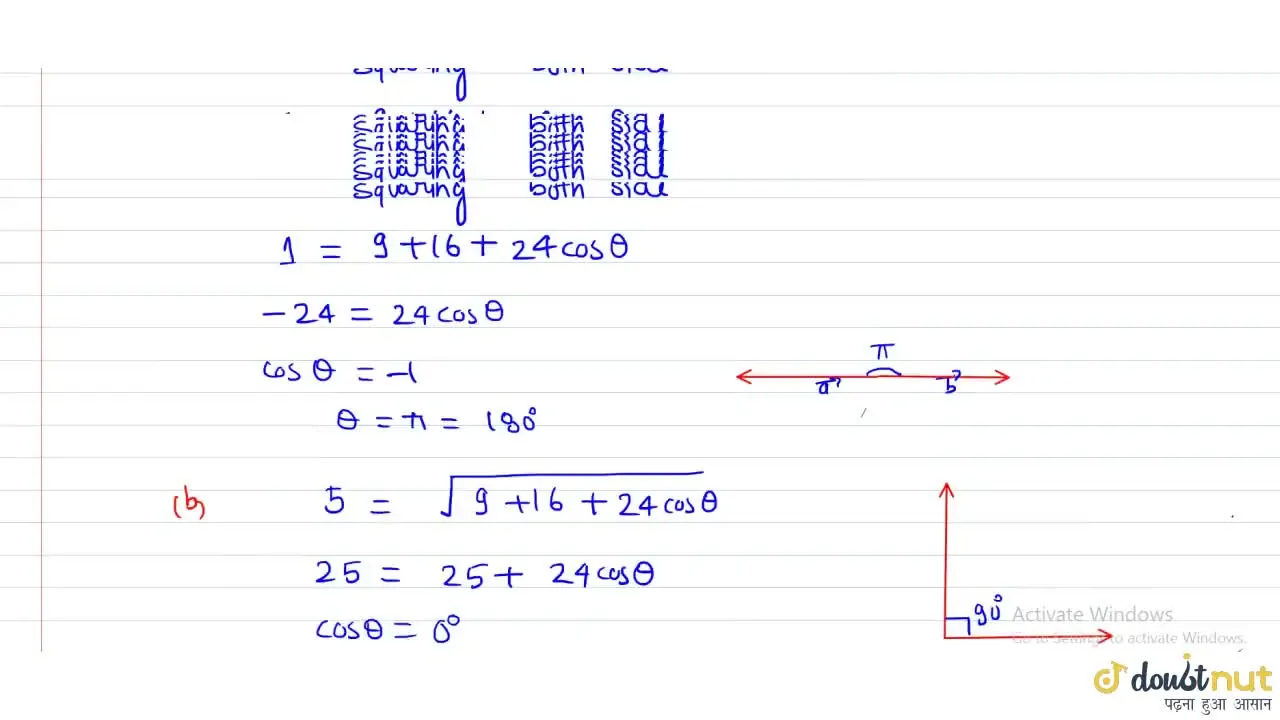
Two vectors have magnitudes 3 unit and 4 unit respectively. What should be the angel between them if the magnitude of the resultant ils a. 1 unit, b. 5 unit and c. 7 unit.
The correct Answer is:A, B, C
−→|a|=3
& ∣∣→b∣∣=4
(a). If R= 1 unit
then √32+42+2.3.4cos(θ)=1
→25+24cos(θ)=1
→24cosθ=−24
→cosθ=−1
→θ=1800
(b).√32+42+2.3.4cosθ=7
→25+24cosθ=49
→24cosθ=24
→cosθ=1
→θ=cos−1(1)=0∘
Hence angle between them is 0∘.
Related Playlists
- BOOK - HC VERMACHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Objective 25videos
PHYSICS AND MATHEMATICS
- BOOK - HC VERMACHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - Exercises42videos
NEWTON'S LAWS OF MOTION
- BOOK - HC VERMACHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Exercises51videos
REST AND MOTION : KINEMATICS
Similar Questions
Two vectors have magnitudes 3 unit and 4 units respectively. What should be the angle between them if the magnitude of the resultant is (a) 1 unit, (b) 6 unit and (c) 7 unit.
View SolutionTwo vectors have magnitudes 3 unit and 4 unit respectively. What should be the angle between them if the magnitude of the resultant is -
(i) 1 unit (ii) 5 unit (iii) 7 unitView SolutionVectors →Cand→D have magnitudes of 3 units and 4 units, respectively. What is the angle between the directions of →Cand→D if →C.→D equals (a) zero, (b) 12 units , and (c ) -12 units ?
View SolutionVectors →Cand→D have magnitudes of 3 units and 4 units, respectively. What is the angle between the directions of →Cand→D if →C.→D equals (a) zero, (b) 12 units , and (c ) -12 units ?
View SolutionThe magnitude in i in ampere unit is
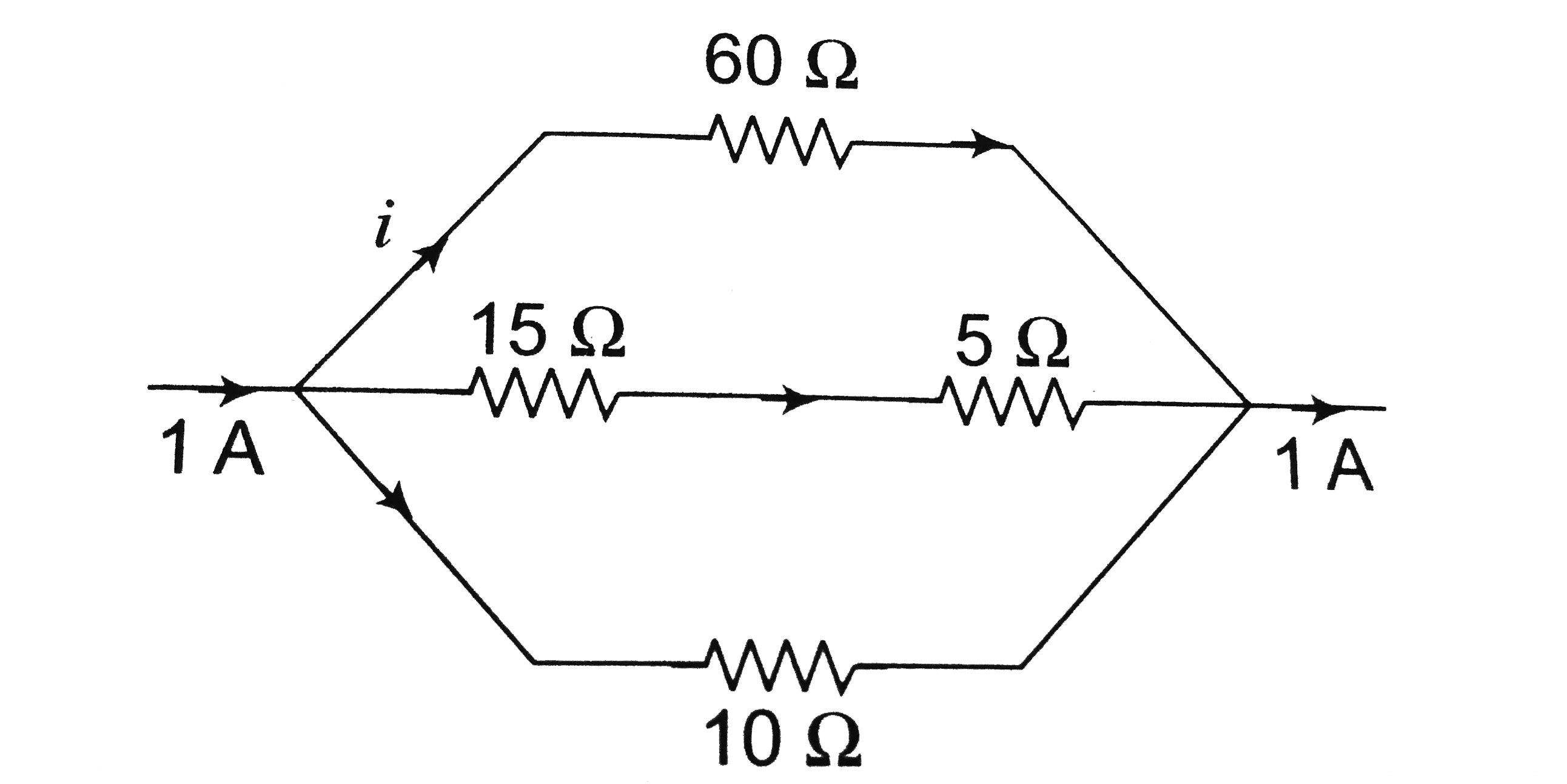 View Solution
View SolutionTwo vectors of magnitudes 10 and 15 units can never have a resultant equal to
View SolutionTwo vectors of magnitudes 10 and 15 units can never have a resultant equal to
View SolutionThe maximum and minimum magnitudes of the resultant of two vectors are 23 units and 7 units respectively. If these two vectors are acting at right angles to each other, the magnitude of their resultant will be
View SolutionThe resultant of two vectors of magnitudes 3 units and 5 units is perpendicular to 3 units.The angle between the vectors is
View SolutionThe maximum and minimum magnitude of the resultant of two given vectors are 17 units and 7 units respectively. If these two vectors are at right angles to each other, the magnitude of their resultant is
View Solution