प्रेक्षणों x1,x2,x3,.......,xn का गुणोत्तर माध्य G1 है।
प्रेक्षणों y1,y2,y3,.......,yn का गुणोत्तर माध्य G2 है।
प्रेक्षणों x1y1,x2y2,x3y3,......xnyn का गुणोत्तर माध्य क्या है?
G1G2
In(G1G2
G1/G2
in(G1/(G2)
The correct Answer is:C
x1,x2,x3,.........,xn का गुणोत्तर माध्य G1 है।
⇒G1=(x1.x2......xn)1n
y1,y2,y3,..........yn का गुणोत्तर माध्य G2 है।
⇒G2=(y1.y2.y3..........yn)1n
∴x1y1.x2y2.x3y3.........xnyn का गुणोत्तर माध्य
=(x1y1.x2y2.x2y3.......xnyn)1/n=(x1x2x3.......xn)1/n(y1y2y3.......yn)1/n=G1G2
Topper's Solved these Questions
Similar Questions
नीचे दिया गया चित्र प्रदर्शित करता है एक
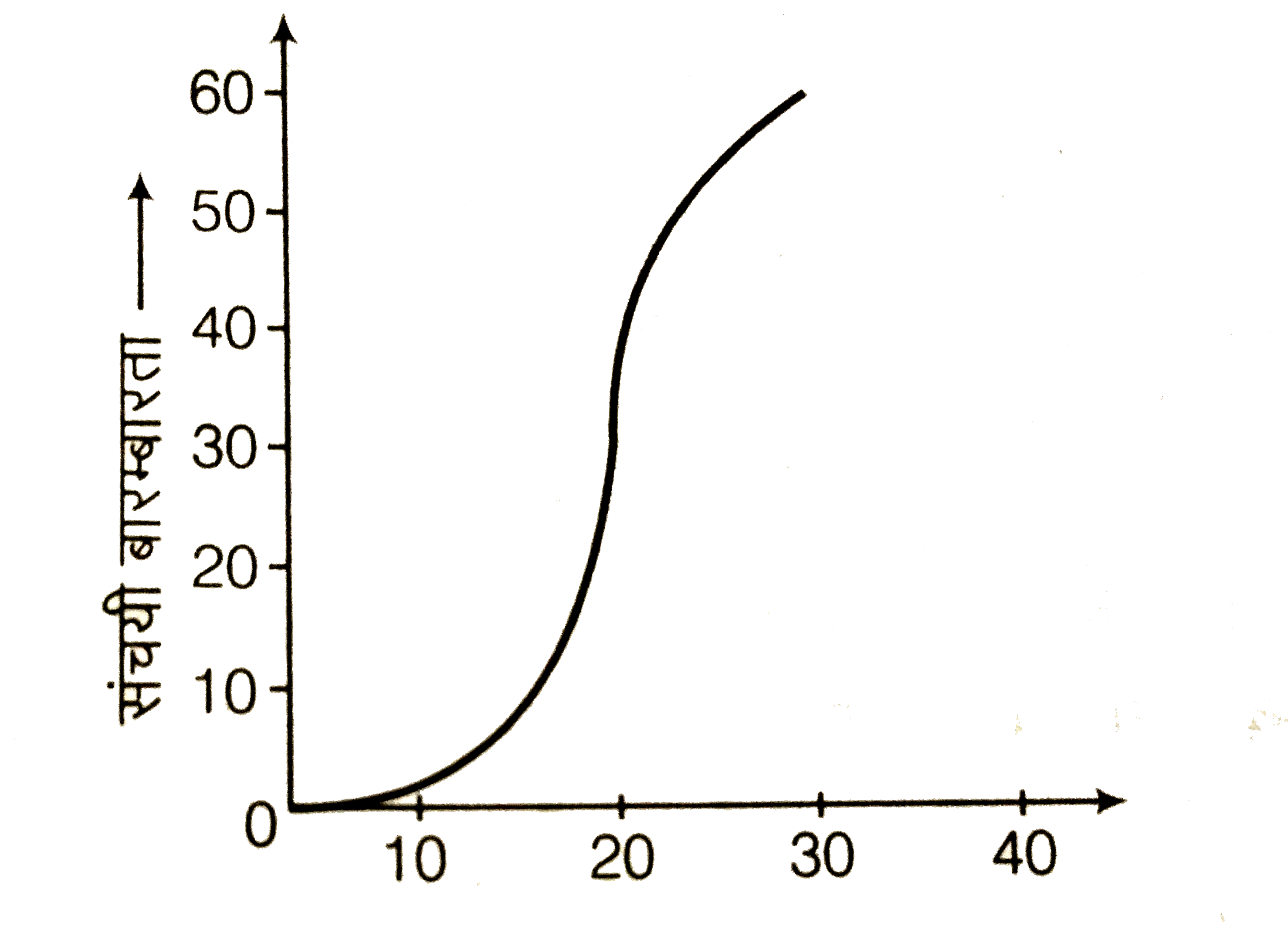
View Solution(i5+i6+i7+i8+i9)(1+i) का मान है
View Solution∣∣ ∣ ∣∣−a2abacab−b2bcacbc−c2∣∣ ∣ ∣∣ किसके बराबर है ?
View SolutionThe value of |2x1y1x1y2+x2y1x1y3+x3y1x1y2+x2y12x2y2x2y3+x3y2x1y3+x3y1x2y3+x3y22x3y3| is.
View SolutionIf the points (x1,y1),(x2,y2), and (x3,y3) are collinear show that y2−y3x2x3+y3−y1x3x1+y1−y2x1x2=0
View SolutionIf the join of (x1,y1) and (x2,y2) makes on obtuse angle at (x3,y3), then prove than (x3−x1)(x3−x2)+(y3−y1)(y3−y2)<0
View SolutionThe geometric mean of the observation x1,x2,x3,……..,n is G1, The geometric mean of the observation y1,y2,y3,…..yn is G2. The geometric mean of observations
x1y1,x2y2,x3y3,……xnyn us
View SolutionLet ¯x be the mean of x1,x2,x3,……..,xn. If xi=a+cyi for some constants a and c, then what will be the mean of y1,y2,y3,……..,yn?
View SolutionA triangle has vertices Ai(xi,yi) for i= 1,2,3,. If the orthocenter of triangle is (0,0) then prove that
∣∣ ∣ ∣∣x2−x3y2−y3y1(y2−y3)+x1(x2−x3)x3−x1y3−y1y2(y3−y1)+x2(x3−x1)x1−x2y1−y2y3(y1−y2)+x3(x1−x2)∣∣ ∣ ∣∣=0
View Solutionअगर बिन्दुएं (x1,y2),(x2,y2) और (x3,y3) संरेखी है तब दिखायें कि y2−y3x2x3+y3,y2x3y1+y1−y2x1x2=0
View SolutionIf A1,A2,A3,…,An are n points in a plane whose coordinates are (x1,y1),(x2,y2),(x3,y3),…,(xn,yn) respectively. A1A2 is bisected in the point G1:G1A3 is divided at G2 in the ratio 1:2,G3A5 at G4 in the1 : 4 and so on untill all the points are exhausted. Show that the coordinates of the final point so obtained are x1+x2+.....+xnn and y1+y2+.....+ynn
View SolutionIf (x1,y1),(x2,y2),(x3,y3)and(x4,y4) be the consecutive vertices of a parallelogram , show that , x1+x3=x2+x4andy1+y3=y2+y4.
View SolutionIf x1,x2,x3 are in A.P. and y1,y2,y3 are in A.P. then the points (x1,y1),(x2,y2),(x3,y3)
View SolutionIf the standard deviation of n observation x1,x2,…….,xn is 5 and for another set of n observation y1,y2,……….,yn is 4, then the standard deviation of n observation
x1−y1,x2−y2,………….,xn−yn is
View SolutionA triangle has vertices Ai(xi,yi) for i= 1,2,3,. If the orthocenter of triangle is (0,0) then prove that
∣∣ ∣ ∣∣x2−x3y2−y3y1(y2−y3)+x1(x2−x3)x3−x1y3−y1y2(y3−y1)+x2(x3−x1)x1−x2y1−y2y3(y1−y2)+x3(x1−x2)∣∣ ∣ ∣∣=0
View Solution
